i-i-i-

i-i-i-

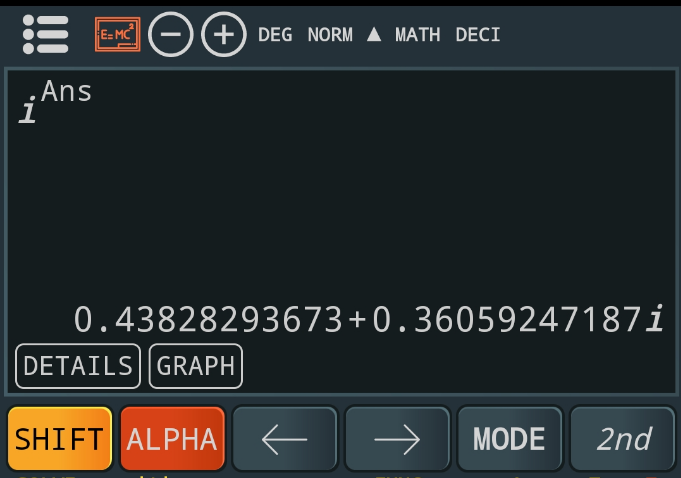
Question Number 101303 by Dwaipayan Shikari last updated on 01/Jul/20

i^{i^{i . \infty}} = ?

Commented by Dwaipayan Shikari last updated on 01/Jul/20

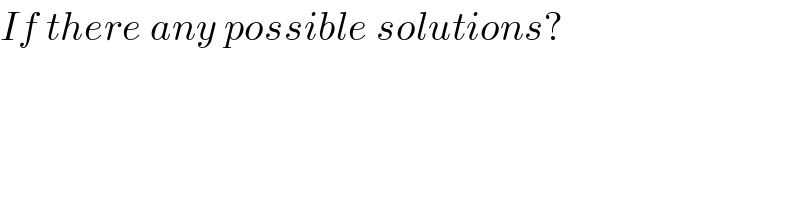
I f t h e r e a n y p o s s i b l e s o l u t i o n s ?

Answered by mr W last updated on 01/Jul/20

s a y i^{i^{i^{\dots}}} = x

i^{x} = x

e^{\frac{π i x}{2}} = x

{x e}^{- \frac{π i x}{2}} = 1

- \frac{π i x}{2} e^{- \frac{π i x}{2}} = - \frac{π i}{2}

- \frac{π i x}{2} = W (- \frac{π i}{2})

\Rightarrow x = - \frac{2}{π i} W (- \frac{π i}{2})

\Rightarrow x = \frac{2 i}{π} W (- \frac{π i}{2})

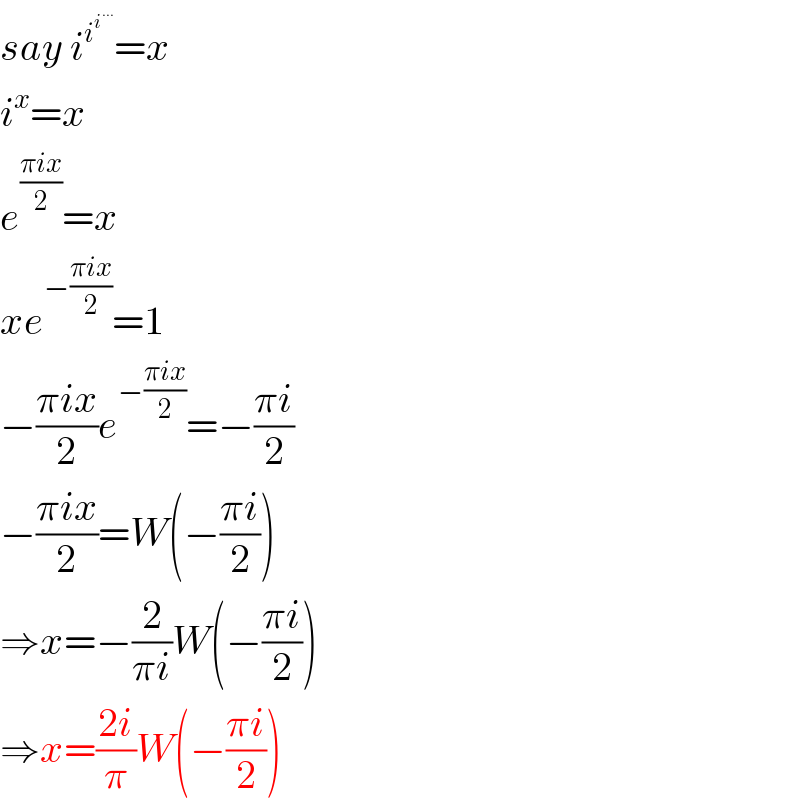
Commented by Dwaipayan Shikari last updated on 01/Jul/20

T h a n k i n g y o u

Commented by mr W last updated on 01/Jul/20

Facebook Tweet Pin