Menu Close

I-n-0-1-n-6n-1-

Tinku Tara 0 Comments
Pin




Question Number 64640 by Tawa1 last updated on 19/Jul/19
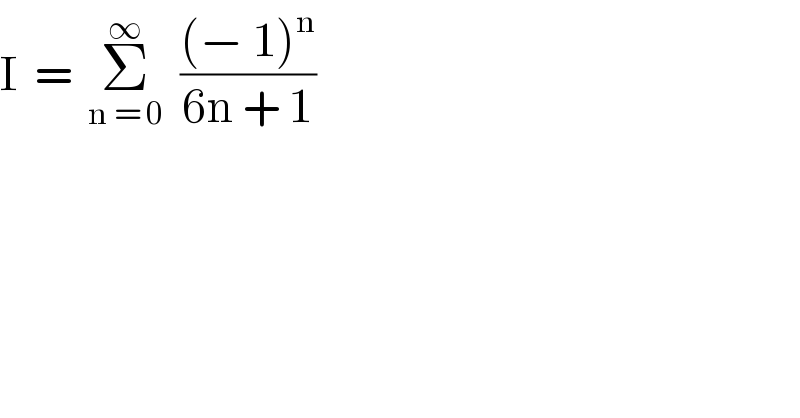
I = Σ_(n = 0) ^∞ (((− 1)^n )/(6n + 1))
$$\mathrm{I}\:\:=\:\:\underset{\mathrm{n}\:=\:\mathrm{0}} {\overset{\infty} {\sum}}\:\:\frac{\left(−\:\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{6n}\:+\:\mathrm{1}} \\ $$
Commented by mathmax by abdo last updated on 20/Jul/19
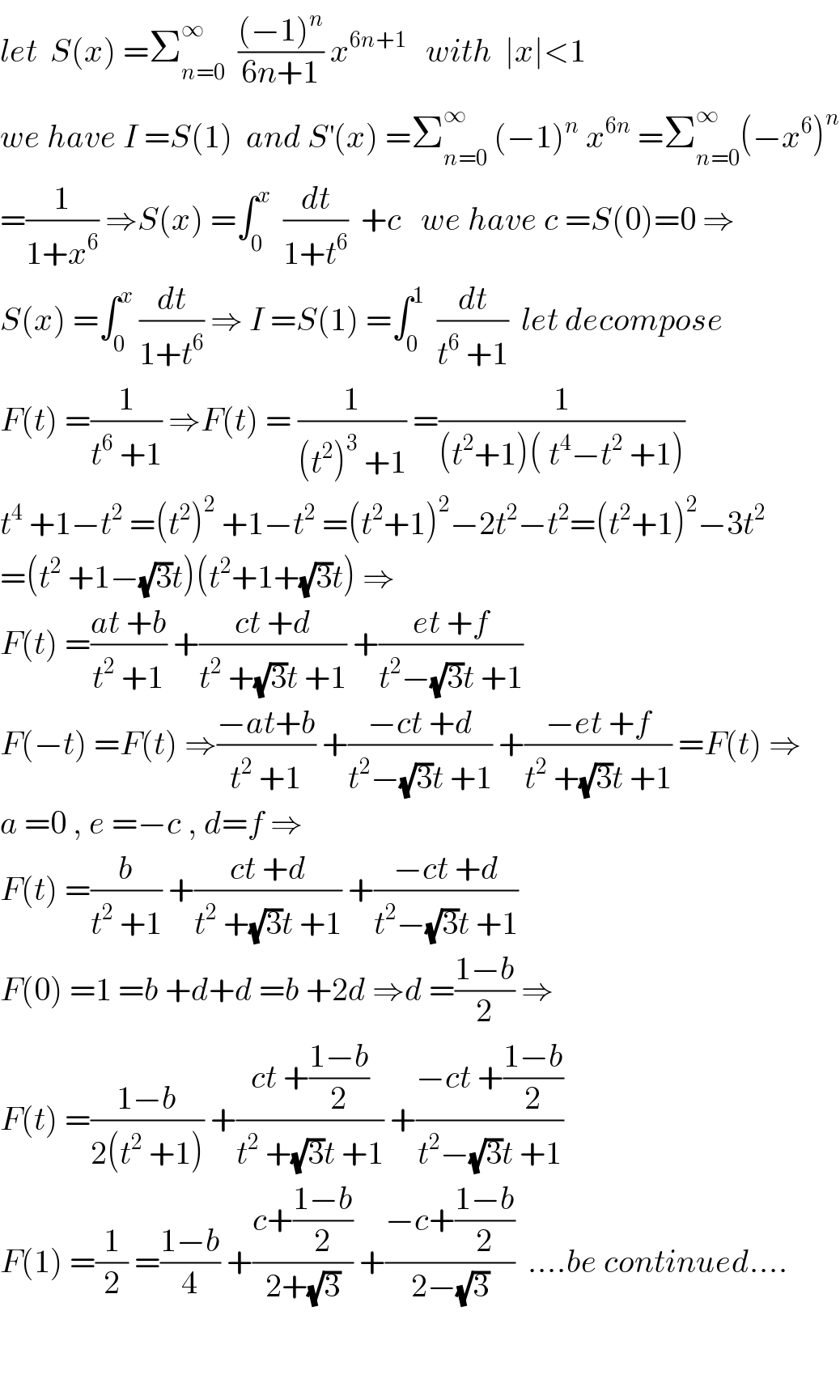
let S(x) =Σ_(n=0) ^∞ (((−1)^n )/(6n+1)) x^(6n+1) with ∣x∣<1 we have I =S(1) and S^′ (x) =Σ_(n=0) ^∞ (−1)^n x^(6n) =Σ_(n=0) ^∞ (−x^6 )^n =(1/(1+x^6 )) ⇒S(x) =∫_0 ^x (dt/(1+t^6 )) +c we have c =S(0)=0 ⇒ S(x) =∫_0 ^x (dt/(1+t^6 )) ⇒ I =S(1) =∫_0 ^1 (dt/(t^6 +1)) let decompose F(t) =(1/(t^6 +1)) ⇒F(t) = (1/((t^2 )^3 +1)) =(1/((t^2 +1)( t^4 −t^2 +1))) t^4 +1−t^2 =(t^2 )^2 +1−t^2 =(t^2 +1)^2 −2t^2 −t^2 =(t^2 +1)^2 −3t^2 =(t^2 +1−(√3)t)(t^2 +1+(√3)t) ⇒ F(t) =((at +b)/(t^2 +1)) +((ct +d)/(t^2 +(√3)t +1)) +((et +f)/(t^2 −(√3)t +1)) F(−t) =F(t) ⇒((−at+b)/(t^2 +1)) +((−ct +d)/(t^2 −(√3)t +1)) +((−et +f)/(t^2 +(√3)t +1)) =F(t) ⇒ a =0 , e =−c , d=f ⇒ F(t) =(b/(t^2 +1)) +((ct +d)/(t^2 +(√3)t +1)) +((−ct +d)/(t^2 −(√3)t +1)) F(0) =1 =b +d+d =b +2d ⇒d =((1−b)/2) ⇒ F(t) =((1−b)/(2(t^2 +1))) +((ct +((1−b)/2))/(t^2 +(√3)t +1)) +((−ct +((1−b)/2))/(t^2 −(√3)t +1)) F(1) =(1/2) =((1−b)/4) +((c+((1−b)/2))/(2+(√3))) +((−c+((1−b)/2))/(2−(√3))) ....be continued....
$${let}\:\:{S}\left({x}\right)\:=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{6}{n}+\mathrm{1}}\:{x}^{\mathrm{6}{n}+\mathrm{1}} \:\:\:{with}\:\:\mid{x}\mid<\mathrm{1} \\ $$$${we}\:{have}\:{I}\:={S}\left(\mathrm{1}\right)\:\:{and}\:{S}^{'} \left({x}\right)\:=\sum_{{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{{n}} \:{x}^{\mathrm{6}{n}} \:=\sum_{{n}=\mathrm{0}} ^{\infty} \left(−{x}^{\mathrm{6}} \right)^{{n}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{6}} }\:\Rightarrow{S}\left({x}\right)\:=\int_{\mathrm{0}} ^{{x}} \:\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{6}} }\:\:+{c}\:\:\:{we}\:{have}\:{c}\:={S}\left(\mathrm{0}\right)=\mathrm{0}\:\Rightarrow \\ $$$${S}\left({x}\right)\:=\int_{\mathrm{0}} ^{{x}} \:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{6}} }\:\Rightarrow\:{I}\:={S}\left(\mathrm{1}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}^{\mathrm{6}} \:+\mathrm{1}}\:\:{let}\:{decompose} \\ $$$${F}\left({t}\right)\:=\frac{\mathrm{1}}{{t}^{\mathrm{6}} \:+\mathrm{1}}\:\Rightarrow{F}\left({t}\right)\:=\:\frac{\mathrm{1}}{\left({t}^{\mathrm{2}} \right)^{\mathrm{3}} \:+\mathrm{1}}\:=\frac{\mathrm{1}}{\left({t}^{\mathrm{2}} +\mathrm{1}\right)\left(\:{t}^{\mathrm{4}} −{t}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$${t}^{\mathrm{4}} \:+\mathrm{1}−{t}^{\mathrm{2}} \:=\left({t}^{\mathrm{2}} \right)^{\mathrm{2}} \:+\mathrm{1}−{t}^{\mathrm{2}} \:=\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}{t}^{\mathrm{2}} −{t}^{\mathrm{2}} =\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −\mathrm{3}{t}^{\mathrm{2}} \\ $$$$=\left({t}^{\mathrm{2}} \:+\mathrm{1}−\sqrt{\mathrm{3}}{t}\right)\left({t}^{\mathrm{2}} +\mathrm{1}+\sqrt{\mathrm{3}}{t}\right)\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{{at}\:+{b}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{{ct}\:+{d}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{3}}{t}\:+\mathrm{1}}\:+\frac{{et}\:+{f}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{3}}{t}\:+\mathrm{1}} \\ $$$${F}\left(−{t}\right)\:={F}\left({t}\right)\:\Rightarrow\frac{−{at}+{b}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{−{ct}\:+{d}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{3}}{t}\:+\mathrm{1}}\:+\frac{−{et}\:+{f}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{3}}{t}\:+\mathrm{1}}\:={F}\left({t}\right)\:\Rightarrow \\ $$$${a}\:=\mathrm{0}\:,\:{e}\:=−{c}\:,\:{d}={f}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{{b}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{{ct}\:+{d}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{3}}{t}\:+\mathrm{1}}\:+\frac{−{ct}\:+{d}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{3}}{t}\:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)\:=\mathrm{1}\:={b}\:+{d}+{d}\:={b}\:+\mathrm{2}{d}\:\Rightarrow{d}\:=\frac{\mathrm{1}−{b}}{\mathrm{2}}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{\mathrm{1}−{b}}{\mathrm{2}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}\:+\frac{{ct}\:+\frac{\mathrm{1}−{b}}{\mathrm{2}}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{3}}{t}\:+\mathrm{1}}\:+\frac{−{ct}\:+\frac{\mathrm{1}−{b}}{\mathrm{2}}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{3}}{t}\:+\mathrm{1}} \\ $$$${F}\left(\mathrm{1}\right)\:=\frac{\mathrm{1}}{\mathrm{2}}\:=\frac{\mathrm{1}−{b}}{\mathrm{4}}\:+\frac{{c}+\frac{\mathrm{1}−{b}}{\mathrm{2}}}{\mathrm{2}+\sqrt{\mathrm{3}}}\:+\frac{−{c}+\frac{\mathrm{1}−{b}}{\mathrm{2}}}{\mathrm{2}−\sqrt{\mathrm{3}}}\:\:….{be}\:{continued}…. \\ $$$$ \\ $$$$ \\ $$
Commented by Tawa1 last updated on 20/Jul/19
God bless you sir. I will be waiting
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{will}\:\mathrm{be}\:\mathrm{waiting} \\ $$
Commented by mathmax by abdo last updated on 20/Jul/19
you are welcome.
$${you}\:{are}\:{welcome}. \\ $$
Answered by mr W last updated on 20/Jul/19
let S(x)=Σ_(n=0) ^∞ (((−1)^n x^(6n+1) )/(6n+1)) I=S(1) S′(x)=Σ_(n=0) ^∞ (−1)^n x^(6n) S′(x)=Σ_(n=0) ^∞ (−x^6 )^n =(1/(1+x^6 )) ⇒S(x)=∫(dx/(1+x^6 ))=(1/(4(√3))) ln ((x^2 +(√3)x+1)/(x^2 −(√3)x+1))+(1/6)[tan^(−1) (2x+(√3))+tan^(−1) (2x−(√3))]+(1/3)tan^(−1) x+C ⇒S(0)=C=0 ⇒S(x)=(1/(4(√3))) ln ((x^2 +(√3)x+1)/(x^2 −(√3)x+1))+(1/6)[tan^(−1) (2x+(√3))+tan^(−1) (2x−(√3))]+(1/3)tan^(−1) x ⇒I=S(1)=(1/(4(√3))) ln ((2+(√3))/(2−(√3)))+(1/6)[tan^(−1) (2+(√3))+tan^(−1) (2−(√3))]+(1/3)tan^(−1) 1 =(1/(2(√3))) ln (2+(√3))+(1/6)×(π/2)+(π/3)×(π/4) ⇒I=(1/6)[π+(√3) ln (2+(√3))]
$${let}\:{S}\left({x}\right)=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}} {x}^{\mathrm{6}{n}+\mathrm{1}} }{\mathrm{6}{n}+\mathrm{1}} \\ $$$${I}={S}\left(\mathrm{1}\right) \\ $$$${S}'\left({x}\right)=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(−\mathrm{1}\right)^{{n}} {x}^{\mathrm{6}{n}} \\ $$$${S}'\left({x}\right)=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(−{x}^{\mathrm{6}} \right)^{{n}} =\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{6}} } \\ $$$$\Rightarrow{S}\left({x}\right)=\int\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{6}} }=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}\:\mathrm{ln}\:\frac{{x}^{\mathrm{2}} +\sqrt{\mathrm{3}}{x}+\mathrm{1}}{{x}^{\mathrm{2}} −\sqrt{\mathrm{3}}{x}+\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{6}}\left[\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}{x}+\sqrt{\mathrm{3}}\right)+\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}{x}−\sqrt{\mathrm{3}}\right)\right]+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{tan}^{−\mathrm{1}} {x}+{C} \\ $$$$\Rightarrow{S}\left(\mathrm{0}\right)={C}=\mathrm{0} \\ $$$$\Rightarrow{S}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}\:\mathrm{ln}\:\frac{{x}^{\mathrm{2}} +\sqrt{\mathrm{3}}{x}+\mathrm{1}}{{x}^{\mathrm{2}} −\sqrt{\mathrm{3}}{x}+\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{6}}\left[\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}{x}+\sqrt{\mathrm{3}}\right)+\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}{x}−\sqrt{\mathrm{3}}\right)\right]+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{tan}^{−\mathrm{1}} {x} \\ $$$$\Rightarrow{I}={S}\left(\mathrm{1}\right)=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}\:\mathrm{ln}\:\frac{\mathrm{2}+\sqrt{\mathrm{3}}}{\mathrm{2}−\sqrt{\mathrm{3}}}+\frac{\mathrm{1}}{\mathrm{6}}\left[\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}+\sqrt{\mathrm{3}}\right)+\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{2}−\sqrt{\mathrm{3}}\right)\right]+\frac{\mathrm{1}}{\mathrm{3}}\mathrm{tan}^{−\mathrm{1}} \mathrm{1} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{3}}}\:\mathrm{ln}\:\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)+\frac{\mathrm{1}}{\mathrm{6}}×\frac{\pi}{\mathrm{2}}+\frac{\pi}{\mathrm{3}}×\frac{\pi}{\mathrm{4}} \\ $$$$\Rightarrow{I}=\frac{\mathrm{1}}{\mathrm{6}}\left[\pi+\sqrt{\mathrm{3}}\:\mathrm{ln}\:\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)\right] \\ $$
Commented by mathmax by abdo last updated on 21/Jul/19
thank you sir mrv.
$${thank}\:{you}\:{sir}\:{mrv}. \\ $$
Commented by mathmax by abdo last updated on 21/Jul/19
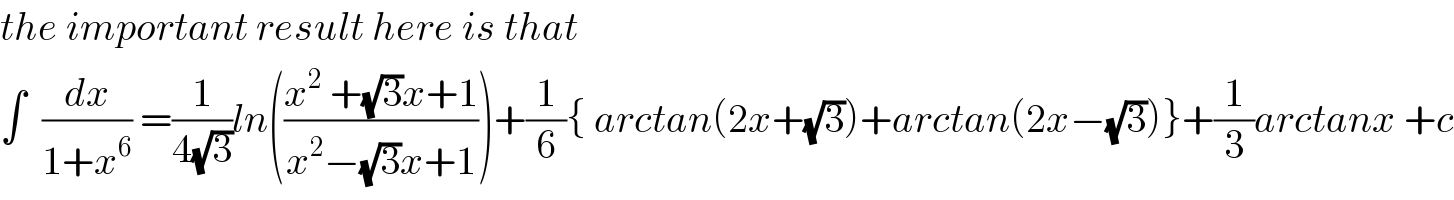
the important result here is that ∫ (dx/(1+x^6 )) =(1/(4(√3)))ln(((x^2 +(√3)x+1)/(x^2 −(√3)x+1)))+(1/6){ arctan(2x+(√3))+arctan(2x−(√3))}+(1/3)arctanx +c
$${the}\:{important}\:{result}\:{here}\:{is}\:{that} \\ $$$$\int\:\:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{6}} }\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{3}}}{ln}\left(\frac{{x}^{\mathrm{2}} \:+\sqrt{\mathrm{3}}{x}+\mathrm{1}}{{x}^{\mathrm{2}} −\sqrt{\mathrm{3}}{x}+\mathrm{1}}\right)+\frac{\mathrm{1}}{\mathrm{6}}\left\{\:{arctan}\left(\mathrm{2}{x}+\sqrt{\mathrm{3}}\right)+{arctan}\left(\mathrm{2}{x}−\sqrt{\mathrm{3}}\right)\right\}+\frac{\mathrm{1}}{\mathrm{3}}{arctanx}\:+{c} \\ $$
Commented by mr W last updated on 21/Jul/19
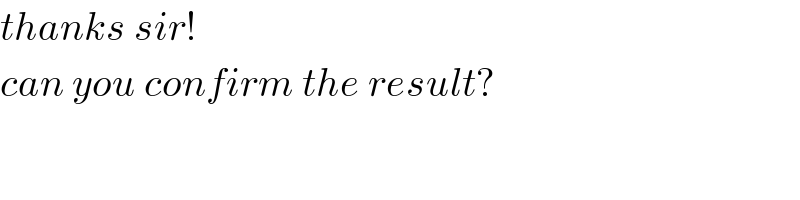
thanks sir! can you confirm the result?
$${thanks}\:{sir}! \\ $$$${can}\:{you}\:{confirm}\:{the}\:{result}? \\ $$
Commented by Tawa1 last updated on 21/Jul/19
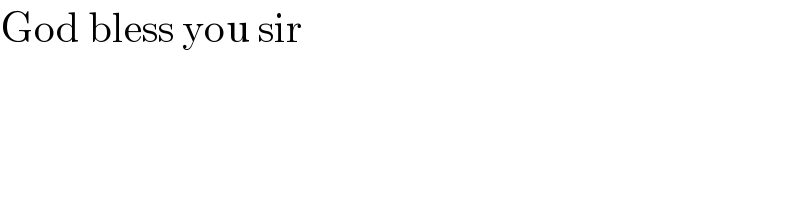
God bless you sir
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by mathmax by abdo last updated on 21/Jul/19
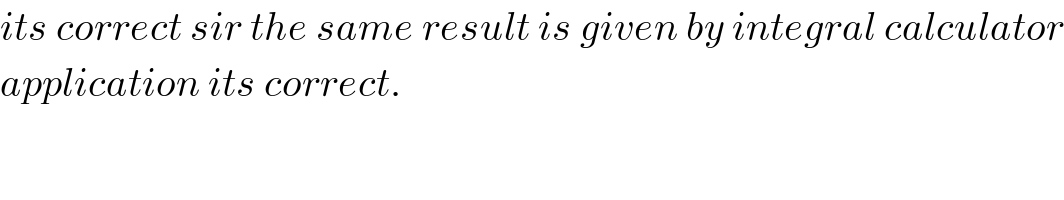
its correct sir the same result is given by integral calculator application its correct.
$${its}\:{correct}\:{sir}\:{the}\:{same}\:{result}\:{is}\:{given}\:{by}\:{integral}\:{calculator} \\ $$$${application}\:{its}\:{correct}. \\ $$
Commented by Cmr 237 last updated on 27/Aug/19
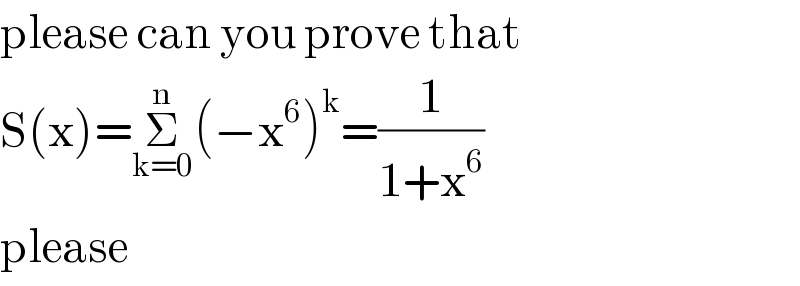
please can you prove that S(x)=Σ_(k=0) ^n (−x^6 )^k =(1/(1+x^6 )) please
$$\mathrm{please}\:\mathrm{can}\:\mathrm{you}\:\mathrm{prove}\:\mathrm{that} \\ $$$$\mathrm{S}\left(\mathrm{x}\right)=\underset{\mathrm{k}=\mathrm{0}} {\overset{\mathrm{n}} {\sum}}\left(−\mathrm{x}^{\mathrm{6}} \right)^{\mathrm{k}} =\frac{\mathrm{1}}{\mathrm{1}+\mathrm{x}^{\mathrm{6}} } \\ $$$$\mathrm{please} \\ $$
Commented by mathmax by abdo last updated on 27/Aug/19
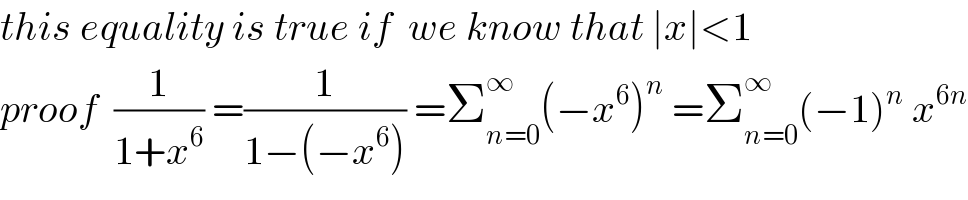
this equality is true if we know that ∣x∣<1 proof (1/(1+x^6 )) =(1/(1−(−x^6 ))) =Σ_(n=0) ^∞ (−x^6 )^n =Σ_(n=0) ^∞ (−1)^n x^(6n)
$${this}\:{equality}\:{is}\:{true}\:{if}\:\:{we}\:{know}\:{that}\:\mid{x}\mid<\mathrm{1} \\ $$$${proof}\:\:\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{6}} }\:=\frac{\mathrm{1}}{\mathrm{1}−\left(−{x}^{\mathrm{6}} \right)}\:=\sum_{{n}=\mathrm{0}} ^{\infty} \left(−{x}^{\mathrm{6}} \right)^{{n}} \:=\sum_{{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} \:{x}^{\mathrm{6}{n}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *