if-3-2-1-2-i-find-z-11-

Question Number 146467 by mathdanisur last updated on 13/Jul/21

i f \frac{\sqrt{3}}{2} + \frac{1}{2} i f i n d z^{11} = ?

Answered by gsk2684 last updated on 13/Jul/21

z^{11} = {(c i s \frac{Π}{6})}^{11} = c i s (\frac{11 Π}{6})

= c i s (2 Π - \frac{Π}{6})

= c i s (- \frac{Π}{6})

= \frac{\sqrt{3}}{2} - \frac{i}{2}

Commented by mathdanisur last updated on 13/Jul/21

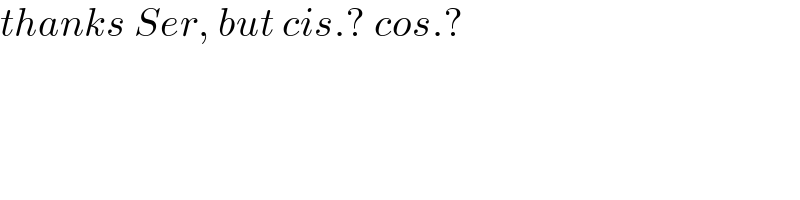
t h a n k s S e r, b u t c i s . ? c o s . ?

Commented by gsk2684 last updated on 13/Jul/21

c i s θ = \cos θ + i \sin θ

Commented by mathdanisur last updated on 13/Jul/21

t h a n k s S e r

Answered by puissant last updated on 13/Jul/21

Z = \cos \frac{π}{6} + i \sin \frac{π}{6} = e^{i \frac{π}{6}}

Z^{11} = {(e^{i \frac{π}{6}})}^{11} = e^{i \frac{11 π}{6}} = \cos \frac{11 π}{6} + i \sin \frac{11 π}{6}

\Rightarrow Z = \frac{\sqrt{3}}{2} - \frac{1}{2} i

Commented by mathdanisur last updated on 13/Jul/21

t h a n k y o u S e r

c o s \frac{11 π}{6} + i s i n \frac{11 π}{6} \Rightarrow z^{11} = \frac{\sqrt{3}}{2} - \frac{1}{2} i . ?

Commented by mathdanisur last updated on 13/Jul/21

t h a n k s S e r