If-4-x-9-1-4-9-x-9-1-8-4-0-then-x-9-1-4-x-9-1-4-

Question Number 118435 by bramlexs22 last updated on 17/Oct/20

I f 4 \sqrt[4]{x^{9}} - 9 \sqrt[8]{x^{9}} + 4 = 0, t h e n

\sqrt[4]{x^{9}} + \sqrt[4]{x^{- 9}} = ?

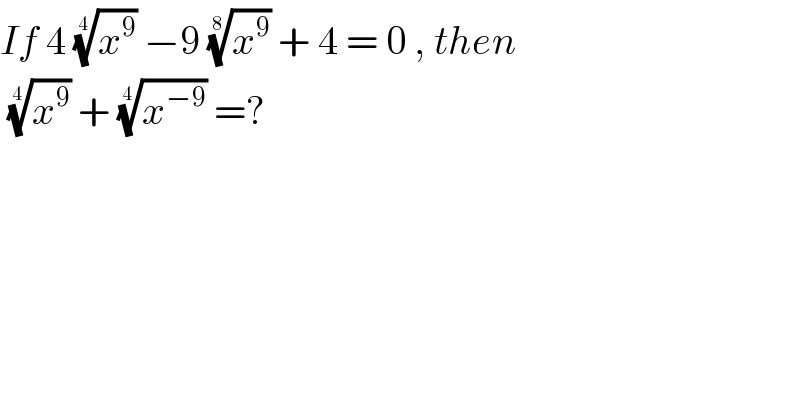
Answered by benjo_mathlover last updated on 18/Oct/20

4 {(x^{\frac{9}{8}})}^{2} - 9 (x^{\frac{9}{8}}) + 4 = 0

l e t t i n g λ = x^{\frac{9}{8}} \Rightarrow 4 λ^{2} - 9 λ + 4 = 0

\Rightarrow λ^{2} - \frac{9}{4} λ + 1 = 0, h a v e t h e r o o t s

a r e λ_{1} = x^{\frac{9}{8}} a n d λ_{2} = x^{- \frac{9}{8}}

\Rightarrow w e w a n t t o c o m p u t e t h e v a l u e o f x^{\frac{9}{4}} + x^{- \frac{9}{4}} i t

e q u a l s t o λ_{1}^{2} + λ_{2}^{2} = {(λ_{1} + λ_{2})}^{2} - 2 λ_{1} . λ_{2}

\Rightarrow λ_{1}^{2} + λ_{2}^{2} = {(\frac{9}{4})}^{2} - 2.1 = \frac{81 - 32}{16} = \frac{49}{16}

t h e r e f o r e : x^{\frac{9}{4}} + x^{- \frac{9}{4}} = \frac{49}{16}

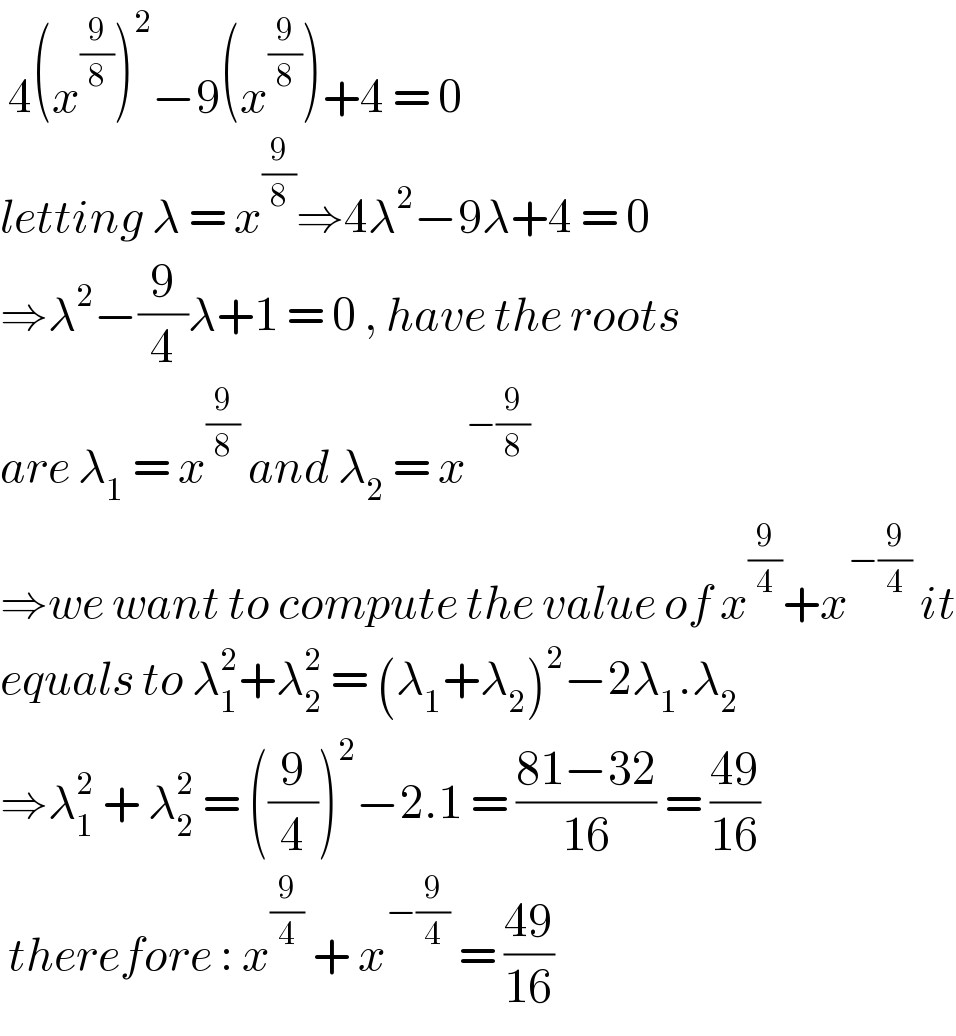
Commented by MJS_new last updated on 17/Oct/20

answer is \frac{49}{16}

Commented by bramlexs22 last updated on 17/Oct/20

h o w s i r ?

Commented by MJS_new last updated on 17/Oct/20

your answer is the same, just expand it!

Answered by MJS_new last updated on 17/Oct/20

we {don}^{'} t have to solve for x

let t = x^{9 / 8}

\Rightarrow the problem turns to

if 4 t^{2} - 9 t + 4 = 0 then t^{2} + t^{- 2} = ?

4 t^{2} - 9 t + 4 = 0

t^{2} - \frac{9}{4} t + 1 = 0

the solutions of t^{2} + p t + 1 are

t_{1} = \frac{- p - \sqrt{p^{2} - 4}}{2} and t_{2} = t_{1}^{- 1}

\Rightarrow t^{2} + t^{- 2} = t_{1}^{2} + t_{2}^{2}

t_{1}^{2} = \frac{p^{2} - 2 + p \sqrt{p^{2} - 4}}{2} \land t_{2}^{2} = \frac{p^{2} - 2 + p \sqrt{p^{2} - 4}}{2}

\Rightarrow t^{2} + t^{- 2} = t_{1}^{2} + t_{2}^{2} = p^{2} - 2

p = - \frac{9}{4} \Rightarrow answer is \frac{49}{16}

Facebook Tweet Pin