Question Number 126400 by F_Nongue last updated on 20/Dec/20
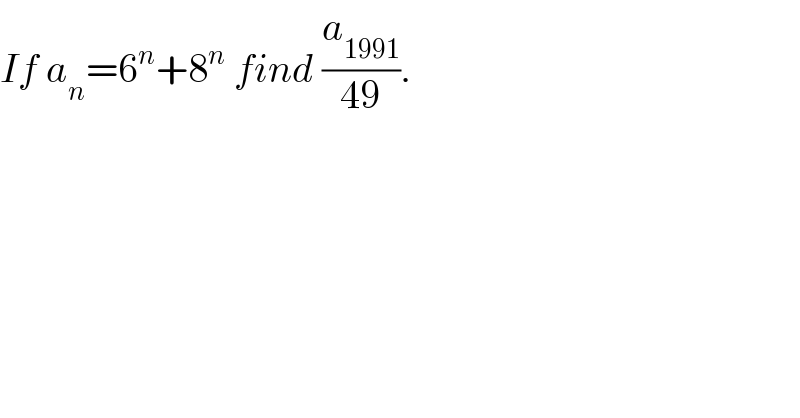
Commented by talminator2856791 last updated on 20/Dec/20
Commented by MJS_new last updated on 20/Dec/20
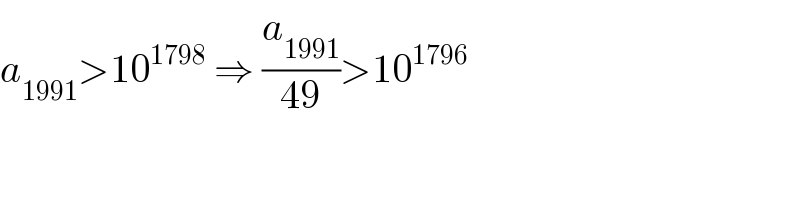
Commented by MJS_new last updated on 20/Dec/20

Commented by F_Nongue last updated on 20/Dec/20

Commented by MJS_new last updated on 20/Dec/20
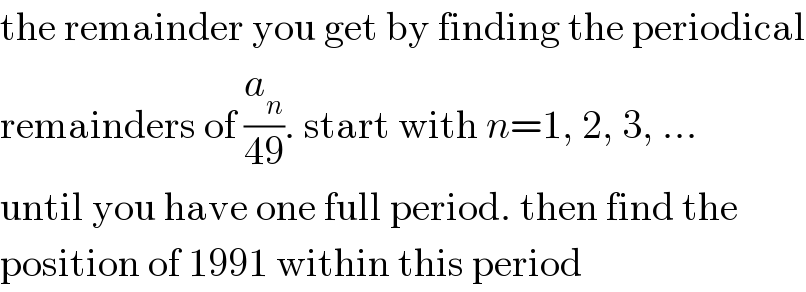
Answered by floor(10²Eta[1]) last updated on 20/Dec/20

