Question Number 30090 by gopikrishnan005@gmail.com last updated on 16/Feb/18

$$\mathrm{if}\:\Delta\mathrm{ABC}\:\mathrm{similar}\:\Delta\mathrm{PQR}\:\mathrm{and}\:\mathrm{area}\:\mathrm{of}\:\Delta\mathrm{PQR}=\mathrm{4area}\left(\Delta\mathrm{ABC}\right)\:\mathrm{then}\:\mathrm{AB}:\mathrm{PQ}\:\mathrm{is} \\ $$
Answered by Rasheed.Sindhi last updated on 17/Feb/18
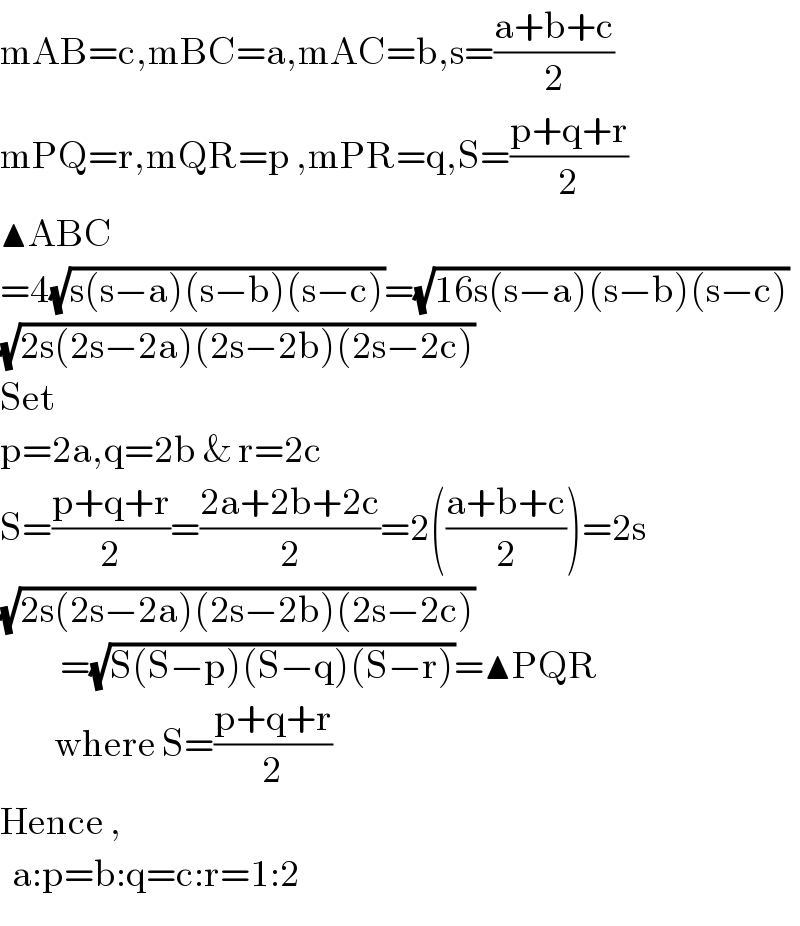
$$\mathrm{mAB}=\mathrm{c},\mathrm{mBC}=\mathrm{a},\mathrm{mAC}=\mathrm{b},\mathrm{s}=\frac{\mathrm{a}+\mathrm{b}+\mathrm{c}}{\mathrm{2}} \\ $$$$\mathrm{mPQ}=\mathrm{r},\mathrm{mQR}=\mathrm{p}\:,\mathrm{mPR}=\mathrm{q},\mathrm{S}=\frac{\mathrm{p}+\mathrm{q}+\mathrm{r}}{\mathrm{2}} \\ $$$$\blacktriangle\mathrm{ABC} \\ $$$$=\mathrm{4}\sqrt{\mathrm{s}\left(\mathrm{s}−\mathrm{a}\right)\left(\mathrm{s}−\mathrm{b}\right)\left(\mathrm{s}−\mathrm{c}\right)}=\sqrt{\mathrm{16s}\left(\mathrm{s}−\mathrm{a}\right)\left(\mathrm{s}−\mathrm{b}\right)\left(\mathrm{s}−\mathrm{c}\right)} \\ $$$$\sqrt{\mathrm{2s}\left(\mathrm{2s}−\mathrm{2a}\right)\left(\mathrm{2s}−\mathrm{2b}\right)\left(\mathrm{2s}−\mathrm{2c}\right)} \\ $$$$\mathrm{Set} \\ $$$$\mathrm{p}=\mathrm{2a},\mathrm{q}=\mathrm{2b}\:\&\:\mathrm{r}=\mathrm{2c} \\ $$$$\mathrm{S}=\frac{\mathrm{p}+\mathrm{q}+\mathrm{r}}{\mathrm{2}}=\frac{\mathrm{2a}+\mathrm{2b}+\mathrm{2c}}{\mathrm{2}}=\mathrm{2}\left(\frac{\mathrm{a}+\mathrm{b}+\mathrm{c}}{\mathrm{2}}\right)=\mathrm{2s} \\ $$$$\sqrt{\mathrm{2s}\left(\mathrm{2s}−\mathrm{2a}\right)\left(\mathrm{2s}−\mathrm{2b}\right)\left(\mathrm{2s}−\mathrm{2c}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:=\sqrt{\mathrm{S}\left(\mathrm{S}−\mathrm{p}\right)\left(\mathrm{S}−\mathrm{q}\right)\left(\mathrm{S}−\mathrm{r}\right)}=\blacktriangle\mathrm{PQR} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{where}\:\mathrm{S}=\frac{\mathrm{p}+\mathrm{q}+\mathrm{r}}{\mathrm{2}} \\ $$$$\mathrm{Hence}\:, \\ $$$$\:\:\mathrm{a}:\mathrm{p}=\mathrm{b}:\mathrm{q}=\mathrm{c}:\mathrm{r}=\mathrm{1}:\mathrm{2}\: \\ $$
