If-for-positive-integers-a-and-b-a-b-a-b-b-a-find-a-2-b-2-

Question Number 16990 by 1234Hello last updated on 29/Jun/17

If for positive integers a and b,

a + b = \frac{a}{b} + \frac{b}{a}, find a^{2} + b^{2} .

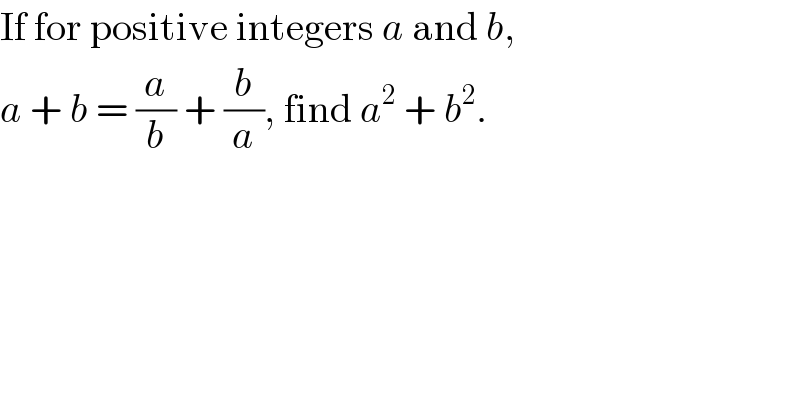
Commented by RasheedSoomro last updated on 29/Jun/17

a + b = \frac{a}{b} + \frac{b}{a}

\frac{a^{2} + b^{2}}{a b} = (a + b)

a^{2} + b^{2} = (a + b) (a b) = a^{2} b + {a b}^{2}

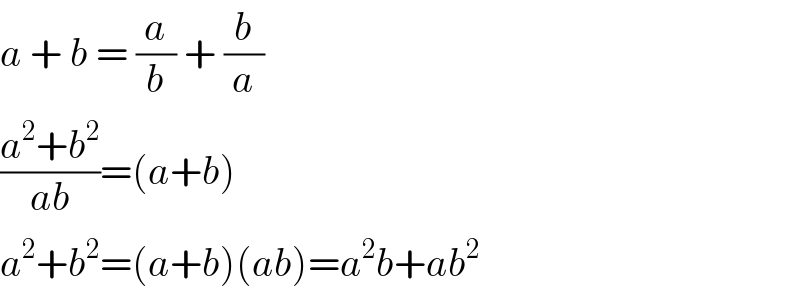
Commented by 1234Hello last updated on 29/Jun/17

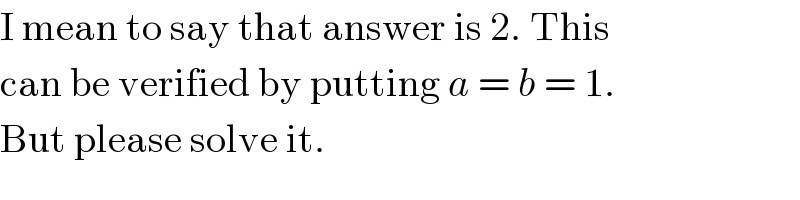
I mean to say that answer is 2 . This

can be verified by putting a = b = 1 .

But please solve it .

Commented by RasheedSoomro last updated on 29/Jun/17

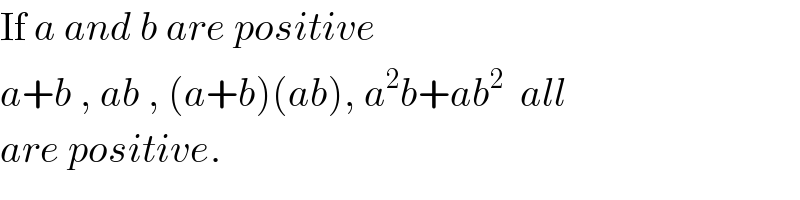
If a a n d b a r e p o s i t i v e

a + b, a b, (a + b) (a b), a^{2} b + {a b}^{2} a l l

a r e p o s i t i v e .

Commented by RasheedSoomro last updated on 29/Jun/17

I now understand the question!

But the solution is difficult .

Answered by mrW1 last updated on 29/Jun/17

let a + b = n and ab = m

n, m = + integer

\frac{a}{b} + \frac{b}{a} = \frac{a^{2} + b^{2}}{ab} = a + b = n

\Rightarrow a^{2} + b^{2} = nab

a^{2} + b^{2} + 2 ab = n^{2}

(n + 2) ab = n^{2}

(n + 2) m = n^{2}

n^{2} - mn - 2 m = 0

D = m^{2} + 8 m = k^{2}

m^{2} + 8 m - k^{2} = 0

D = 8^{2} + {4 k}^{2} = 4 (4^{2} + k^{2}) = i^{2}

4^{2} + k^{2} = {(\frac{i}{2})}^{2}

only solution is k = 3, i = 10

m^{2} + 8 m = 9

\Rightarrow m = 1

\Rightarrow ab = 1

only solution is

a = b = 1

\Rightarrow a^{2} + b^{2} = 2

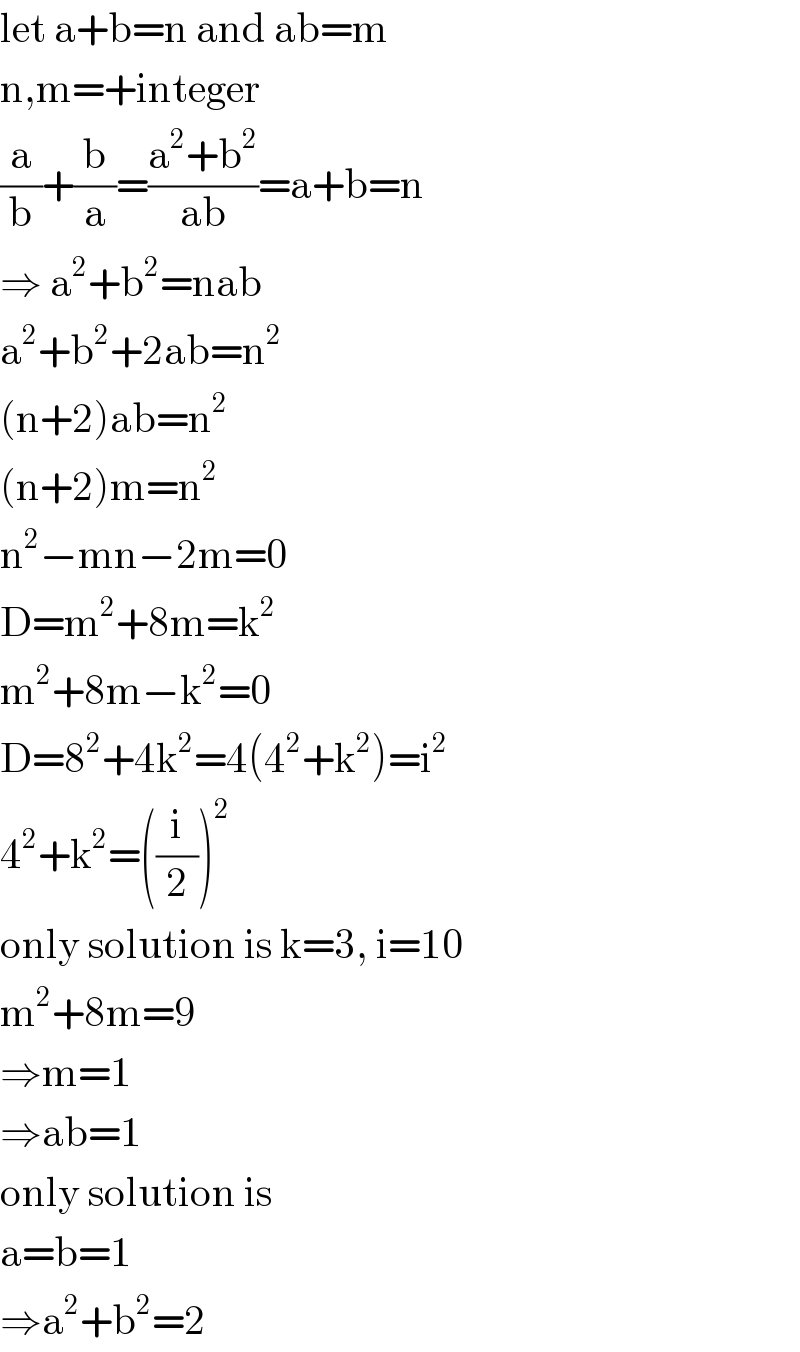
Commented by Tinkutara last updated on 29/Jun/17

Thanks Sir!

Commented by RasheedSoomro last updated on 29/Jun/17

Very nice sir!