If-in-a-ABC-cos-A-cos-B-cos-C-3-2-Prove-that-ABC-is-an-equilateral-triangle-

Question Number 15824 by Tinkutara last updated on 18/Jun/17

If in a Δ A B C, \cos A + \cos B + \cos C = \frac{3}{2} .

Prove that Δ A B C is an equilateral

triangle .

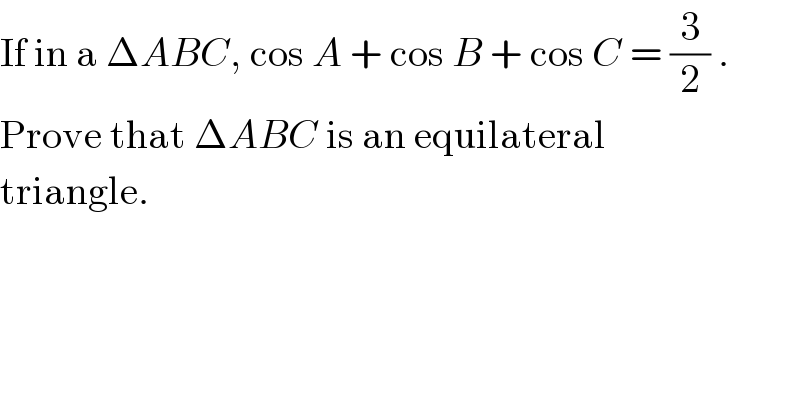
Answered by mrW1 last updated on 14/Jun/17

C = 180 - (A + B)

\cos C = - \cos (A + B)

S = \cos A + \cos B + \cos C = \cos A + \cos B - \cos (A + B)

let x = A, y = B

S = \cos x + \cos y - \cos (x + y)

\frac{\partial S}{\partial x} = - \sin x + \sin (x + y) = 0

\Rightarrow \sin x - \sin (x + y) = 0 \dots (i)

\frac{\partial S}{\partial y} = - \sin y + \sin (x + y) = 0

\Rightarrow \sin y - \sin (x + y) = 0 \dots (ii)

from (i) and (ii)

\sin x = \sin y

\Rightarrow x = y

\sin 2 x + \sin x = 0

\sin x (1 - 2 \cos x) = 0

\Rightarrow \cos x = \frac{1}{2}

x = y = \frac{π}{3}

S_{\max} = \frac{1}{2} + \frac{1}{2} - (- \frac{1}{2}) = \frac{3}{2}

S ⩽ \frac{3}{2}

only for x = y = z = \frac{π}{3}, S = S_{\max} = \frac{3}{2}

\Rightarrow the only solution for \cos A + \cos B + \cos C = \frac{3}{2}

is x = y = z = \frac{π}{3}, i . e . Δ ABC is equilateral .

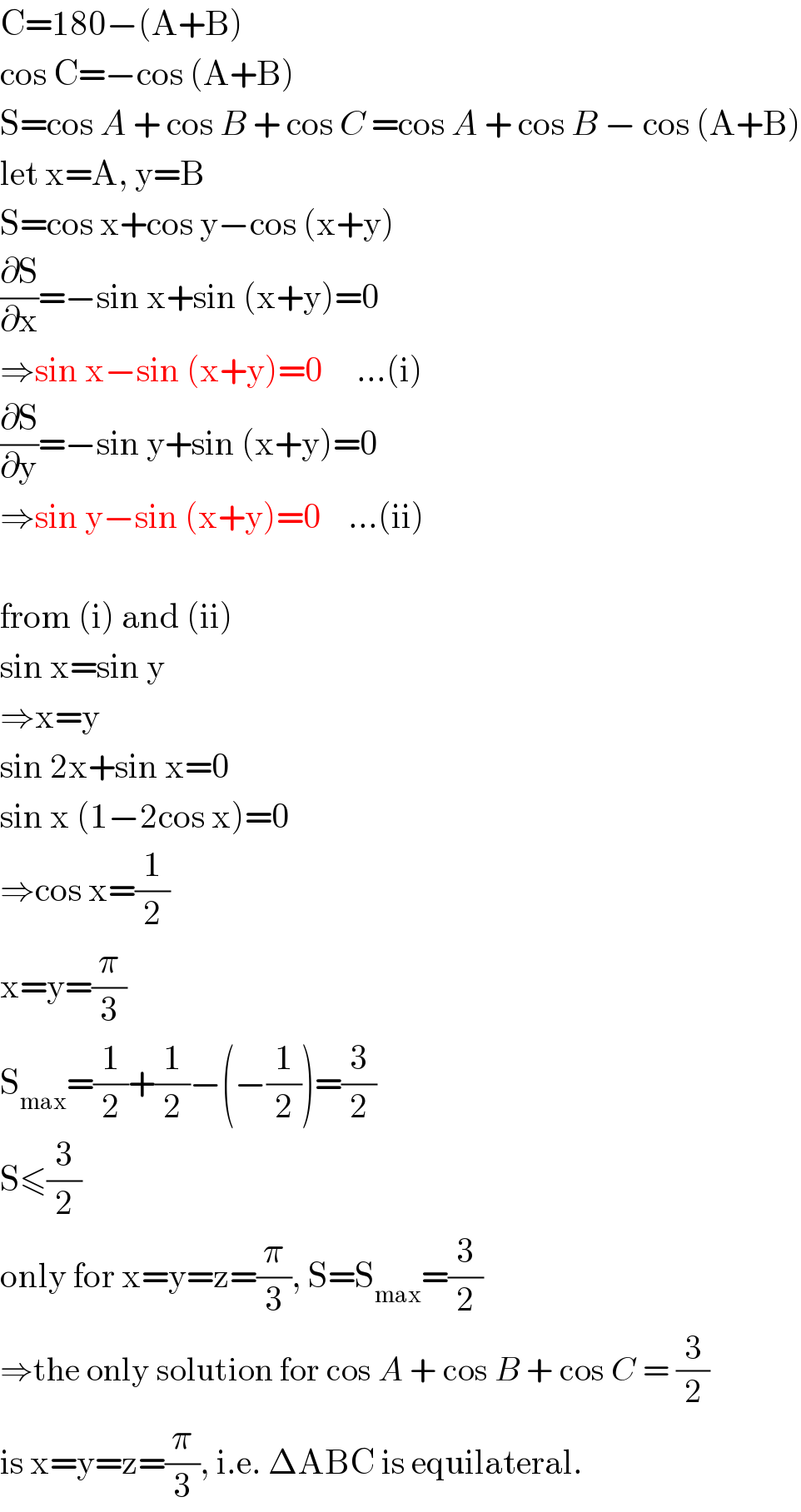
Commented by Tinkutara last updated on 14/Jun/17

Thanks Sir!

Commented by mrW1 last updated on 14/Jun/17

from eqn . (i) and (ii) \Rightarrow x = y

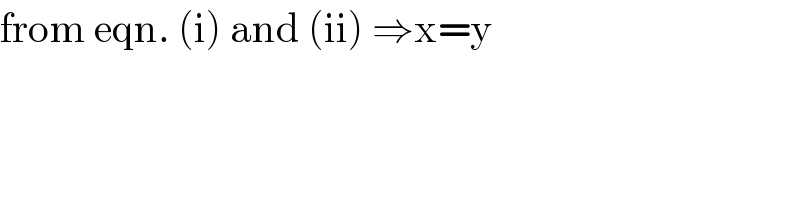
Answered by b.e.h.i.8.3.4.1.7@gmail.com last updated on 14/Jun/17

c o s A + c o s B + c o s C = 2 c o s \frac{A + B}{2} c o s \frac{A - B}{2} + c o s (180 - (A + B)) =

= 2 c o s \frac{A + B}{2} c o s \frac{A - B}{2} - c o s (A + B) =

2 c o s \frac{A + B}{2} c o s \frac{A - B}{2} - 2 {c o s}^{2} \frac{A + B}{2} + 1 =

2 c o s \frac{A + B}{2} (c o s \frac{A - B}{2} - c o s \frac{A + B}{2}) + 1 =

2 c o s (90 - \frac{C}{2}) (s i n \frac{A}{2} s i n \frac{B}{2}) + 1 =

= 4 s i n \frac{A}{2} s i n \frac{B}{2} s i n \frac{C}{2} + 1

\Rightarrow 4 s i n \frac{A}{2} s i n \frac{B}{2} s i n \frac{C}{2} + 1 = \frac{3}{2} \Rightarrow

\Rightarrow s i n \frac{A}{2} s i n \frac{B}{2} s i n \frac{C}{2} = \frac{1}{8}

s i n \frac{A}{2} = \sqrt{\frac{1 - c o s A}{2}} = \sqrt{\frac{1 - \frac{b^{2} + c^{2} - a^{2}}{2 b c}}{2}} =

= \sqrt{\frac{a^{2} - {(b - c)}^{2}}{4 b c}} = \sqrt{\frac{(a + b - c) (a + c - b)}{4 b c}} =

= \sqrt{\frac{2 (p - c) 2 (p - b)}{4 b c}} = \sqrt{\frac{(p - b) (p - c)}{b c}}

\Rightarrow \sqrt{\frac{(p - b) (p - c)}{b c}} . \sqrt{\frac{(p - a) (p - c)}{a c}} . \sqrt{\frac{(p - a) (p - b)}{a b}} = \frac{1}{8}

\Rightarrow \frac{(p - a) (p - b) (p - c)}{a b c} = \frac{1}{8} \Rightarrow \frac{S^{2}}{p . a b c} = \frac{1}{8}

\Rightarrow \frac{S}{p} . \frac{S}{a b c} = \frac{1}{8} \Rightarrow r . \frac{1}{4 R} = \frac{1}{8} \Rightarrow R = 2 r .

n o t e :

i n a n y t r i a n g l e A B C :

1) S = p . r (r = r a d i u s o f i n c i r c l e o f A B C)

2) a b c = 4 R . S (R = r s d i u s o f o u t c i r c l e o f A B C)

3) d^{2} = R^{2} - 2 r . R ({E u l e r}^{'} s r u l e, d = d i s t a n c e

b e t w e e n c e n t e r s o f (i n) a n d) o u t (c i r c l e s)

o n l y i n e q u i l a t e r a l t r i a n g l e, c e n t e r s o f

(i n) a n d) o u t (c i r c l e s a r e t h e s a m e p o i n t .

i . e : d = 0 \Rightarrow R = 2 r .

s o t h i s t r i a n g l e i s e q u i l a t e r a l .

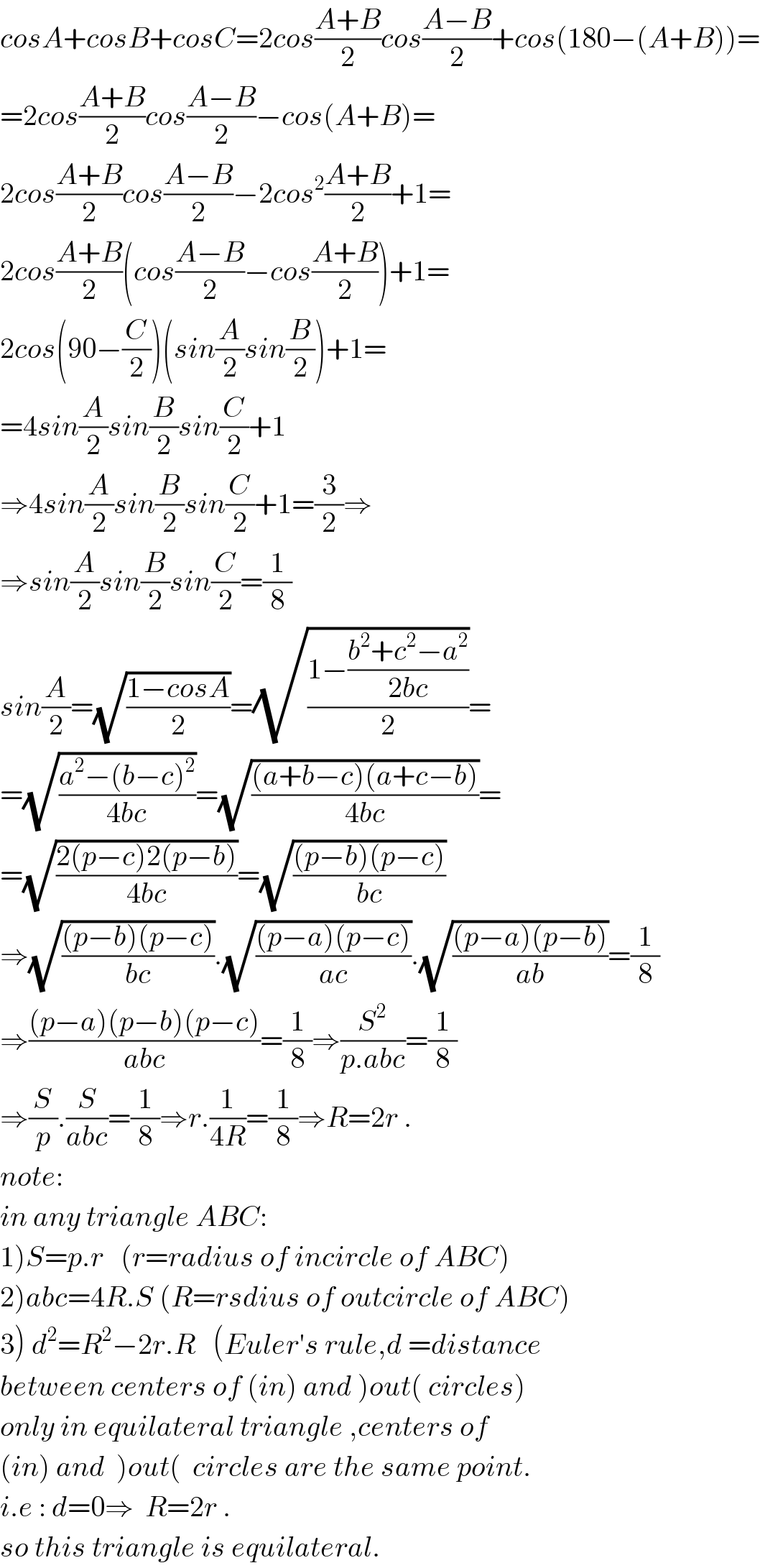
Commented by Tinkutara last updated on 15/Jun/17

Thanks Sir!