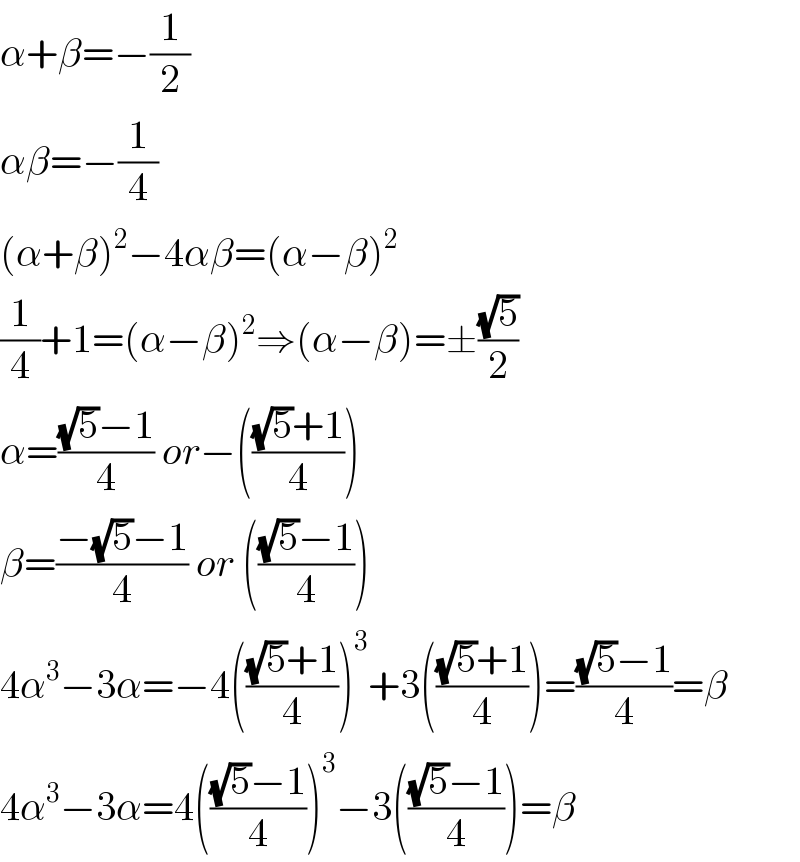Menu Close

If-is-a-root-of-the-equation-4x-2-2x-1-0-How-do-you-prove-the-other-root-is-4-3-3-

Tinku Tara 0 Comments
Pin




Question Number 113211 by bemath last updated on 11/Sep/20
If α is a root of the equation 4x^2 +2x−1=0 . How do you prove the other root is 4α^3 −3α ?
Ifαisarootoftheequation4x2+2x−1=0.Howdoyouprovetheotherrootis4α3−3α?
Answered by 1549442205PVT last updated on 11/Sep/20
α is a root of the equation 4x^2 +2x−1=0 (1) ,so we have 4α^2 +2α−1=0 ⇒4α^3 =α(1−2α)=α−2α^2 .From that 4α^3 −3α=−2α^2 −2α.Replace into(1) we get 4(2α^2 +2α)^2 −2(2α^2 +2α)−1 =16(α^4 +2α^3 +α^2 )−4α^2 −4α−1 =16α^4 +32α^3 +12α^2 −4α−1 =(4α^2 +2α−1)^2 +16α^3 +16α^2 −2 =16α^3 +16α^2 −2=4α(4α^2 +2α−1) +8α^2 +4α−2=8α^2 +4α−2 =2(4α^2 +2α−1)=0 This show that 4α^3 −3α is also other root of the equation 4x^2 +2x−1=0(q.e.d)
αisarootoftheequation4x2+2x−1=0(1),sowehave4α2+2α−1=0⇒4α3=α(1−2α)=α−2α2.Fromthat4α3−3α=−2α2−2α.Replaceinto(1)weget4(2α2+2α)2−2(2α2+2α)−1=16(α4+2α3+α2)−4α2−4α−1=16α4+32α3+12α2−4α−1=(4α2+2α−1)2+16α3+16α2−2=16α3+16α2−2=4α(4α2+2α−1)+8α2+4α−2=8α2+4α−2=2(4α2+2α−1)=0Thisshowthat4α3−3αisalsootherrootoftheequation4x2+2x−1=0(q.e.d)
Answered by $@y@m last updated on 11/Sep/20
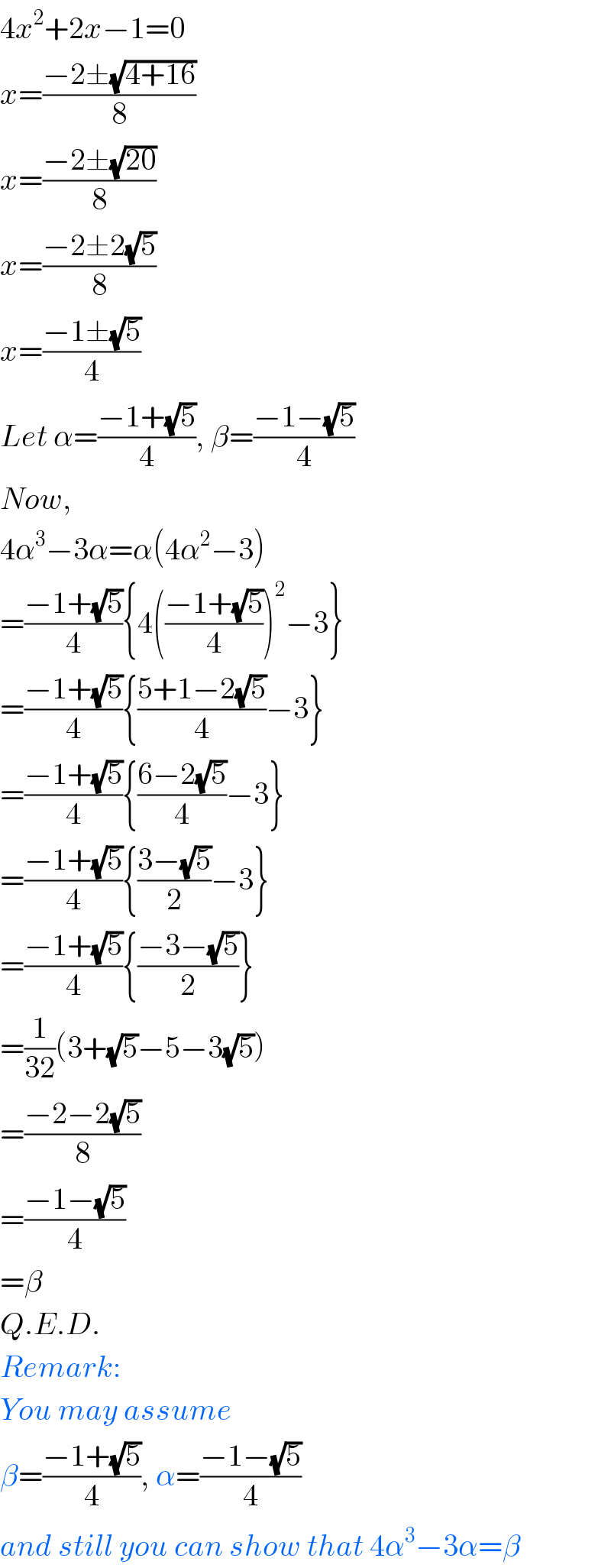
4x^2 +2x−1=0 x=((−2±(√(4+16)))/8) x=((−2±(√(20)))/8) x=((−2±2(√5))/8) x=((−1±(√5))/4) Let α=((−1+(√5))/4), β=((−1−(√5))/4) Now, 4α^3 −3α=α(4α^2 −3) =((−1+(√5))/4){4(((−1+(√5))/4))^2 −3} =((−1+(√5))/4){((5+1−2(√5))/4)−3} =((−1+(√5))/4){((6−2(√5))/4)−3} =((−1+(√5))/4){((3−(√5))/2)−3} =((−1+(√5))/4){((−3−(√5))/2)} =(1/(32))(3+(√5)−5−3(√5)) =((−2−2(√5))/8) =((−1−(√5))/4) =β Q.E.D. Remark: You may assume β=((−1+(√5))/4), α=((−1−(√5))/4) and still you can show that 4α^3 −3α=β
4x2+2x−1=0x=−2±4+168x=−2±208x=−2±258x=−1±54Letα=−1+54,β=−1−54Now,4α3−3α=α(4α2−3)=−1+54{4(−1+54)2−3}=−1+54{5+1−254−3}=−1+54{6−254−3}=−1+54{3−52−3}=−1+54{−3−52}=132(3+5−5−35)=−2−258=−1−54=βQ.E.D.Remark:Youmayassumeβ=−1+54,α=−1−54andstillyoucanshowthat4α3−3α=β
Commented by 1549442205PVT last updated on 12/Sep/20
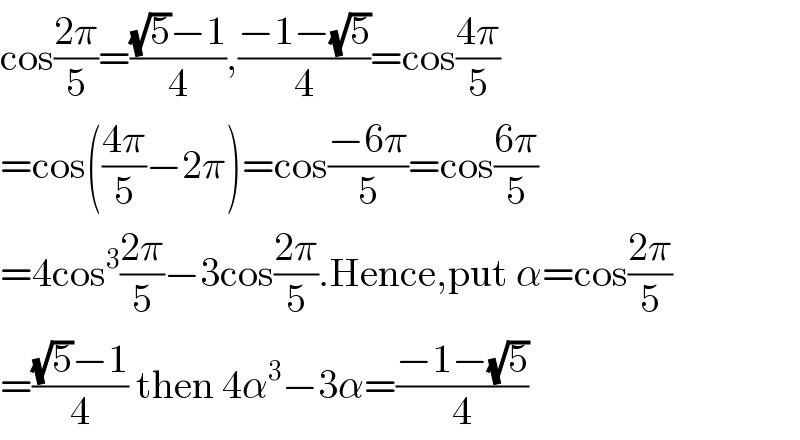
cos((2π)/5)=(((√5)−1)/4),((−1−(√5))/4)=cos((4π)/5) =cos(((4π)/5)−2π)=cos((−6π)/5)=cos((6π)/5) =4cos^3 ((2π)/5)−3cos((2π)/5).Hence,put α=cos((2π)/5) =(((√5)−1)/4) then 4α^3 −3α=((−1−(√5))/4)
cos2π5=5−14,−1−54=cos4π5=cos(4π5−2π)=cos−6π5=cos6π5=4cos32π5−3cos2π5.Hence,putα=cos2π5=5−14then4α3−3α=−1−54
Answered by Dwaipayan Shikari last updated on 11/Sep/20
α+β=−(1/2) αβ=−(1/4) (α+β)^2 −4αβ=(α−β)^2 (1/4)+1=(α−β)^2 ⇒(α−β)=±((√5)/2) α=(((√5)−1)/4) or−((((√5)+1)/4)) β=((−(√5)−1)/4) or ((((√5)−1)/4)) 4α^3 −3α=−4((((√5)+1)/4))^3 +3((((√5)+1)/4))=(((√5)−1)/4)=β 4α^3 −3α=4((((√5)−1)/4))^3 −3((((√5)−1)/4))=β
α+β=−12αβ=−14(α+β)2−4αβ=(α−β)214+1=(α−β)2⇒(α−β)=±52α=5−14or−(5+14)β=−5−14or(5−14)4α3−3α=−4(5+14)3+3(5+14)=5−14=β4α3−3α=4(5−14)3−3(5−14)=β

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *