Question Number 165044 by alephzero last updated on 25/Jan/22
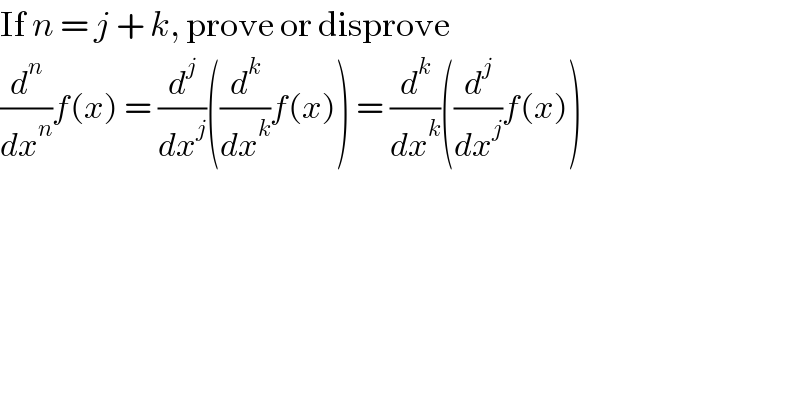
$$\mathrm{If}\:{n}\:=\:{j}\:+\:{k},\:\mathrm{prove}\:\mathrm{or}\:\mathrm{disprove} \\ $$$$\frac{{d}^{{n}} }{{dx}^{{n}} }{f}\left({x}\right)\:=\:\frac{{d}^{{j}} }{{dx}^{{j}} }\left(\frac{{d}^{{k}} }{{dx}^{{k}} }{f}\left({x}\right)\right)\:=\:\frac{{d}^{{k}} }{{dx}^{{k}} }\left(\frac{{d}^{{j}} }{{dx}^{{j}} }{f}\left({x}\right)\right) \\ $$
Commented by mr W last updated on 25/Jan/22
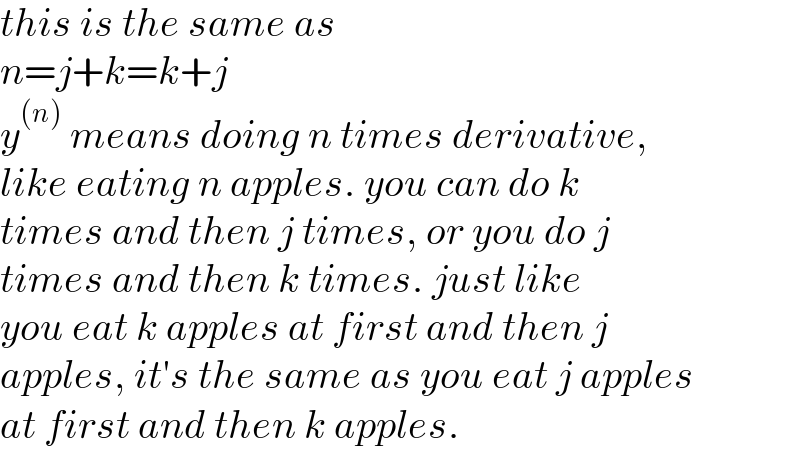
$${this}\:{is}\:{the}\:{same}\:{as} \\ $$$${n}={j}+{k}={k}+{j} \\ $$$${y}^{\left({n}\right)} \:{means}\:{doing}\:{n}\:{times}\:{derivative}, \\ $$$${like}\:{eating}\:{n}\:{apples}.\:{you}\:{can}\:{do}\:{k} \\ $$$${times}\:{and}\:{then}\:{j}\:{times},\:{or}\:{you}\:{do}\:{j} \\ $$$${times}\:{and}\:{then}\:{k}\:{times}.\:{just}\:{like} \\ $$$${you}\:{eat}\:{k}\:{apples}\:{at}\:{first}\:{and}\:{then}\:{j}\: \\ $$$${apples},\:{it}'{s}\:{the}\:{same}\:{as}\:{you}\:{eat}\:{j}\:{apples}\: \\ $$$${at}\:{first}\:{and}\:{then}\:{k}\:{apples}. \\ $$
Commented by alephzero last updated on 25/Jan/22

$$\mathrm{Thank}\:\mathrm{you},\:\mathrm{mr}\:\boldsymbol{\mathcal{W}} \\ $$
