Question Number 128712 by Study last updated on 09/Jan/21
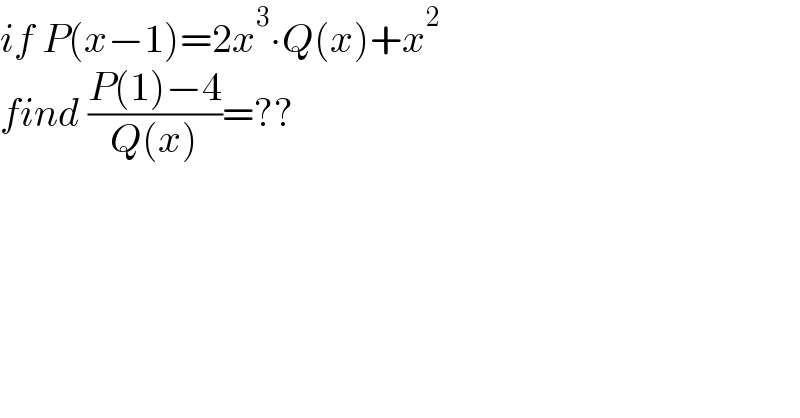
Commented by Adel last updated on 13/Jan/21
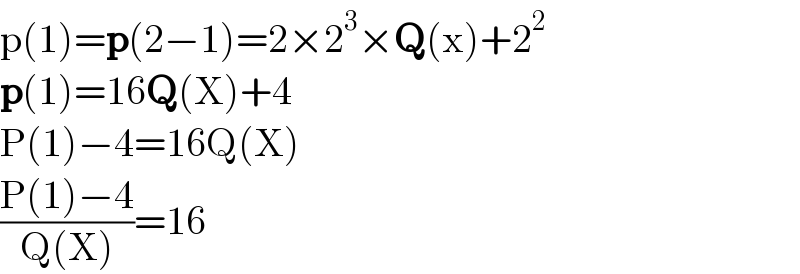
Answered by essafty last updated on 09/Jan/21

Commented by Study last updated on 09/Jan/21
Answered by hknkrc46 last updated on 09/Jan/21
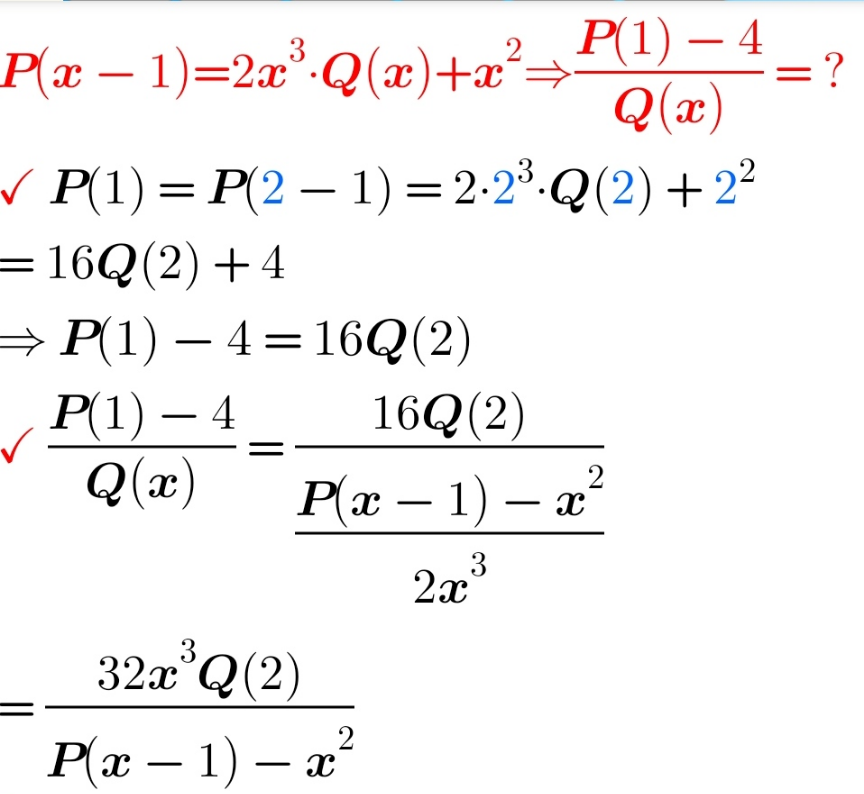
Commented by Study last updated on 10/Jan/21
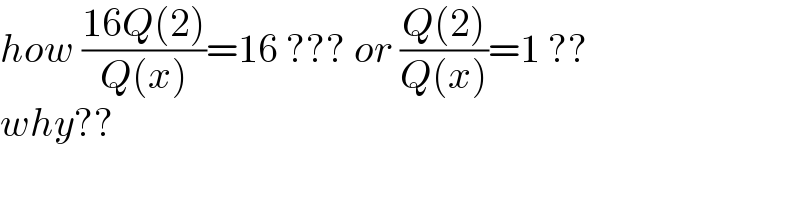
Commented by Study last updated on 11/Jan/21

Commented by Study last updated on 11/Jan/21

