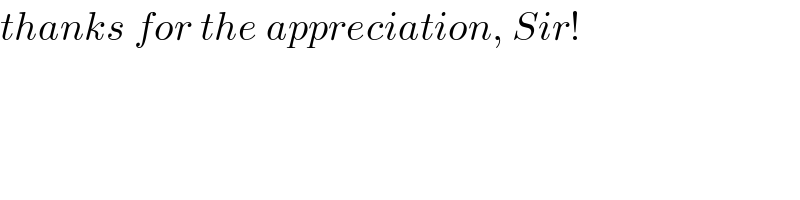if-point-of-intersection-of-curves-C-1-x-2-4y-2-2xy-9x-3-and-C-2-2x-2-3y-2-4xy-3x-1-subtends-a-right-angle-at-origin-the-value-of-is-

Question Number 31147 by momo last updated on 03/Mar/18

i f p o i n t o f i n t e r s e c t i o n o f c u r v e s

C_{1} = λ x^{2} + 4 y^{2} - 2 x y - 9 x + 3 a n d

C_{2} = 2 x^{2} + 3 y^{2} - 4 x y + 3 x - 1

s u b t e n d s a r i g h t a n g l e a t o r i g i n t h e

v a l u e o f λ i s ?

Answered by ajfour last updated on 03/Mar/18

\frac{y_{1}}{x_{1}} = m_{1} a n d \frac{y_{2}}{x_{2}} = m_{2} (s a y)

m_{1} m_{2} = - 1

A s p o i n t s o f i n t e r s e c t i o n s a y

A (x_{1}, y_{1}) a n d B (x_{2}, y_{2}) l i e s o n

b o t h c u r v e s

C_{1} + 3 C_{2} g i v e s

(λ + 6) x^{2} + 13 y^{2} - 14 x y = 0

\Rightarrow (λ + 6) + 13 {(\frac{y}{x})}^{2} - 14 (\frac{y}{x}) = 0

a s m_{1}, m_{2} a r e r o o t s o f t h i s e q .

m_{1} m_{2} = \frac{λ + 6}{13} = - 1

\Rightarrow λ = - 19 .

Commented by momo last updated on 03/Mar/18

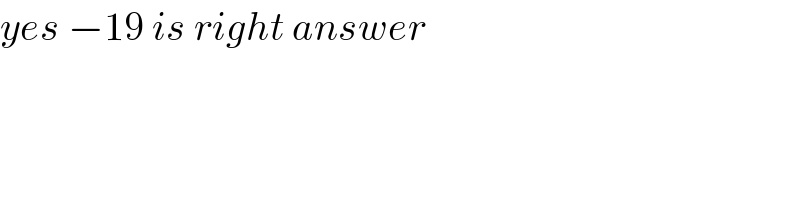
y e s - 19 i s r i g h t a n s w e r

Commented by momo last updated on 03/Mar/18

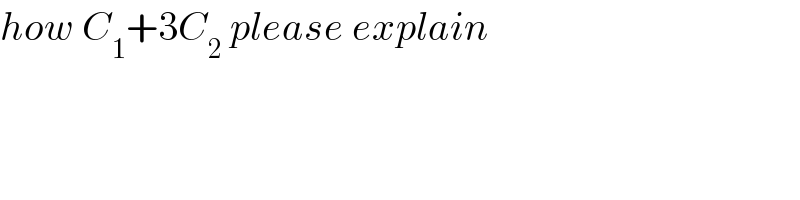
h o w C_{1} + 3 C_{2} p l e a s e e x p l a i n

Commented by ajfour last updated on 03/Mar/18

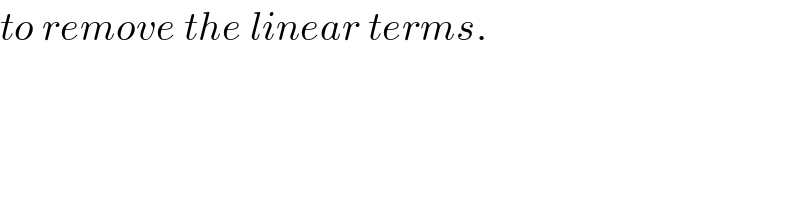
t o r e m o v e t h e l i n e a r t e r m s .

Commented by ajfour last updated on 03/Mar/18

i n E n g l i s h s i r ?

Commented by mrW2 last updated on 03/Mar/18

Ausgezeichnet!

Commented by mrW2 last updated on 03/Mar/18

Excellent! Outstanding!

Commented by ajfour last updated on 03/Mar/18

t h a n k s f o r t h e a p p r e c i a t i o n, S i r!

Facebook Tweet Pin