If-polynomial-x-3-9x-2-11x-1-0-have-the-roots-are-a-b-an-c-Given-a-b-c-then-4-18-2-8-

Question Number 166958 by cortano1 last updated on 03/Mar/22

If polynomial x^{3} - {9 x}^{2} + 11 x - 1 = 0

have the roots are a, b an c .

Given Δ = \sqrt{a} + \sqrt{b} + \sqrt{c} then

Δ^{4} - 18 Δ^{2} - 8 Δ = ?

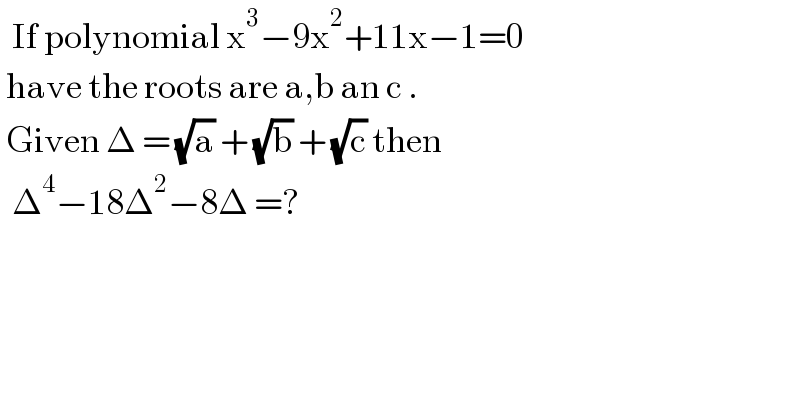
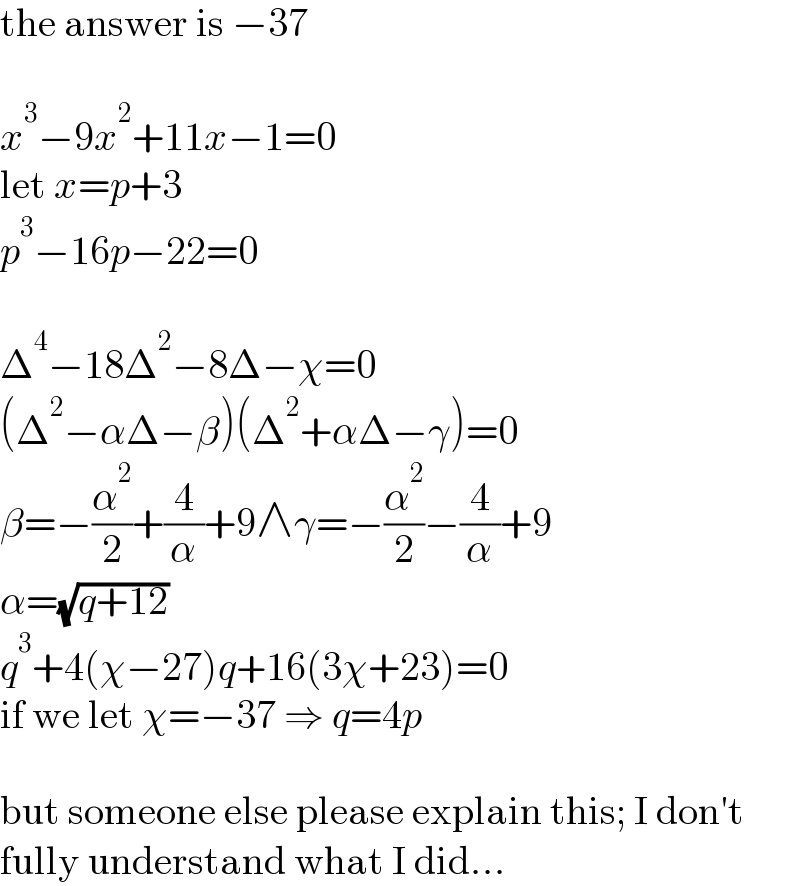
Commented by MJS_new last updated on 03/Mar/22

the answer is - 37

x^{3} - 9 x^{2} + 11 x - 1 = 0

let x = p + 3

p^{3} - 16 p - 22 = 0

Δ^{4} - 18 Δ^{2} - 8 Δ - χ = 0

(Δ^{2} - α Δ - β) (Δ^{2} + α Δ - γ) = 0

β = - \frac{α^{2}}{2} + \frac{4}{α} + 9 \land γ = - \frac{α^{2}}{2} - \frac{4}{α} + 9

α = \sqrt{q + 12}

q^{3} + 4 (χ - 27) q + 16 (3 χ + 23) = 0

if we let χ = - 37 \Rightarrow q = 4 p

but someone else please explain this; I {don}^{'} t

fully understand what I did \dots

Answered by mr W last updated on 03/Mar/22

a + b + c = 9

a b + b c + c a = 11

a b c = 1

Δ = \sqrt{a} + \sqrt{b} + \sqrt{c}

Δ^{2} = a + b + c + 2 (\sqrt{a b} + \sqrt{b c} + \sqrt{c a})

Δ^{2} = 9 + 2 (\sqrt{a b} + \sqrt{b c} + \sqrt{c a})

Δ^{4} = 81 + 36 (\sqrt{a b} + \sqrt{b c} + \sqrt{c a}) + 4 (a b + b c + c a + 2 \sqrt{a^{2} b c} + 2 \sqrt{{a b}^{2} c} + 2 \sqrt{{a b c}^{2}})

Δ^{4} = 81 + 36 (\sqrt{a b} + \sqrt{b c} + \sqrt{c a}) + 4 (11 + 2 \sqrt{a} + 2 \sqrt{b} + 2 \sqrt{c})

Δ^{4} = 125 + 36 (\sqrt{a b} + \sqrt{b c} + \sqrt{c a}) + 8 (\sqrt{a} + \sqrt{b} + \sqrt{c})

Δ^{4} - 18 Δ^{2} - 8 Δ

= 125 + 36 (\sqrt{a b} + \sqrt{b c} + \sqrt{c a}) + 8 (\sqrt{a} + \sqrt{b} + \sqrt{c})

- 18 \times 9 - 18 \times 2 (\sqrt{a b} + \sqrt{b c} + \sqrt{c a})

- 8 \times (\sqrt{a} + \sqrt{b} + \sqrt{c})

= 125 - 18 \times 9

= - 37

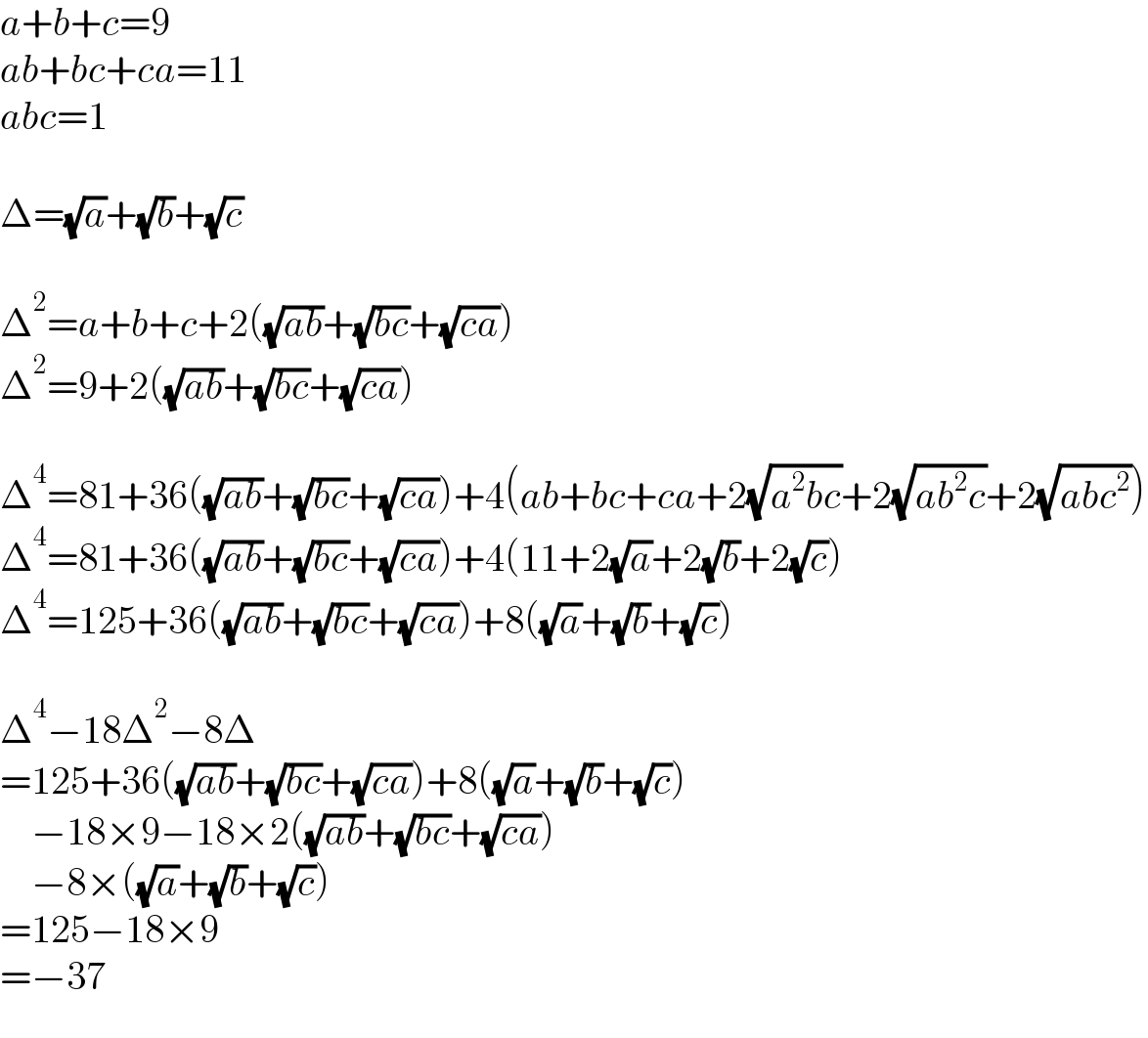
Answered by null last updated on 03/Mar/22

u p s i l o n

Answered by null last updated on 04/Mar/22

upsilon = - 37

υ = - 37

Facebook Tweet Pin