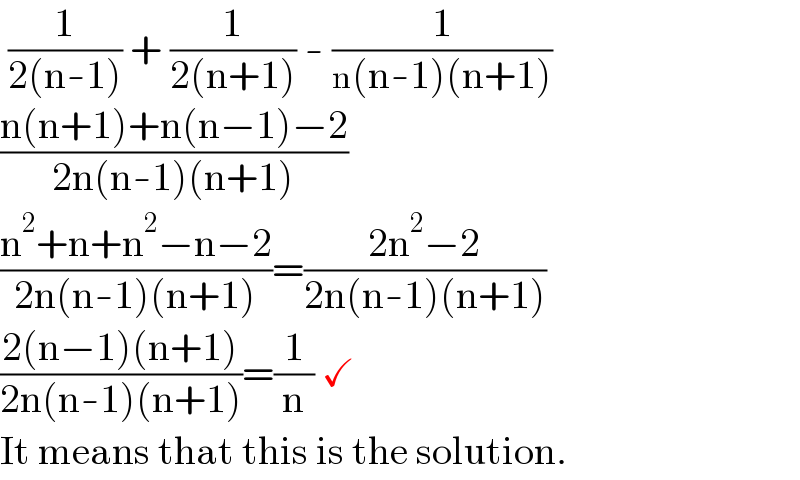Question Number 149567 by mathdanisur last updated on 06/Aug/21
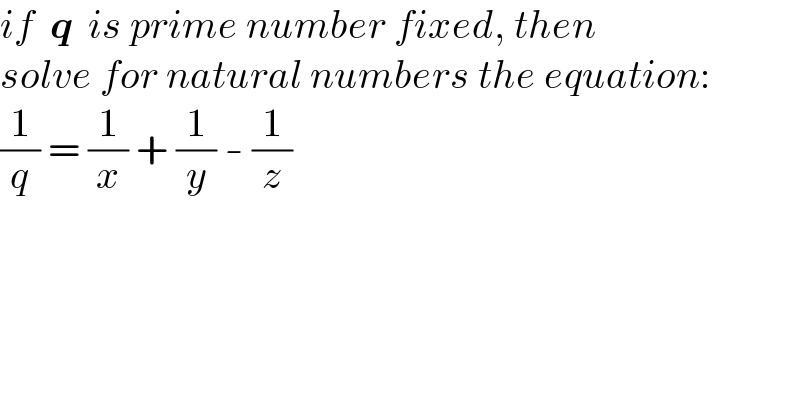
Commented by Rasheed.Sindhi last updated on 07/Aug/21

Commented by mathdanisur last updated on 07/Aug/21
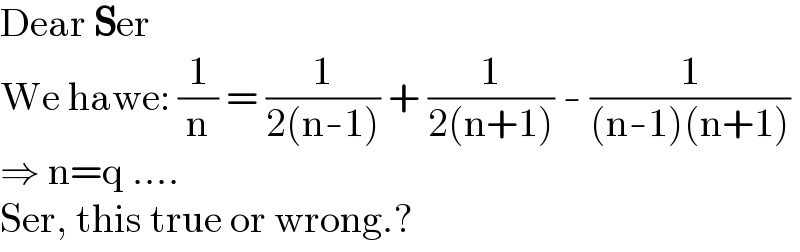
Commented by Rasheed.Sindhi last updated on 07/Aug/21
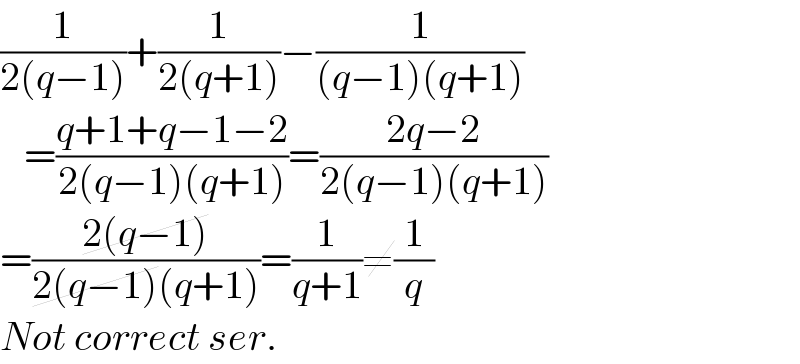
Commented by mathdanisur last updated on 07/Aug/21

Commented by Rasheed.Sindhi last updated on 07/Aug/21