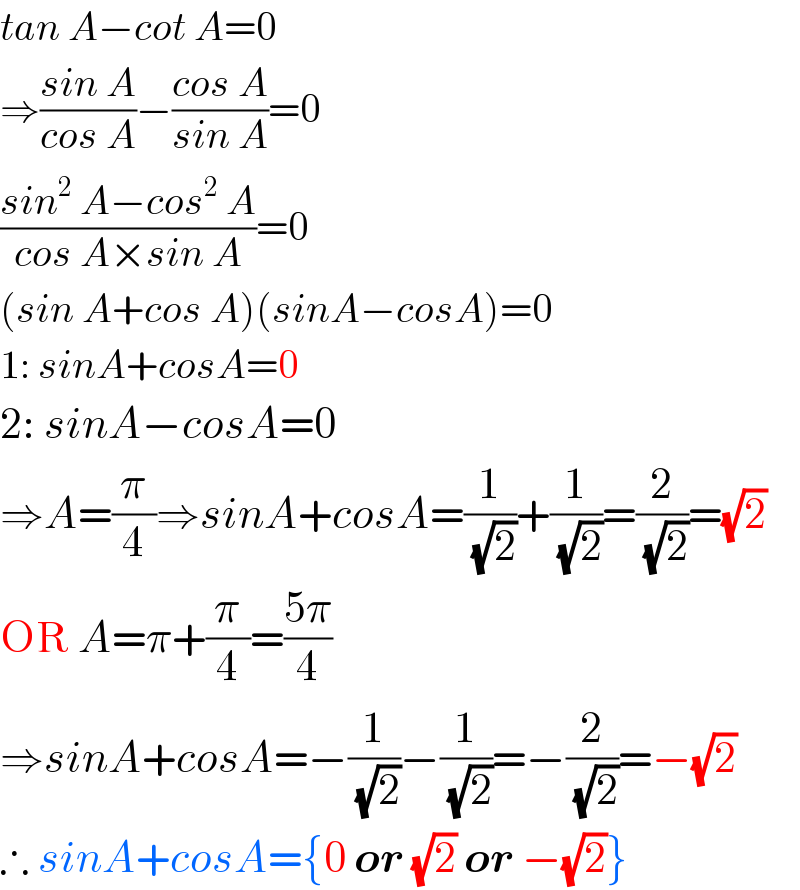Menu Close

if-tan-A-cot-A-0-prove-that-sin-A-cos-A-

Tinku Tara 0 Comments
Pin




Question Number 60269 by Askash last updated on 19/May/19
if tan A − cot A = 0 prove that sin A + cos A=?
$${if} \\ $$$${tan}\:{A}\:−\:{cot}\:{A}\:=\:\mathrm{0} \\ $$$${prove}\:{that} \\ $$$${sin}\:{A}\:+\:{cos}\:{A}=? \\ $$
Commented by mr W last updated on 19/May/19
sin A+cos A=0 or (√2) or −(√2)
$$\mathrm{sin}\:{A}+\mathrm{cos}\:{A}=\mathrm{0}\:{or}\:\sqrt{\mathrm{2}}\:{or}\:−\sqrt{\mathrm{2}} \\ $$
Commented by Askash last updated on 19/May/19
how − (√(2 )) is possible
$${how}\:−\:\sqrt{\mathrm{2}\:\:} \\ $$$${is}\:{possible} \\ $$
Commented by mr W last updated on 19/May/19
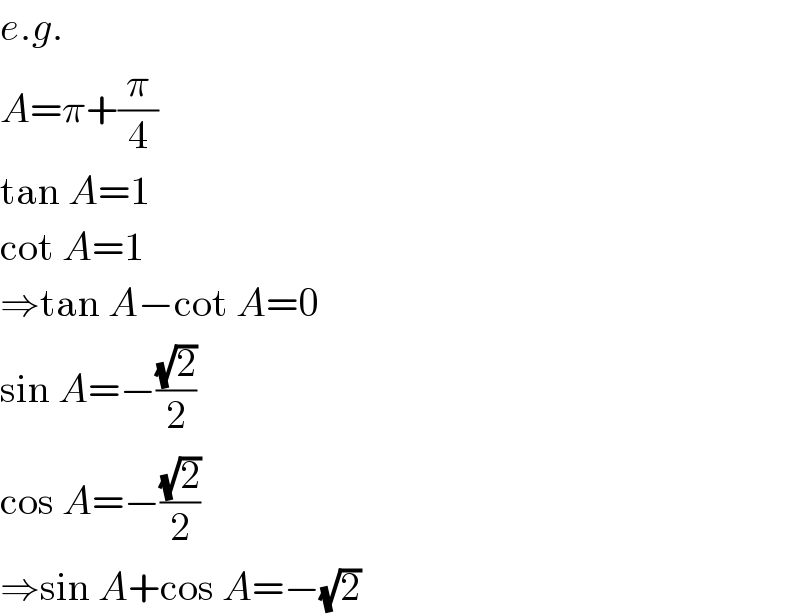
e.g. A=π+(π/4) tan A=1 cot A=1 ⇒tan A−cot A=0 sin A=−((√2)/2) cos A=−((√2)/2) ⇒sin A+cos A=−(√2)
$${e}.{g}. \\ $$$${A}=\pi+\frac{\pi}{\mathrm{4}} \\ $$$$\mathrm{tan}\:{A}=\mathrm{1} \\ $$$$\mathrm{cot}\:{A}=\mathrm{1} \\ $$$$\Rightarrow\mathrm{tan}\:{A}−\mathrm{cot}\:{A}=\mathrm{0} \\ $$$$\mathrm{sin}\:{A}=−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\mathrm{cos}\:{A}=−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{sin}\:{A}+\mathrm{cos}\:{A}=−\sqrt{\mathrm{2}} \\ $$
Answered by Kunal12588 last updated on 19/May/19
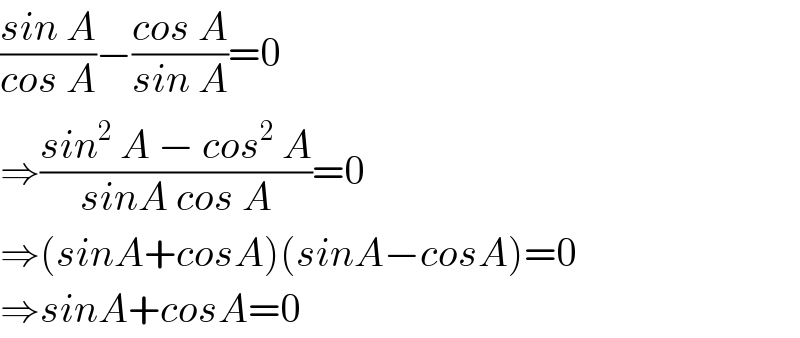
((sin A)/(cos A))−((cos A)/(sin A))=0 ⇒((sin^2 A − cos^2 A)/(sinA cos A))=0 ⇒(sinA+cosA)(sinA−cosA)=0 ⇒sinA+cosA=0
$$\frac{{sin}\:{A}}{{cos}\:{A}}−\frac{{cos}\:{A}}{{sin}\:{A}}=\mathrm{0} \\ $$$$\Rightarrow\frac{{sin}^{\mathrm{2}} \:{A}\:−\:{cos}^{\mathrm{2}} \:{A}}{{sinA}\:{cos}\:{A}}=\mathrm{0} \\ $$$$\Rightarrow\left({sinA}+{cosA}\right)\left({sinA}−{cosA}\right)=\mathrm{0} \\ $$$$\Rightarrow{sinA}+{cosA}=\mathrm{0} \\ $$
Commented by Askash last updated on 19/May/19
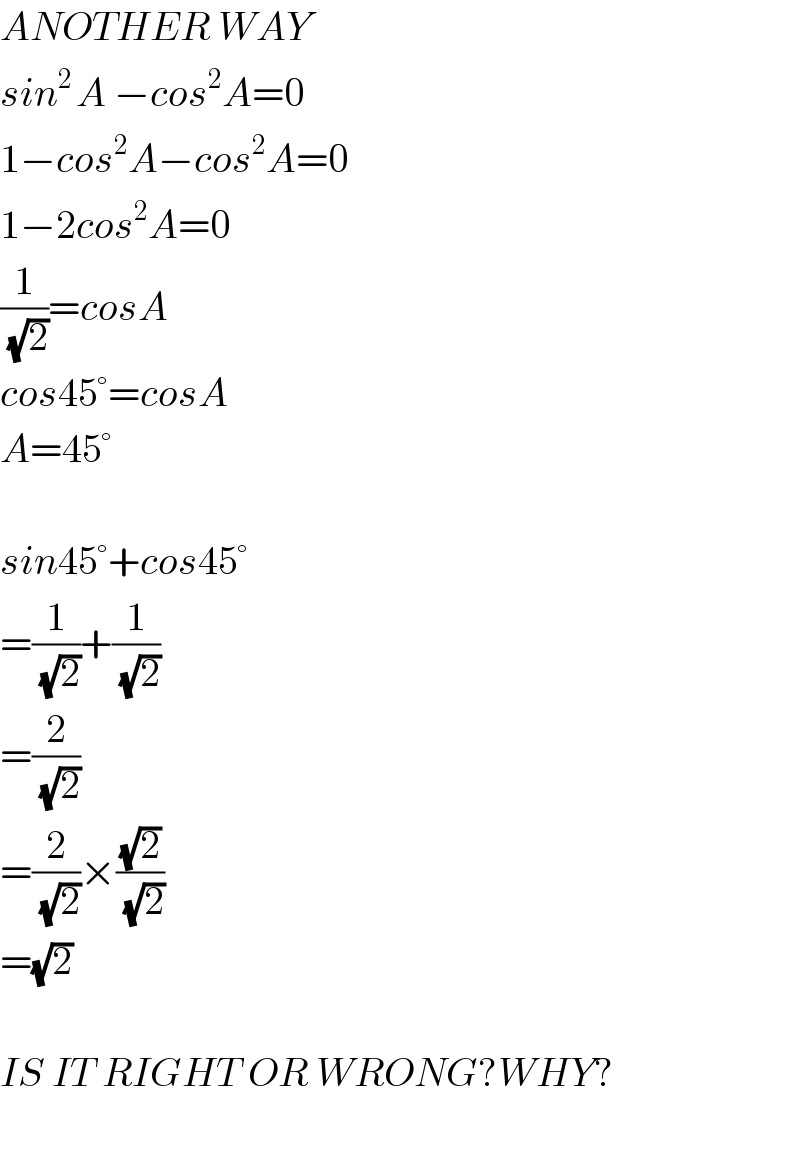
ANOTHER WAY sin^(2 ) A −cos^2 A=0 1−cos^2 A−cos^2 A=0 1−2cos^2 A=0 (1/( (√2)))=cosA cos45°=cosA A=45° sin45°+cos45° =(1/( (√2)))+(1/( (√2))) =(2/( (√2))) =(2/( (√2)))×((√2)/( (√2))) =(√2) IS IT RIGHT OR WRONG?WHY?
$${ANOTHER}\:{WAY} \\ $$$${sin}^{\mathrm{2}\:} {A}\:−{cos}^{\mathrm{2}} {A}=\mathrm{0} \\ $$$$\mathrm{1}−{cos}^{\mathrm{2}} {A}−{cos}^{\mathrm{2}} {A}=\mathrm{0} \\ $$$$\mathrm{1}−\mathrm{2}{cos}^{\mathrm{2}} {A}=\mathrm{0} \\ $$$$\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}={cosA} \\ $$$${cos}\mathrm{45}°={cosA} \\ $$$${A}=\mathrm{45}° \\ $$$$ \\ $$$${sin}\mathrm{45}°+{cos}\mathrm{45}° \\ $$$$=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}} \\ $$$$=\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}}} \\ $$$$=\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}}}×\frac{\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{2}}} \\ $$$$=\sqrt{\mathrm{2}} \\ $$$$ \\ $$$${IS}\:{IT}\:{RIGHT}\:{OR}\:{WRONG}?{WHY}? \\ $$$$ \\ $$
Commented by Kunal12588 last updated on 19/May/19
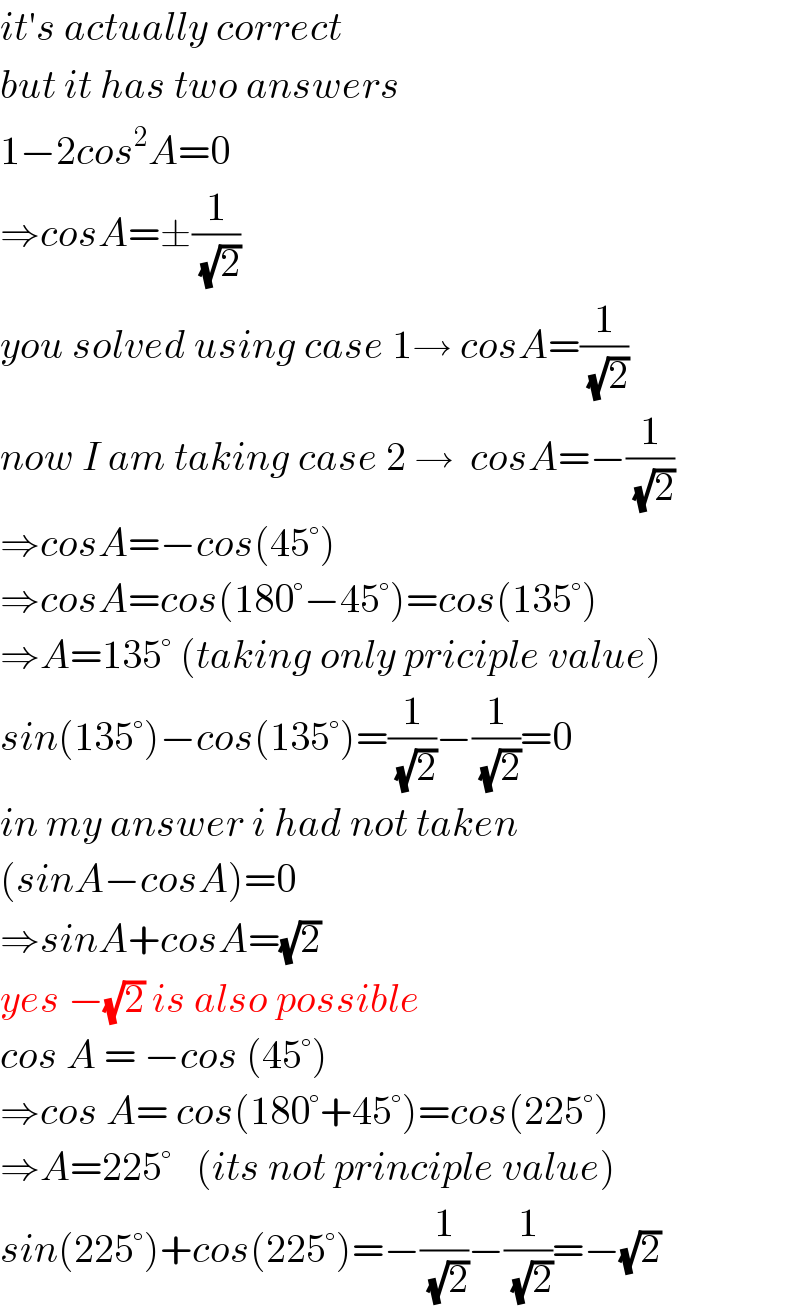
it′s actually correct but it has two answers 1−2cos^2 A=0 ⇒cosA=±(1/( (√2))) you solved using case 1→ cosA=(1/( (√2))) now I am taking case 2 → cosA=−(1/( (√2))) ⇒cosA=−cos(45°) ⇒cosA=cos(180°−45°)=cos(135°) ⇒A=135° (taking only priciple value) sin(135°)−cos(135°)=(1/( (√2)))−(1/( (√2)))=0 in my answer i had not taken (sinA−cosA)=0 ⇒sinA+cosA=(√2) yes −(√2) is also possible cos A = −cos (45°) ⇒cos A= cos(180°+45°)=cos(225°) ⇒A=225° (its not principle value) sin(225°)+cos(225°)=−(1/( (√2)))−(1/( (√2)))=−(√2)
$${it}'{s}\:{actually}\:{correct} \\ $$$${but}\:{it}\:{has}\:{two}\:{answers} \\ $$$$\mathrm{1}−\mathrm{2}{cos}^{\mathrm{2}} {A}=\mathrm{0} \\ $$$$\Rightarrow{cosA}=\pm\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}} \\ $$$${you}\:{solved}\:{using}\:{case}\:\mathrm{1}\rightarrow\:{cosA}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}} \\ $$$${now}\:{I}\:{am}\:{taking}\:{case}\:\mathrm{2}\:\rightarrow\:\:{cosA}=−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}} \\ $$$$\Rightarrow{cosA}=−{cos}\left(\mathrm{45}°\right) \\ $$$$\Rightarrow{cosA}={cos}\left(\mathrm{180}°−\mathrm{45}°\right)={cos}\left(\mathrm{135}°\right) \\ $$$$\Rightarrow{A}=\mathrm{135}°\:\left({taking}\:{only}\:{priciple}\:{value}\right) \\ $$$${sin}\left(\mathrm{135}°\right)−{cos}\left(\mathrm{135}°\right)=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}=\mathrm{0} \\ $$$${in}\:{my}\:{answer}\:{i}\:{had}\:{not}\:{taken}\: \\ $$$$\left({sinA}−{cosA}\right)=\mathrm{0} \\ $$$$\Rightarrow{sinA}+{cosA}=\sqrt{\mathrm{2}} \\ $$$${yes}\:−\sqrt{\mathrm{2}}\:{is}\:{also}\:{possible} \\ $$$${cos}\:{A}\:=\:−{cos}\:\left(\mathrm{45}°\right) \\ $$$$\Rightarrow{cos}\:{A}=\:{cos}\left(\mathrm{180}°+\mathrm{45}°\right)={cos}\left(\mathrm{225}°\right) \\ $$$$\Rightarrow{A}=\mathrm{225}°\:\:\:\left({its}\:{not}\:{principle}\:{value}\right) \\ $$$${sin}\left(\mathrm{225}°\right)+{cos}\left(\mathrm{225}°\right)=−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}=−\sqrt{\mathrm{2}} \\ $$
Commented by mr W last updated on 19/May/19
what about A=225° ?
$${what}\:{about}\:{A}=\mathrm{225}°\:? \\ $$
Commented by Kunal12588 last updated on 19/May/19
thank you sir
$${thank}\:{you}\:{sir} \\ $$
Answered by ajfour last updated on 19/May/19
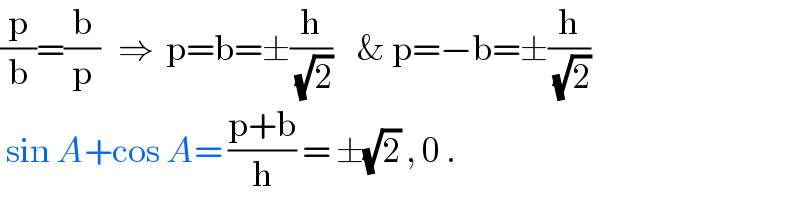
(p/b)=(b/p) ⇒ p=b=±(h/( (√2))) & p=−b=±(h/( (√2))) sin A+cos A= ((p+b)/h) = ±(√2) , 0 .
$$\frac{\mathrm{p}}{\mathrm{b}}=\frac{\mathrm{b}}{\mathrm{p}}\:\:\:\Rightarrow\:\:\mathrm{p}=\mathrm{b}=\pm\frac{\mathrm{h}}{\:\sqrt{\mathrm{2}}}\:\:\:\:\&\:\mathrm{p}=−\mathrm{b}=\pm\frac{\mathrm{h}}{\:\sqrt{\mathrm{2}}} \\ $$$$\:\mathrm{sin}\:{A}+\mathrm{cos}\:{A}=\:\frac{\mathrm{p}+\mathrm{b}}{\mathrm{h}}\:=\:\pm\sqrt{\mathrm{2}}\:,\:\mathrm{0}\:. \\ $$
Commented by Kunal12588 last updated on 19/May/19
great i used this method to prove the trig identities in first year but teachers always deduct my marks.
$${great}\:{i}\:{used}\:{this}\:{method}\:{to}\:{prove}\:{the}\: \\ $$$${trig}\:{identities}\:{in}\:{first}\:{year}\:{but}\:{teachers} \\ $$$${always}\:{deduct}\:{my}\:{marks}. \\ $$
Commented by Askash last updated on 20/May/19
Same with me. Some Teachers only check their solutions what they had taught.
$${Same}\:{with}\:{me}.\: \\ $$$${Some}\:{Teachers}\:{only}\:{check}\:{their} \\ $$$${solutions}\:{what}\:{they}\:{had}\:{taught}. \\ $$
Answered by malwaan last updated on 22/May/19
tan A−cot A=0 ⇒((sin A)/(cos A))−((cos A)/(sin A))=0 ((sin^2 A−cos^2 A)/(cos A×sin A))=0 (sin A+cos A)(sinA−cosA)=0 1: sinA+cosA=0 2: sinA−cosA=0 ⇒A=(π/4)⇒sinA+cosA=(1/( (√2)))+(1/( (√2)))=(2/( (√2)))=(√2) OR A=π+(π/4)=((5π)/4) ⇒sinA+cosA=−(1/( (√2)))−(1/( (√2)))=−(2/( (√2)))=−(√2) ∴ sinA+cosA={0 or (√2) or −(√2)}
$${tan}\:{A}−{cot}\:{A}=\mathrm{0} \\ $$$$\Rightarrow\frac{{sin}\:{A}}{{cos}\:{A}}−\frac{{cos}\:{A}}{{sin}\:{A}}=\mathrm{0} \\ $$$$\frac{{sin}^{\mathrm{2}} \:{A}−{cos}^{\mathrm{2}} \:{A}}{{cos}\:{A}×{sin}\:{A}}=\mathrm{0} \\ $$$$\left({sin}\:{A}+{cos}\:{A}\right)\left({sinA}−{cosA}\right)=\mathrm{0} \\ $$$$\mathrm{1}:\:{sinA}+{cosA}=\mathrm{0} \\ $$$$\mathrm{2}:\:{sinA}−{cosA}=\mathrm{0} \\ $$$$\Rightarrow{A}=\frac{\pi}{\mathrm{4}}\Rightarrow{sinA}+{cosA}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}}}=\sqrt{\mathrm{2}} \\ $$$$\mathrm{OR}\:{A}=\pi+\frac{\pi}{\mathrm{4}}=\frac{\mathrm{5}\pi}{\mathrm{4}} \\ $$$$\Rightarrow{sinA}+{cosA}=−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}=−\frac{\mathrm{2}}{\:\sqrt{\mathrm{2}}}=−\sqrt{\mathrm{2}} \\ $$$$\therefore\:{sinA}+{cosA}=\left\{\mathrm{0}\:\boldsymbol{{or}}\:\sqrt{\mathrm{2}}\:\boldsymbol{{or}}\:−\sqrt{\mathrm{2}}\right\} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *