Question Number 148987 by mathdanisur last updated on 02/Aug/21

$${if}\:\:\:{tg}\left(\mathrm{0},\mathrm{5}{x}\right)\:=\:−\mathrm{2} \\ $$$${find}\:\:\:\frac{{sin}\left({x}\right)\:+\:\mathrm{2}}{{cos}\left({x}\right)\:-\:\mathrm{3}}\:=\:? \\ $$
Answered by EDWIN88 last updated on 02/Aug/21
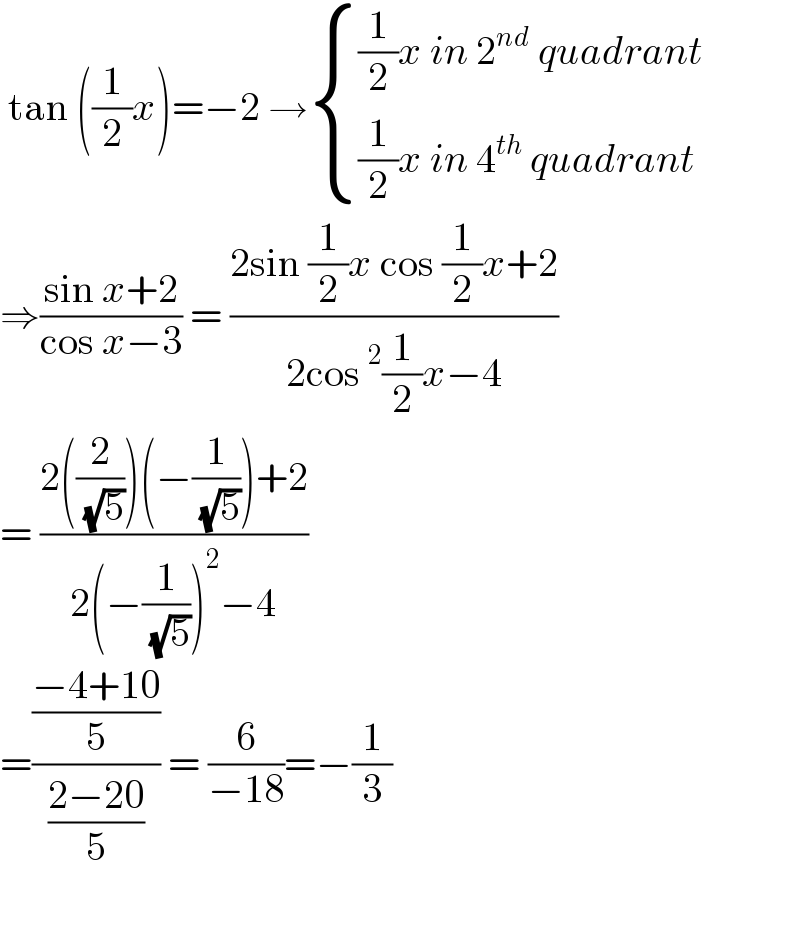
$$\:\mathrm{tan}\:\left(\frac{\mathrm{1}}{\mathrm{2}}{x}\right)=−\mathrm{2}\:\rightarrow\begin{cases}{\frac{\mathrm{1}}{\mathrm{2}}{x}\:{in}\:\mathrm{2}^{{nd}} \:{quadrant}}\\{\frac{\mathrm{1}}{\mathrm{2}}{x}\:{in}\:\mathrm{4}^{{th}} \:{quadrant}}\end{cases} \\ $$$$\Rightarrow\frac{\mathrm{sin}\:{x}+\mathrm{2}}{\mathrm{cos}\:{x}−\mathrm{3}}\:=\:\frac{\mathrm{2sin}\:\frac{\mathrm{1}}{\mathrm{2}}{x}\:\mathrm{cos}\:\frac{\mathrm{1}}{\mathrm{2}}{x}+\mathrm{2}}{\mathrm{2cos}\:^{\mathrm{2}} \frac{\mathrm{1}}{\mathrm{2}}{x}−\mathrm{4}} \\ $$$$=\:\frac{\mathrm{2}\left(\frac{\mathrm{2}}{\:\sqrt{\mathrm{5}}}\right)\left(−\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}\right)+\mathrm{2}}{\mathrm{2}\left(−\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}\right)^{\mathrm{2}} −\mathrm{4}} \\ $$$$=\frac{\frac{−\mathrm{4}+\mathrm{10}}{\mathrm{5}}}{\frac{\mathrm{2}−\mathrm{20}}{\mathrm{5}}}\:=\:\frac{\mathrm{6}}{−\mathrm{18}}=−\frac{\mathrm{1}}{\mathrm{3}}\: \\ $$$$ \\ $$
Commented by mathdanisur last updated on 02/Aug/21

$${Thankyou}\:{Ser} \\ $$
