If-the-roots-of-the-equation-24x-4-52x-3-18x-2-13x-6-0-are-and-1-Find-the-value-of-and-

Question Number 119396 by bemath last updated on 24/Oct/20

I f t h e r o o t s o f t h e e q u a t i o n

24 x^{4} - 52 x^{3} + 18 x^{2} + 13 x - 6 = 0 a r e

α, - α, β a n d \frac{1}{β} . F i n d t h e v a l u e o f

α a n d β .

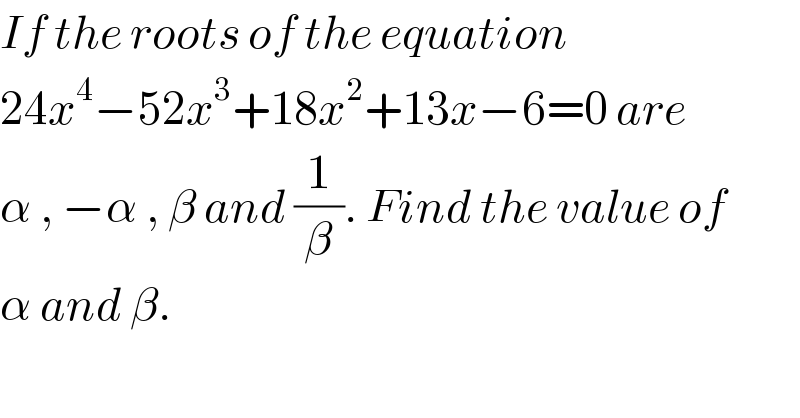
Commented by Dwaipayan Shikari last updated on 24/Oct/20

24 x^{4} - 52 x^{3} + 18 x^{2} + 13 x - 6 = 0

α - α + β + \frac{1}{β} = \frac{52}{24}

24 β^{2} - 52 β + 24 = 0 \Rightarrow 6 β^{2} - 13 β + 6 = 0

β = \frac{13 \pm \sqrt{169 - 144}}{12} = \frac{3}{2} o r \frac{2}{3}

α (- α) β . \frac{1}{β} = - \frac{6}{24}

α = \pm \frac{1}{2}

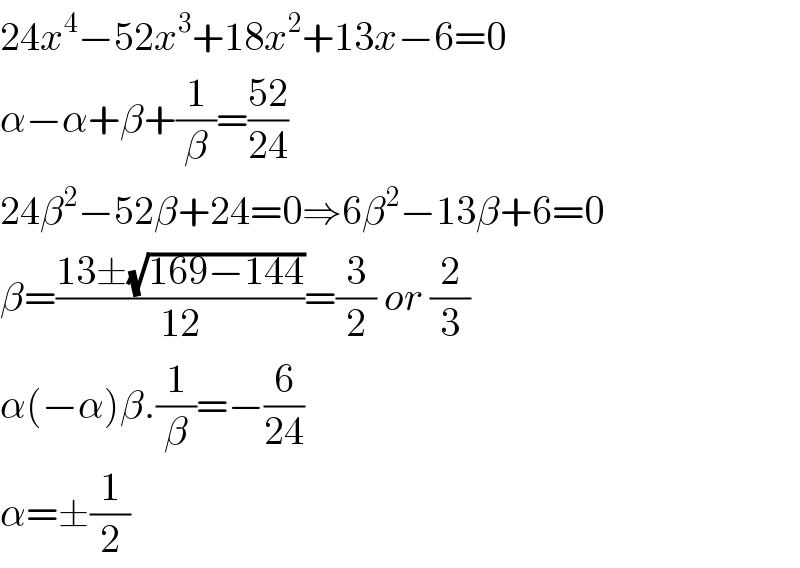
Answered by TANMAY PANACEA last updated on 24/Oct/20

α + (- α) + β + \frac{1}{β} = \frac{- (- 52)}{24} = \frac{13}{6}

6 β^{2} + 6 - 13 β = 0

6 β^{2} - 9 β - 4 β + 6 = 0

3 β (2 β - 3) - 2 (2 β - 3) = 0

(2 β - 3) (3 β - 2) = 0

β = \frac{2}{3} a n d \frac{3}{2}

α \times (- α) \times β \times \frac{1}{β} = \frac{- 6}{24}

α^{2} = \frac{1}{4} \to α = \pm \frac{1}{2}

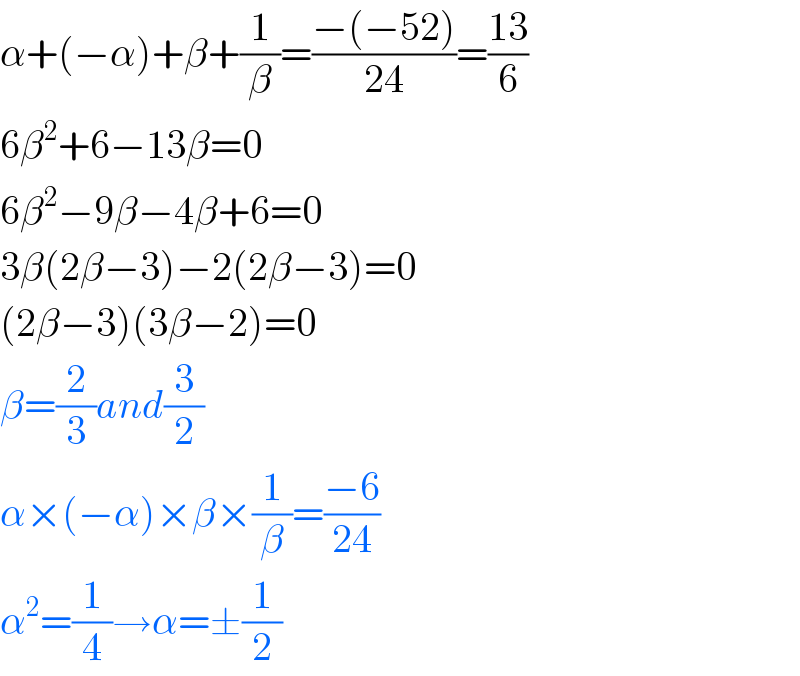
Answered by $@y@m last updated on 24/Oct/20

24 x^{4} - 52 x^{3} + 18 x^{2} + 13 x - 6 = 0 \equiv

24 (x^{2} - α^{2}) {x^{2} - (β + \frac{1}{β}) x + 1}

\Rightarrow 24 α^{2} = 6 \Rightarrow α = \pm \frac{1}{2} A n s P a r t I

A l s o, 24 α^{2} (β + \frac{1}{β}) = 13

β + \frac{1}{β} = \frac{13}{6}

6 β^{2} - 13 β + 6 = 0

β = \frac{13 \pm \sqrt{169 - 144}}{12}

β = \frac{13 \pm 5}{12} = \frac{18}{12}, \frac{8}{12}

β = {\frac{3}{2}}^{'} \frac{2}{3} A n s P a r t II

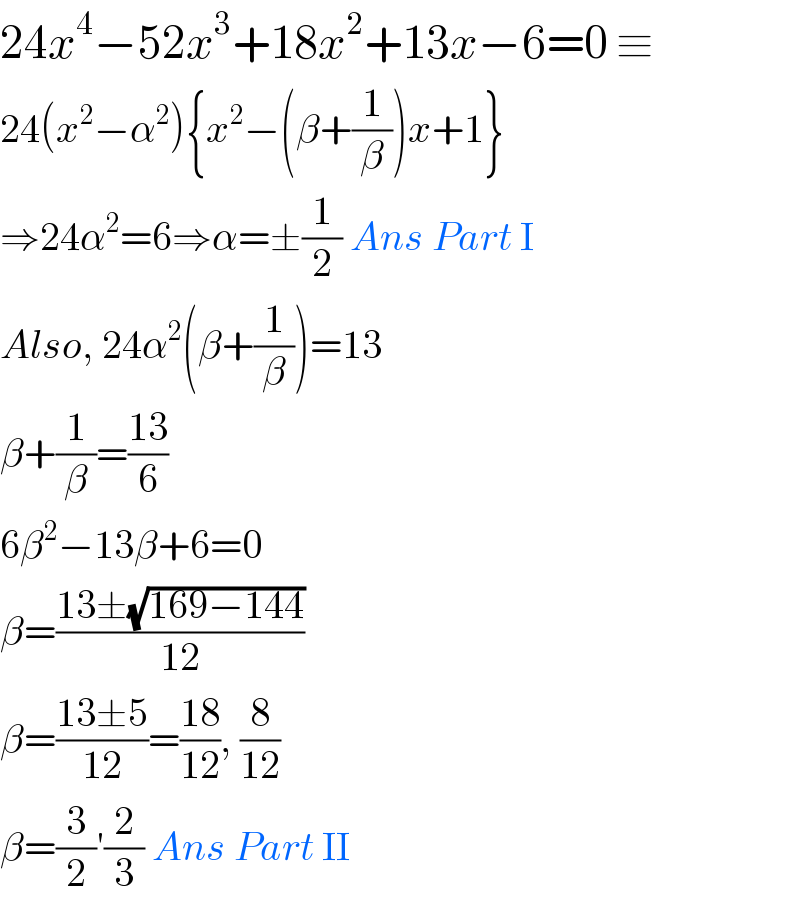
Answered by 1549442205PVT last updated on 24/Oct/20

{24 x}^{4} - {52 x}^{3} + {18 x}^{2} + 13 x - 6

= 24 (x + α) (x - α) (x - β) (x - \frac{1}{β})

= 24 (x^{2} - α^{2}) [x^{2} - (β + \frac{1}{β}) x + 1]

= 24 {x^{4} - [α^{2} (β + \frac{1}{β}) + β + \frac{1}{β}] x^{3}

+ (1 - α^{2}) x^{2} + α^{2} (β + \frac{1}{β}) x - α^{2}}

\Leftrightarrow {\begin{cases} - 24 α^{2} (β + \frac{1}{β}) = - 52 (1) \\ 24 (1 - α^{2}) = 18 (2) \\ 24 α^{2} (β + \frac{1}{β}) = 13 (3) \\ - 24 α^{2} = - 6 (4) \end{cases}

(1) \Rightarrow α^{2} = 1 / 4 \Rightarrow α = \pm 1 / 2 . Replace (1)

(3) we get β + \frac{1}{β} = \frac{13}{6} \Leftrightarrow 6 β^{2} - 13 β + 6 = 0

Δ = 13^{2} - 144 = 25 \Rightarrow β = \frac{13 \pm 5}{12} \in {\frac{3}{2}, \frac{2}{3}}

Thus, α = \pm \frac{1}{2}, β \in {\frac{3}{2}, \frac{2}{3}}