if-the-value-of-x-is-in-degrees-what-is-the-derivative-of-f-x-sin-x-

Question Number 90881 by M±th+et+s last updated on 26/Apr/20

i f t h e v a l u e o f x i s i n d e g r e e s w h a t i s

t h e d e r i v a t i v e o f f (x) = s i n (x)

Commented by mr W last updated on 28/Apr/20

w e h a v e d i f f e r e n t u n d e r s t a n d i n g :

i f x i s i n r a d, t h e s l o p e \frac{d y}{d x} i s i n u n i t

[1 / r a d] . b u t i f x i s i n d e g r e e, t h e

s l o p e i s i n u n i t [1 / d e g r e e] . b o t h s l o p e s

m u s t n o t e q u a l i n v a l u e, b u t e q u a l i n

t h e p h y s i c a l s e n s e .

w h e n w e d e s c r i b e t h e m o t i o n o f a c a r

w i t h y = f (t) = s i n (t) w h e r e t i s i n s e c o n d,

t h e s l o p e \frac{d y}{d t} w h i c h i s t h e s p e e d i n u n i t

[m / s] . b u t i f t i s i n m i l e, t h e s l o p e \frac{d y}{d t}

i s a l s o t h e s p e e d, j u s t i n a n o t h e r

u n i t [m / m i l e] . b o t h a r e o f d i f f e r e n t

v a l u e s, b u t m e a n t h e s a m e p h y s i c a l

s e n s e . t i n f (t) c a n b e i n s e c o n d o r m i l e,

x i n s i n (x) c a n b e i n r a d o r d e g r e e,

w h i c h {d o e n}^{'} t a f f e c t h o w w e c a l c u l a t e

\frac{d y}{d t} o r \frac{d y}{d x} . t h i s i s m y u n d e r s t a n d i n g,

m a y b e c o r r e c t o r m a y b e n o t .

Commented by mr W last updated on 26/Apr/20

\frac{d y}{d x} = \cos (x) n o m a t t e r i f x i s i n r a d o r

i n d e g r e e .

t h i s i s t h e s a m e a s i f s = f (t),

v = \frac{d s}{d t} = f^{'} (t), n o m a t t e r i f t i s i n h o u r

o r s e c o n d .

Commented by M±th+et+s last updated on 26/Apr/20

t h a n k y o u s i r f o r e x p l a i n t h a t

Commented by MJS last updated on 26/Apr/20

Sir, this obviuosly is wrong

the derivate is the slope of the tangent

\frac{d}{d x} [\sin x^{rad}] = \cos x^{rad}

but the slope of \sin x ° is not as steep

\frac{d}{d x} [\sin x °] = \frac{π}{180} \sin x °

generally if f (x °) is any trigonometric

function

\frac{d}{d x} [f (x °)] = \frac{π}{180} f^{'} (x °)

Commented by mr W last updated on 26/Apr/20

i f x i n f (x) i s i n d e g r e e, t h e n t h e x i n

d x i s a l s o i n d e g r e e .

s a y f (x) = \sin (x) w i t h x i n d e g r e e .

t = \frac{x π}{180} \Rightarrow x = \frac{180 t}{π}

\frac{d (\sin x)}{d x} = \frac{d (\sin \frac{180 t}{π})}{\frac{180}{π} d t} = \frac{(\cos \frac{180 t}{π}) \frac{180}{π}}{\frac{180}{π}} = \cos \frac{180 t}{π} = \cos x

i t h i n k i n \frac{d y}{d x} w e s e e o n l y f u n c t i o n, i . e .

t h e m a t h . r e l a t i o n s h i p b e t w e e n y a n d x,

w e {d o n}^{'} t c a r e a b o u t t h e u n i t o f y a n d x .

Commented by MJS last updated on 26/Apr/20

I {don}^{'} t think this is true . we plot the function

in our coordinate system . if the angle is

measured in radiants, the slope of \sin x at

x = 0 is 1 . but if the angle is measured in

degree the slope cannot be 1 because

now the \lim_{h \to 0} \frac{\sin (0 + h) - \sin (0 - h)}{2 h} = \frac{π}{180}

\Rightarrow we need a function with f (0) = \frac{π}{180}

Answered by TANMAY PANACEA. last updated on 26/Apr/20

x d e g r e e = \frac{π}{180} x r a d i a n

y = s i n (\frac{π}{180} x)

\frac{d y}{d x} = c o s (\frac{π}{180} x) \times \frac{π}{180}

Commented by M±th+et+s last updated on 26/Apr/20

t h a n k y o u s i r b u t i h a v e a q u e s t i o n

w h y s i n (\frac{π}{180} x) = s i n (x)

b u t \frac{π}{180} c o s (\frac{π}{180} x) = c o s (x)

b e c a u s e w e k n o w \frac{d}{d x} s i n (x) = c o s (x)

Answered by M±th+et+s last updated on 27/Apr/20

y = s i n (x) y = s i n (\frac{π}{180} x)

\frac{π}{180} x = u y = s i n u

\frac{d y}{d x} = c o s (u) \frac{d y}{d x} = c o s (\frac{π}{180} x)

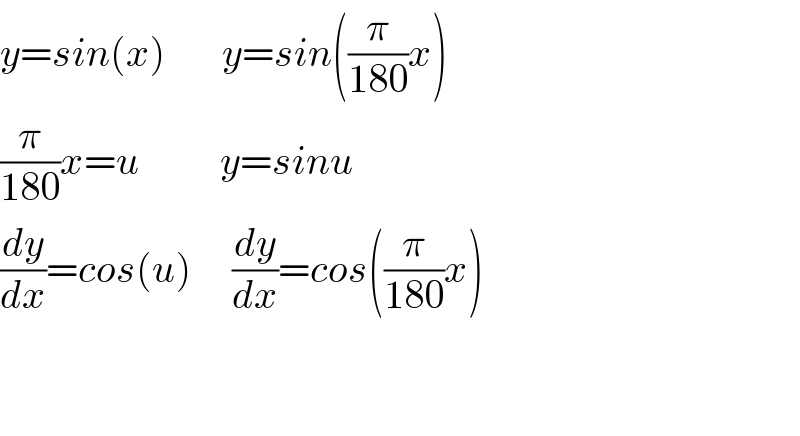
Commented by M±th+et+s last updated on 27/Apr/20

\frac{d y}{d x} s i n (x) = c o s (x)

x r a d = \frac{π}{180} x d e g

c o s (x) = c o s (\frac{π}{180} x)

s o \frac{d y}{d x} s i n (\frac{π}{180} x) = c o s (\frac{π}{180} x)