Menu Close

If-three-positive-numbers-a-b-c-are-in-A-P-and-1-a-2-1-b-2-1-c-2-also-in-A-P-then-1-a-b-c-2-2b-3a-c-3-b-2-ac-8-4-2c-2b-a-

Tinku Tara 0 Comments
Pin




Question Number 24858 by Tinkutara last updated on 27/Nov/17
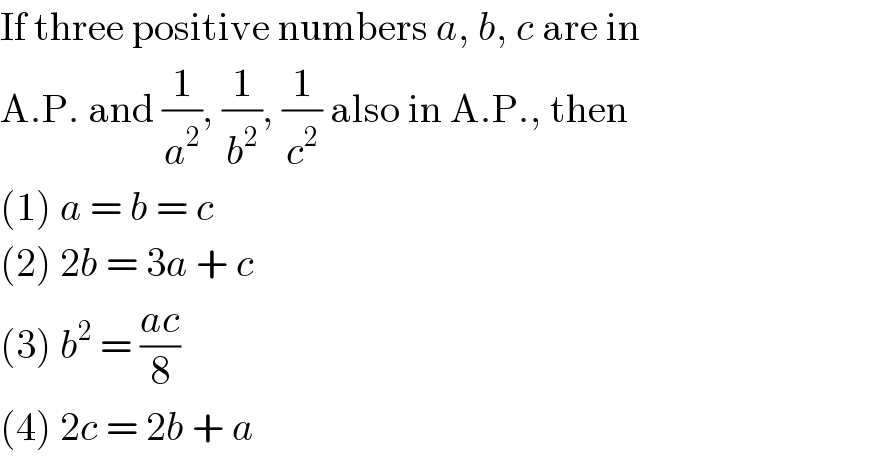
If three positive numbers a, b, c are in A.P. and (1/a^2 ), (1/b^2 ), (1/c^2 ) also in A.P., then (1) a = b = c (2) 2b = 3a + c (3) b^2 = ((ac)/8) (4) 2c = 2b + a
$$\mathrm{If}\:\mathrm{three}\:\mathrm{positive}\:\mathrm{numbers}\:{a},\:{b},\:{c}\:\mathrm{are}\:\mathrm{in} \\ $$$$\mathrm{A}.\mathrm{P}.\:\mathrm{and}\:\frac{\mathrm{1}}{{a}^{\mathrm{2}} },\:\frac{\mathrm{1}}{{b}^{\mathrm{2}} },\:\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\:\mathrm{also}\:\mathrm{in}\:\mathrm{A}.\mathrm{P}.,\:\mathrm{then} \\ $$$$\left(\mathrm{1}\right)\:{a}\:=\:{b}\:=\:{c} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{2}{b}\:=\:\mathrm{3}{a}\:+\:{c} \\ $$$$\left(\mathrm{3}\right)\:{b}^{\mathrm{2}} \:=\:\frac{{ac}}{\mathrm{8}} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{2}{c}\:=\:\mathrm{2}{b}\:+\:{a} \\ $$
Answered by ajfour last updated on 28/Nov/17
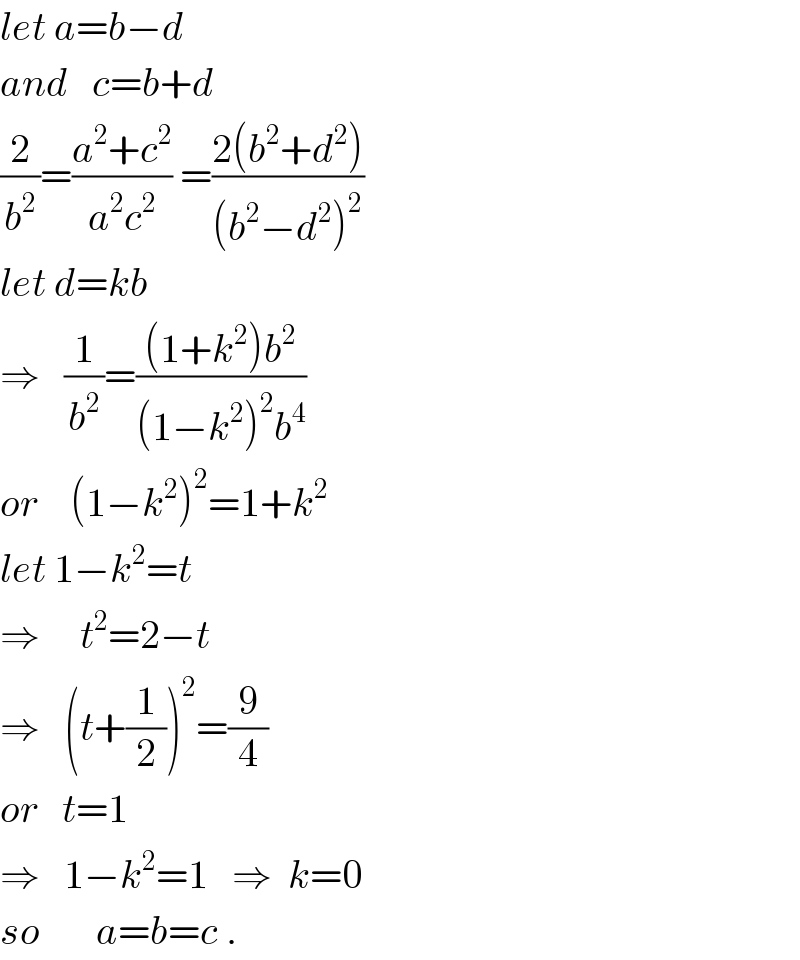
let a=b−d and c=b+d (2/b^2 )=((a^2 +c^2 )/(a^2 c^2 )) =((2(b^2 +d^2 ))/((b^2 −d^2 )^2 )) let d=kb ⇒ (1/b^2 )=(((1+k^2 )b^2 )/((1−k^2 )^2 b^4 )) or (1−k^2 )^2 =1+k^2 let 1−k^2 =t ⇒ t^2 =2−t ⇒ (t+(1/2))^2 =(9/4) or t=1 ⇒ 1−k^2 =1 ⇒ k=0 so a=b=c .
$${let}\:{a}={b}−{d} \\ $$$${and}\:\:\:{c}={b}+{d} \\ $$$$\frac{\mathrm{2}}{{b}^{\mathrm{2}} }=\frac{{a}^{\mathrm{2}} +{c}^{\mathrm{2}} }{{a}^{\mathrm{2}} {c}^{\mathrm{2}} }\:=\frac{\mathrm{2}\left({b}^{\mathrm{2}} +{d}^{\mathrm{2}} \right)}{\left({b}^{\mathrm{2}} −{d}^{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$$${let}\:{d}={kb} \\ $$$$\Rightarrow\:\:\:\frac{\mathrm{1}}{{b}^{\mathrm{2}} }=\frac{\left(\mathrm{1}+{k}^{\mathrm{2}} \right){b}^{\mathrm{2}} }{\left(\mathrm{1}−{k}^{\mathrm{2}} \right)^{\mathrm{2}} {b}^{\mathrm{4}} } \\ $$$${or}\:\:\:\:\left(\mathrm{1}−{k}^{\mathrm{2}} \right)^{\mathrm{2}} =\mathrm{1}+{k}^{\mathrm{2}} \\ $$$${let}\:\mathrm{1}−{k}^{\mathrm{2}} ={t} \\ $$$$\Rightarrow\:\:\:\:\:{t}^{\mathrm{2}} =\mathrm{2}−{t} \\ $$$$\Rightarrow\:\:\:\left({t}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} =\frac{\mathrm{9}}{\mathrm{4}} \\ $$$${or}\:\:\:{t}=\mathrm{1} \\ $$$$\Rightarrow\:\:\:\mathrm{1}−{k}^{\mathrm{2}} =\mathrm{1}\:\:\:\Rightarrow\:\:{k}=\mathrm{0} \\ $$$${so}\:\:\:\:\:\:\:{a}={b}={c}\:. \\ $$
Commented by prakash jain last updated on 30/Nov/17
t=−2 k=(√3) b=b a=b(1−(√3)) c=b(1+(√3)) That is the solution provided by jota+.
$${t}=−\mathrm{2} \\ $$$${k}=\sqrt{\mathrm{3}} \\ $$$${b}={b} \\ $$$${a}={b}\left(\mathrm{1}−\sqrt{\mathrm{3}}\right) \\ $$$${c}={b}\left(\mathrm{1}+\sqrt{\mathrm{3}}\right) \\ $$$$\mathrm{That}\:\mathrm{is}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{provided} \\ $$$$\mathrm{by}\:\mathrm{jota}+. \\ $$
Commented by Tinkutara last updated on 28/Nov/17
Thank you Sir!
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{Sir}! \\ $$
Commented by prakash jain last updated on 30/Nov/17
What about the solution posted below by jota+. I have put my calculation as a comment there.
$$\mathrm{What}\:\mathrm{about}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{posted} \\ $$$$\mathrm{below}\:\mathrm{by}\:\mathrm{jota}+.\:\mathrm{I}\:\mathrm{have}\:\mathrm{put} \\ $$$$\mathrm{my}\:\mathrm{calculation}\:\mathrm{as}\:\mathrm{a}\:\mathrm{comment}\:\mathrm{there}. \\ $$
Answered by jota+ last updated on 29/Nov/17
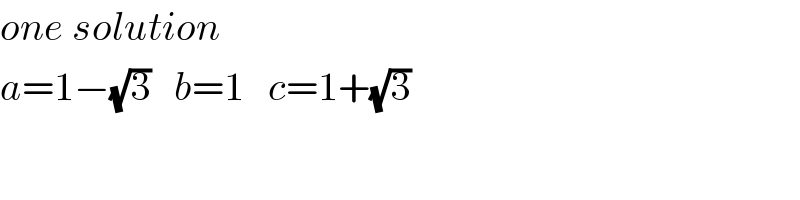
one solution a=1−(√3) b=1 c=1+(√3)
$${one}\:{solution} \\ $$$${a}=\mathrm{1}−\sqrt{\mathrm{3}}\:\:\:{b}=\mathrm{1}\:\:\:{c}=\mathrm{1}+\sqrt{\mathrm{3}} \\ $$
Commented by prakash jain last updated on 30/Nov/17
ok.
$${ok}. \\ $$
Commented by prakash jain last updated on 30/Nov/17
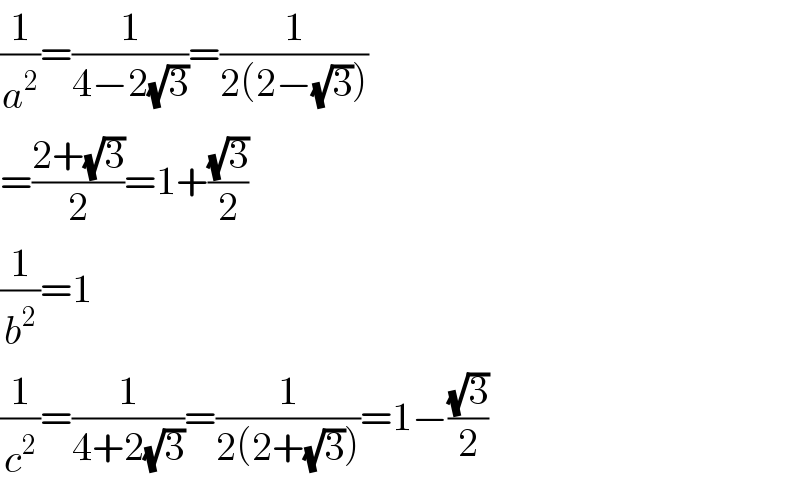
(1/a^2 )=(1/(4−2(√3)))=(1/(2(2−(√3)))) =((2+(√3))/2)=1+((√3)/2) (1/b^2 )=1 (1/c^2 )=(1/(4+2(√3)))=(1/(2(2+(√3))))=1−((√3)/2)
$$\frac{\mathrm{1}}{{a}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{4}−\mathrm{2}\sqrt{\mathrm{3}}}=\frac{\mathrm{1}}{\mathrm{2}\left(\mathrm{2}−\sqrt{\mathrm{3}}\right)} \\ $$$$=\frac{\mathrm{2}+\sqrt{\mathrm{3}}}{\mathrm{2}}=\mathrm{1}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\frac{\mathrm{1}}{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\frac{\mathrm{1}}{{c}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{3}}}=\frac{\mathrm{1}}{\mathrm{2}\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)}=\mathrm{1}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$
Commented by Tinkutara last updated on 30/Nov/17
But my ques. says a,b,c to be +ve.
$$\mathrm{But}\:\mathrm{my}\:\mathrm{ques}.\:\mathrm{says}\:{a},{b},{c}\:\mathrm{to}\:\mathrm{be}\:+\mathrm{ve}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *