If-x-1-x-2-x-0-prove-that-x-n-1-x-n-2-n-Z-

Question Number 108516 by Rasheed.Sindhi last updated on 17/Aug/20

If x + \frac{1}{x} = 2 (x \neq 0), prove that

x^{n} + \frac{1}{x^{n}} = 2 \forall n \in Z

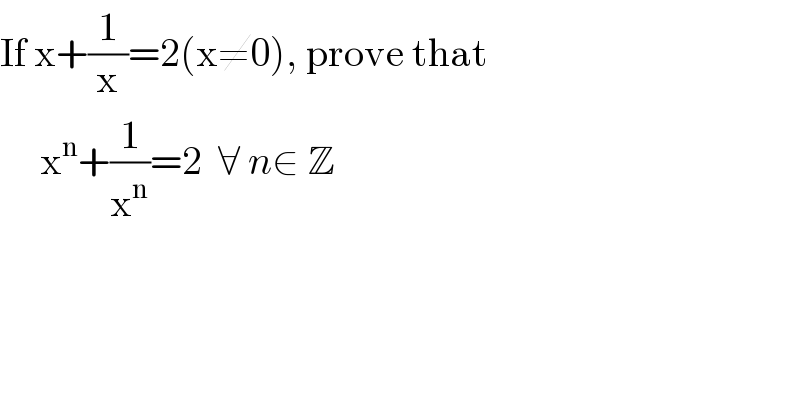
Commented by mr W last updated on 17/Aug/20

i f x > 0 :

x + \frac{1}{x} ⩾ 2 \sqrt{x \times \frac{1}{x}} = 2

= o n l y f o r x = \frac{1}{x} = 1

i f x < 0 :

x + \frac{1}{x} = - (- x + \frac{1}{- x}) ⩽ - 2

\Rightarrow n o s o l u t i o n f o r x + \frac{1}{x} = 2

\Rightarrow o n l y s o l u t i o n f o r x + \frac{1}{x} = 2 i s

x = 1

\Rightarrow x^{n} + \frac{1}{x^{n}} = 1 + 1 = 2

Commented by Rasheed.Sindhi last updated on 17/Aug/20

e^{x} cellent!

Commented by udaythool last updated on 17/Aug/20

By induction it follows \dots

Commented by Rasheed.Sindhi last updated on 17/Aug/20

T h a n k s s i r w a i t i n g f o r p r o o f b y

i n d u c t i o n \dots .

Commented by udaythool last updated on 17/Aug/20

Let n = 0, 1, \dots

Induction statment :

P_{n} : x^{n} + \frac{1}{x^{n}} = 2

For n = 0 and 1, P_{n} is true

Induction hypothesis :

Let P_{n} is true for all n = 0, \dots, k

∴ (x^{k + 1} + \frac{1}{x^{k + 1}}) = (x + \frac{1}{x}) (x^{k} + \frac{1}{x^{k}}) - 2 = 2 \times 2 - 2 = 2

i . e . P_{k} \Rightarrow P_{k + 1}

Thus by PI P_{n} is true for all n \in Z^{+}

If n \in Z^{-} \Rightarrow n = - m for m =∣ n ∣\in Z^{+}

∴ x^{n} + \frac{1}{x^{n}} = x^{- m} + \frac{1}{x^{- m}} = x^{m} + \frac{1}{x^{m}} = 2

And hence P_{n} is true \forall n \in Z

etc \dots

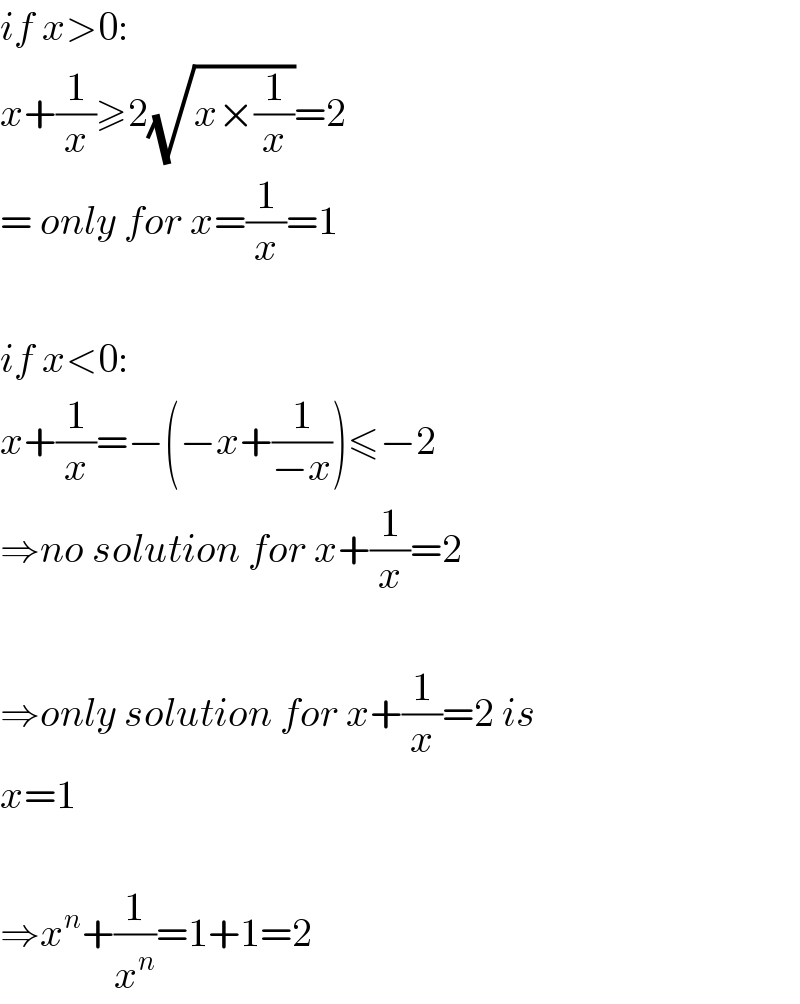
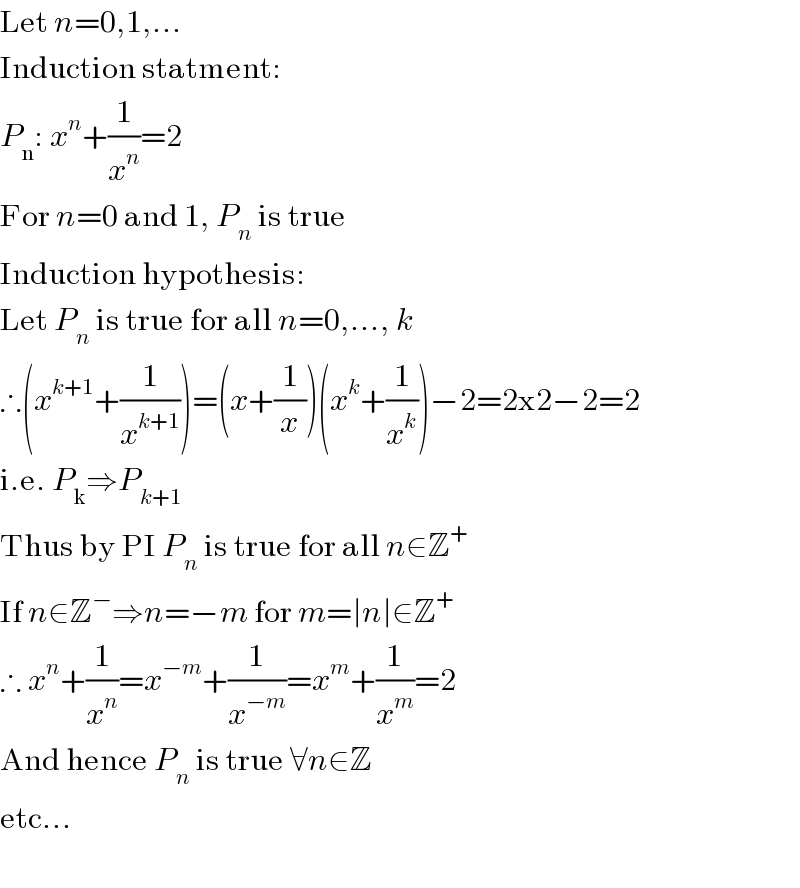
Commented by Rasheed.Sindhi last updated on 18/Aug/20

N i c e s i r!

T h e l i n e b e l o w n e e d s s o m e

d e t a i l : T h a n k s .

∴ (x^{k + 1} + \frac{1}{x^{k + 1}}) = (x + \frac{1}{x}) (x^{k} + \frac{1}{x^{k}}) - 2 = 2 \times 2 - 2 = 2

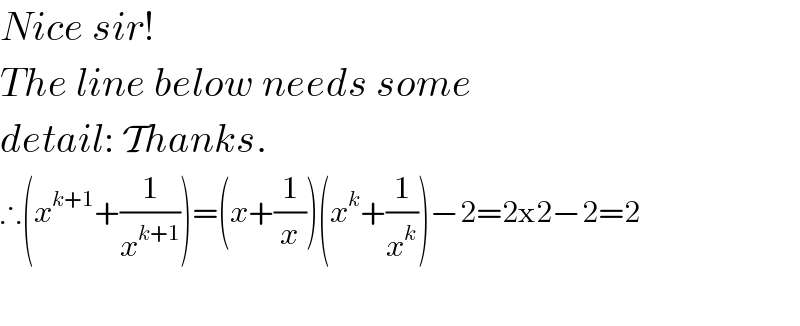
Commented by udaythool last updated on 13/Sep/20

Actually;

(x + \frac{1}{x}) (x^{k} + \frac{1}{x^{k}}) = (x^{k + 1} + \frac{1}{x^{k + 1}}) + (x^{k - 1} + \frac{1}{x^{k - 1}})

\Rightarrow (x^{k + 1} + \frac{1}{x^{k + 1}}) = (x + \frac{1}{x}) (x^{k} + \frac{1}{x^{k}}) - (x^{k - 1} + \frac{1}{x^{k - 1}})

we have assumed (induction

hypothesis) p_{n} is true for n = k

i . e . p_{n} = 2 for n = 1, 2, \dots, k

Thus (x^{k + 1} + \frac{1}{x^{k + 1}}) = 2 \times 2 - 2 = 2

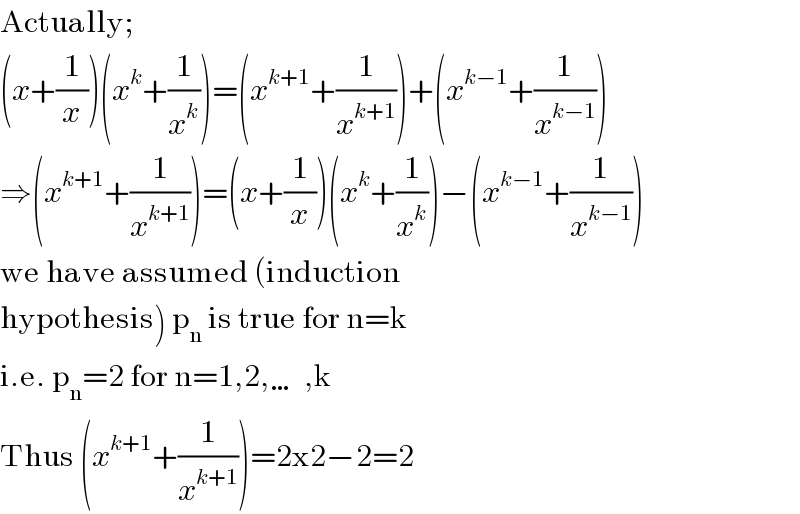
Answered by floor(10²Eta[1]) last updated on 17/Aug/20

x^{2} - 2 x + 1 = {(x - 1)}^{2} = 0 \Rightarrow x = 1

\Rightarrow x^{n} = 1 ∴ x^{n} + \frac{1}{x^{n}} = 2 = 1 + \frac{1}{1}

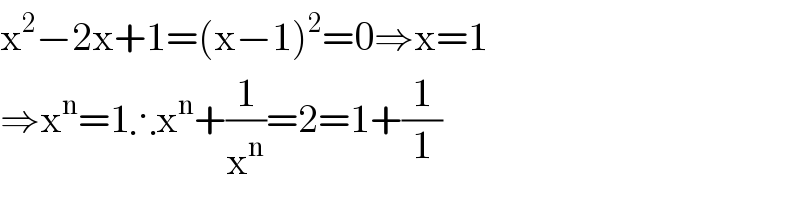
Commented by Rasheed.Sindhi last updated on 17/Aug/20

θ α n X s i r!

Answered by mathmax by abdo last updated on 17/Aug/20

x + \frac{1}{x} = 2 \Rightarrow x^{2} + 1 = 2 x \Rightarrow x^{2} - 2 x + 1 = 0 \Rightarrow {(x - 1)}^{2} = 0 \Rightarrow x = 1 \Rightarrow

\forall n we hsve x^{n} + \frac{1}{x^{n}} = 2

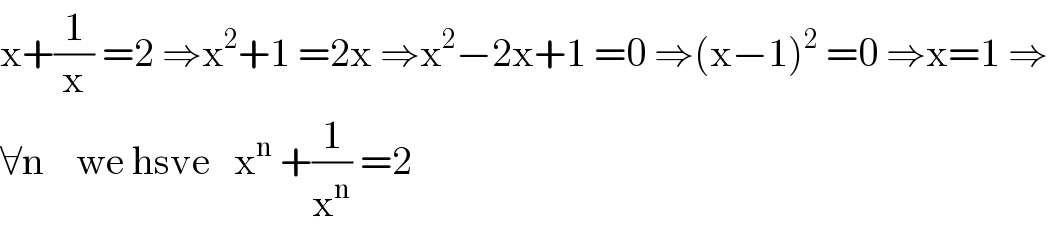
Commented by Rasheed.Sindhi last updated on 18/Aug/20

T h a n x s i r