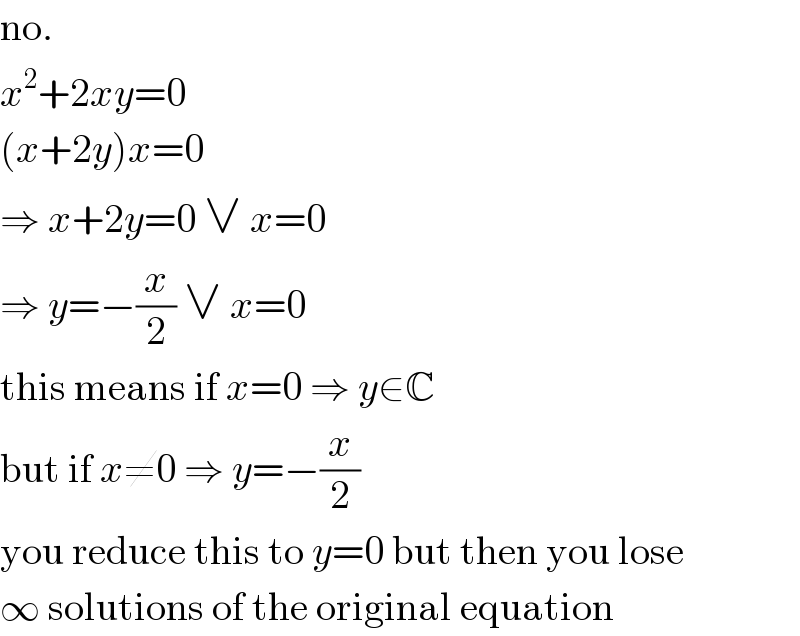If-x-2-2xy-0-find-y-

Question Number 92426 by Jidda28 last updated on 06/May/20

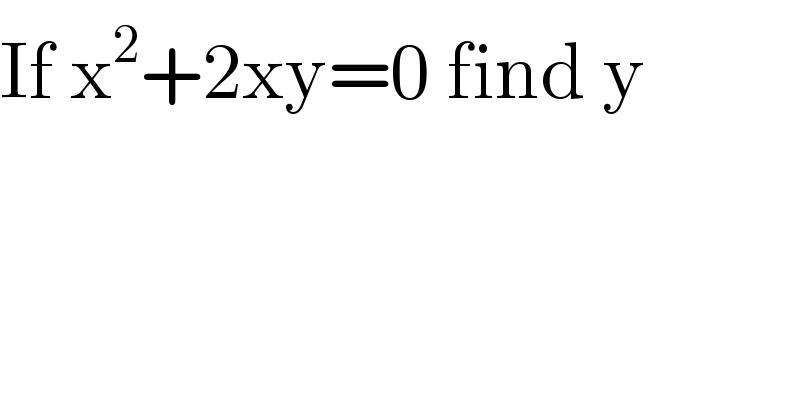
If x^{2} + 2 xy = 0 find y

Commented by Jidda28 last updated on 07/May/20

thank you so much sir

Commented by Rio Michael last updated on 06/May/20

sir please reduce your font sizes alittle

solution

x^{2} + 2 x y = 0

\Rightarrow x (x + 2 y) = 0

either x = 0 or x + 2 y = 0

y = - \frac{x}{2}

\Rightarrow y = 0

Commented by Jidda28 last updated on 06/May/20

ok sir thank for the solution

Commented by MJS last updated on 07/May/20

no .

x^{2} + 2 x y = 0

(x + 2 y) x = 0

\Rightarrow x + 2 y = 0 \lor x = 0

\Rightarrow y = - \frac{x}{2} \lor x = 0

this means if x = 0 \Rightarrow y \in C

but if x \neq 0 \Rightarrow y = - \frac{x}{2}

you reduce this to y = 0 but then you lose

\infty solutions of the original equation

Facebook Tweet Pin