If-x-y-Z-23-2-x-5-y-k-and-k-is-an-even-number-then-what-is-max-x-y-

Question Number 176847 by Ar Brandon last updated on 27/Sep/22

If x, y \in Z

23! = 2^{x} 5^{y} k

and k is an even number, then what is

\max (x + y) ?

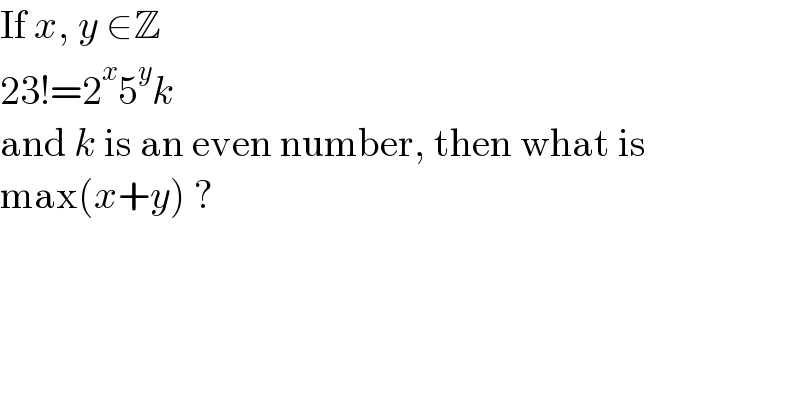
Answered by BaliramKumar last updated on 27/Sep/22

\begin{array}{cccccc} 2 & 23 \\ 2 & 11 \\ 2 & 5 \\ 2 & 2 \\ 2 & 1 \\ 0 \end{array} & \begin{array}{ccc} 5 & 23 \\ 5 & 4 \\ 0 \end{array} \begin{array}{cc}  \end{array}

x = 11 + 5 + 2 + 1 + 0 = 19

y = 4 + 0 = 4

23! = 2^{19} \times 5^{4} \times x [w h e r e^{'} x^{'} w i l l b e o d d]

23! = 2^{18} \times 5^{4} \times (2 x)

23! = 2^{18} \times 5^{4} \times k [2 x = k]

m a x (x + y) = 18 + 4 = \begin{matrix} 22 \end{matrix}

Commented by Ar Brandon last updated on 28/Sep/22

Thanks

Answered by mr W last updated on 27/Sep/22

⌊ \frac{23}{2} ⌋ + ⌊ \frac{23}{2^{2}} ⌋ + ⌊ \frac{23}{2^{3}} ⌋ + ⌊ \frac{23}{2^{4}} ⌋ = 11 + 5 + 2 + 1 = 19

⌊ \frac{23}{5} ⌋ = 4

\Rightarrow 23! = 2^{19} \times 5^{4} \times \dots

s u c h t h a t (\dots) i s e v e n, i t m u s t c o n t a i n

a t l e a s t o n e f a c t o r 2 .

\Rightarrow x_{m a x} = 18, y_{m a x} = 4

\Rightarrow {(x + y)}_{m a x} = 18 + 4 = 22

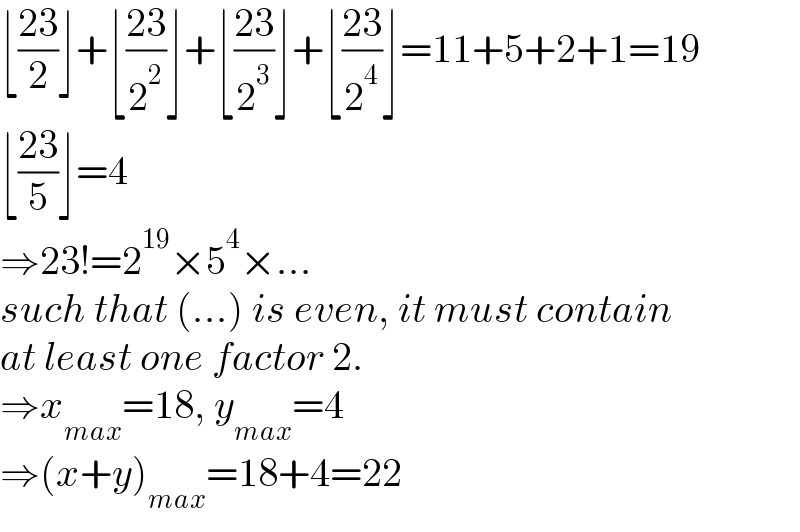
Commented by Ar Brandon last updated on 27/Sep/22

Thank you ��

Answered by Rasheed.Sindhi last updated on 27/Sep/22

2 4 6 8 10 12 14 16 18 20 22 \dots \dots 11

4 8 12 16 20 \dots \dots \dots . 5

8 16 \dots \dots \dots \dots \dots \dots 2

16 \dots \dots \dots \dots \dots \dots \dots 1

11 + 5 + 2 + 1 = 19 2^{'} s

k i e v e n m a x i m u m x i s 18

5 10 15 20 \dots \dots \dots . . 4

m a x i m u m y 4

m a x (x + y) = 18 + 4 = 22

Commented by Ar Brandon last updated on 27/Sep/22

Thanks ��