Question Number 16893 by chux last updated on 27/Jun/17
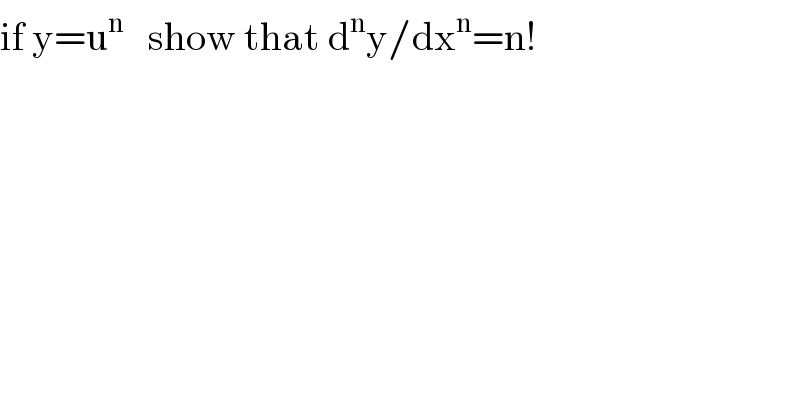
$$\mathrm{if}\:\mathrm{y}=\mathrm{u}^{\mathrm{n}} \:\:\:\mathrm{show}\:\mathrm{that}\:\mathrm{d}^{\mathrm{n}} \mathrm{y}/\mathrm{dx}^{\mathrm{n}} =\mathrm{n}! \\ $$
Commented by mrW1 last updated on 28/Jun/17

$$\mathrm{if}\:\mathrm{y}=\mathrm{u}^{\mathrm{n}} \:\:\:\mathrm{then}\:\mathrm{d}^{\mathrm{n}} \mathrm{y}/\mathrm{dx}^{\mathrm{n}} =\mathrm{0} \\ $$$$\mathrm{if}\:\mathrm{y}=\mathrm{x}^{\mathrm{n}} \:\:\:\mathrm{then}\:\mathrm{d}^{\mathrm{n}} \mathrm{y}/\mathrm{dx}^{\mathrm{n}} =\mathrm{n}! \\ $$
Commented by chux last updated on 28/Jun/17

$$\mathrm{please}\:\mathrm{i}\:\mathrm{dont}\:\mathrm{really}\:\mathrm{understand}\:\mathrm{it} \\ $$
Commented by prakash jain last updated on 28/Jun/17
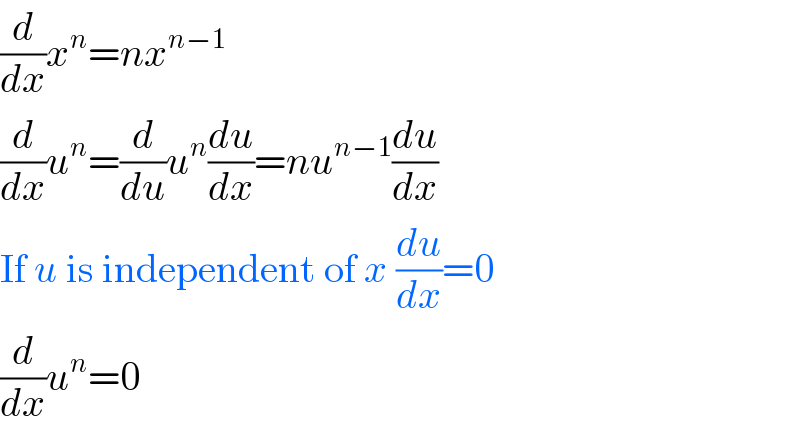
$$\frac{{d}}{{dx}}{x}^{{n}} ={nx}^{{n}−\mathrm{1}} \\ $$$$\frac{{d}}{{dx}}{u}^{{n}} =\frac{{d}}{{du}}{u}^{{n}} \frac{{du}}{{dx}}={nu}^{{n}−\mathrm{1}} \frac{{du}}{{dx}} \\ $$$$\mathrm{If}\:{u}\:\mathrm{is}\:\mathrm{independent}\:\mathrm{of}\:{x}\:\frac{{du}}{{dx}}=\mathrm{0} \\ $$$$\frac{{d}}{{dx}}{u}^{{n}} =\mathrm{0} \\ $$
Commented by chux last updated on 28/Jun/17

$$\mathrm{thanks}…..\:\mathrm{i}\:\mathrm{get}\:\mathrm{you}\:\mathrm{now}. \\ $$
