Question Number 16876 by tawa tawa last updated on 27/Jun/17

$$\mathrm{In}\:\mathrm{how}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{a}\:\mathrm{family}\:\mathrm{of}\:\mathrm{5}\:\mathrm{brothers}\:\mathrm{be}\:\mathrm{seated}\:\mathrm{round}\:\mathrm{a}\:\mathrm{table} \\ $$$$\mathrm{if}\:\left(\mathrm{i}\right)\:\mathrm{2}\:\mathrm{brothers}\:\mathrm{must}\:\mathrm{seat}\:\mathrm{next}\:\mathrm{to}\:\mathrm{each}\:\mathrm{other}. \\ $$$$\left(\mathrm{ii}\right)\:\mathrm{2}\:\mathrm{brothers}\:\mathrm{must}\:\mathrm{not}\:\mathrm{seat}\:\mathrm{together}. \\ $$
Answered by mrW1 last updated on 27/Jun/17
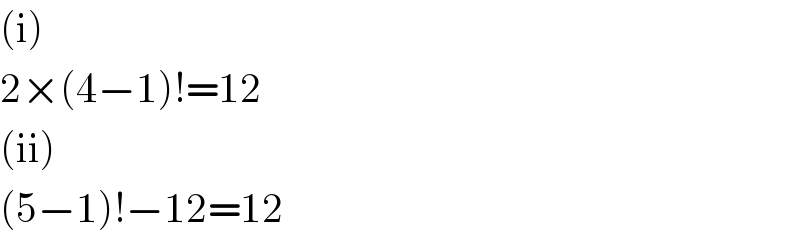
$$\left(\mathrm{i}\right) \\ $$$$\mathrm{2}×\left(\mathrm{4}−\mathrm{1}\right)!=\mathrm{12} \\ $$$$\left(\mathrm{ii}\right) \\ $$$$\left(\mathrm{5}−\mathrm{1}\right)!−\mathrm{12}=\mathrm{12} \\ $$
Commented by tawa tawa last updated on 27/Jun/17

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
