In-Matrices-Is-A-1-B-B-A-1-

Question Number 41332 by rahul 19 last updated on 05/Aug/18

In Matrices,

Is (A^{- 1}) B = B (A^{- 1}) ?

Commented by rahul 19 last updated on 06/Aug/18

ok prof .

Commented by candre last updated on 06/Aug/18

| \begin{matrix} 1 \\ 2 \end{matrix} | | \begin{matrix} 1 & 2 \end{matrix} | = | \begin{matrix} 1 \\ 2 \end{matrix} |

| \begin{matrix} 1 & 2 \end{matrix} | | \begin{matrix} 1 \\ 2 \end{matrix} | = | \begin{matrix} 3 \end{matrix} |

also AB e x i s t i n g {d o n}^{'} t e v e n i m p l y B A e x i s t

so, in general A B \neq B A

B u t i t n o t n e c e s s a r y m e a n t h a t t h e r e n o v a l u e s

s u c h A B = B A, f o r s q u a r e s m a t r i z e s

I A = A I

w h e r e I i s i n d e n t y m a t r i z

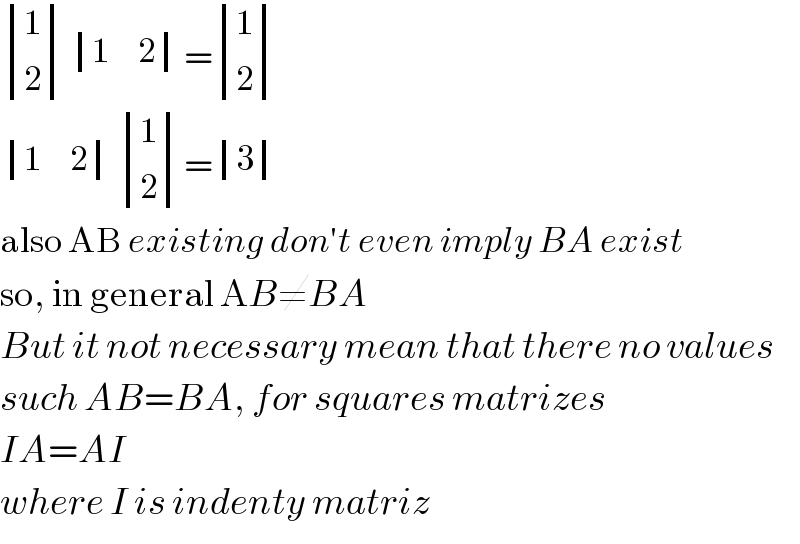
Commented by maxmathsup by imad last updated on 05/Aug/18

g e n e r a l l y t w o m a t r i c e d o n t c o m m u t e m e a n s A . B \neq B . A s o

g e n a r a l l y A^{- 1} . B \neq B . A^{- 1}

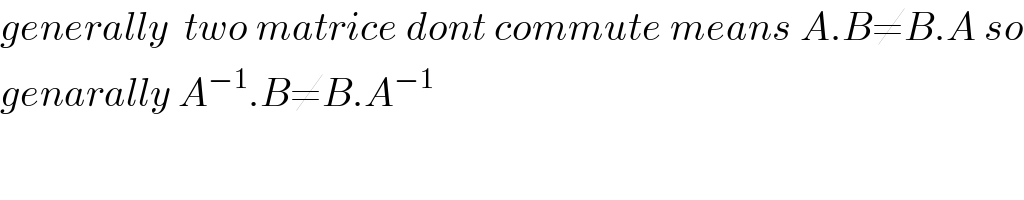
Answered by candre last updated on 05/Aug/18

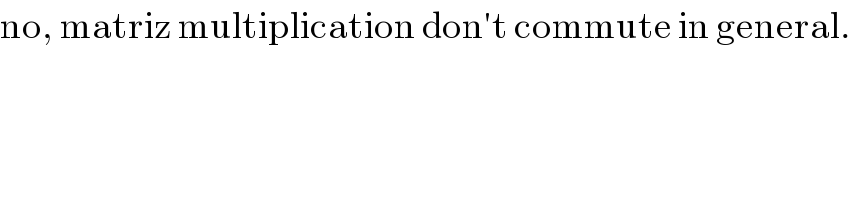
no, matriz multiplication {don}^{'} t commute in general .

Commented by rahul 19 last updated on 05/Aug/18

ok sir .