Question Number 129271 by MathSh last updated on 14/Jan/21
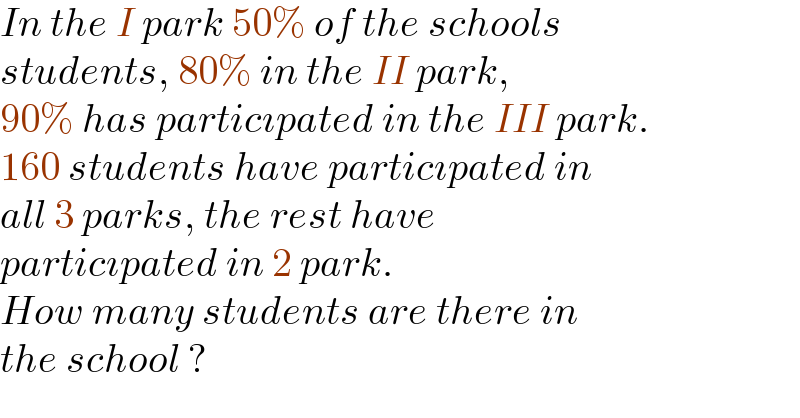
$${In}\:{the}\:{I}\:{park}\:\mathrm{50\%}\:{of}\:{the}\:{schools} \\ $$$${students},\:\mathrm{80\%}\:{in}\:{the}\:{II}\:{park}, \\ $$$$\mathrm{90\%}\:{has}\:{partic}\imath{pated}\:{in}\:{the}\:{III}\:{park}. \\ $$$$\mathrm{160}\:{students}\:{have}\:{partic}\imath{pated}\:{in} \\ $$$${all}\:\mathrm{3}\:{parks},\:{the}\:{rest}\:{have} \\ $$$${partic}\imath{pated}\:{in}\:\mathrm{2}\:{park}. \\ $$$${How}\:{many}\:{students}\:{are}\:{there}\:{in} \\ $$$${the}\:{school}\:?\: \\ $$
Commented by prakash jain last updated on 14/Jan/21

$$\mathrm{I}\:\mathrm{get}\:−\mathrm{ve}\:\mathrm{answer} \\ $$$$\mathrm{set}\:\mathrm{A}=\mathrm{student}\:\mathrm{in}\:\mathrm{I}\:\mathrm{park} \\ $$$$\mathrm{set}\:\mathrm{B}=\mathrm{student}\:\mathrm{in}\:\mathrm{II}\:\mathrm{park} \\ $$$$\mathrm{set}\:\mathrm{C}=\mathrm{student}\:\mathrm{in}\:\mathrm{III}\:\mathrm{park} \\ $$$$\mathrm{Total}\:\mathrm{student}\:{x}={n}\left(\mathrm{A}\cup\mathrm{B}\cup\mathrm{C}\right) \\ $$$${n}\left({A}\cup\mathrm{B}\cup\mathrm{C}\right)={n}\left({A}\right)+{n}\left({B}\right)+{n}\left({C}\right) \\ $$$$\:\:\:−{n}\left({A}\cup{B}\right)−{n}\left({A}\cup{C}\right)−{n}\left({B}\cup{C}\right) \\ $$$$\:\:\:\:+{n}\left({A}\cup{B}\cup{C}\right) \\ $$$${x}−\mathrm{160}=\mathrm{student}\:\mathrm{in}\:\mathrm{2}\:\mathrm{parks} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:={n}\left({A}\cup{B}\right)+{n}\left({A}\cup{C}\right)+{n}\left({B}\cup{C}\right) \\ $$$${x}=.\mathrm{5}{x}+.\mathrm{8}{x}+.\mathrm{9}{x}−\left({x}−\mathrm{160}\right)+\mathrm{160} \\ $$$${x}=\mathrm{2}.\mathrm{2}{x}−{x}+\mathrm{320} \\ $$$$−.\mathrm{2}{x}=\mathrm{320} \\ $$$${x}=−\mathrm{1600} \\ $$
Commented by MathSh last updated on 14/Jan/21

$${Thanks}\:{Sir},\:{ansver}\:{student}\:“−''? \\ $$
Commented by MathSh last updated on 14/Jan/21

$${Perfect}\:{Thanks}\:{Sir} \\ $$
Commented by MathSh last updated on 14/Jan/21

$${Ansver}:\:\mathrm{640}\:{Sir}? \\ $$
Commented by prakash jain last updated on 14/Jan/21

$$\mathrm{sorry}\:\mathrm{mistake}. \\ $$$$\mathrm{number}\:\mathrm{of}\:\mathrm{students}\:{x}−\mathrm{160} \\ $$$$\mathrm{is}\:\mathrm{number}\:\mathrm{of}\:\mathrm{student}\:\mathrm{who} \\ $$$$\mathrm{attended}\:\mathrm{exactly}\:\mathrm{2}\:\mathrm{parks} \\ $$$${x}−\mathrm{160}={n}\left({A}\cup{B}\right)−{n}\left({A}\cap{B}\cap{C}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+{n}\left({B}\cup{C}\right)−{n}\left({A}\cap{B}\cap{C}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+{n}\left({C}\cup{A}\right)−{n}\left({A}\cap{B}\cap{C}\right) \\ $$$$\:{n}\left({A}\cup{B}\right)+{n}\left({A}\cup{C}\right)+{n}\left({B}\cup{C}\right) \\ $$$$\:\:\:\:\:\:=\:{x}−\mathrm{160}+\mathrm{3}×\mathrm{160} \\ $$$$\:\:\:\:\:\:={x}+\mathrm{320} \\ $$$$−−−−−−−−−−−−− \\ $$$$\mathrm{substituting} \\ $$$${x}=\mathrm{2}.\mathrm{2}{x}−\left({x}+\mathrm{320}\right)+\mathrm{160} \\ $$$$\mathrm{160}=.\mathrm{2}{x}\Rightarrow{x}=\mathrm{800} \\ $$
Commented by prakash jain last updated on 15/Jan/21

$$\mathrm{800}.\:\mathrm{See}\:\mathrm{very}\:\mathrm{good}\:\mathrm{explaination} \\ $$$$\mathrm{from}\:\mathrm{ajfour}\:\mathrm{sir} \\ $$
