Question Number 127383 by Lordose last updated on 29/Dec/20
$$\mathrm{Is}\:\mathrm{there}\:\mathrm{any}\:\mathrm{analytic}\:\mathrm{proof}\:\mathrm{of}\:\mathrm{the}\:\mathrm{result} \\ $$$$\mathrm{sin}\left(\mathrm{x}+\mathrm{y}\right)\:=\:\mathrm{sin}\left(\mathrm{x}\right)\mathrm{cos}\left(\mathrm{y}\right)\:+\:\mathrm{sin}\left(\mathrm{y}\right)\mathrm{cos}\left(\mathrm{x}\right) \\ $$
Answered by bemath last updated on 29/Dec/20
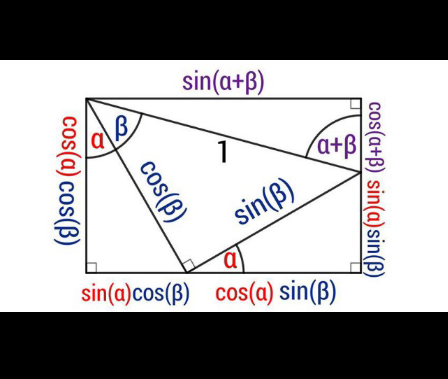
Answered by liberty last updated on 29/Dec/20
$$\mathrm{sin}\:\alpha\:=\:\mathrm{sin}\:\angle\:{ACB}\:=\:\frac{{AB}}{{AC}}\:=\:\frac{{AB}}{{AE}+{EC}}=\frac{\mathrm{sin}\:\left(\alpha+\beta\right)}{\mathrm{cos}\:\beta+\frac{\mathrm{sin}\:\beta\:\mathrm{cos}\:\alpha}{\mathrm{sin}\:\alpha}} \\ $$$$\:{so}\:{we}\:{have}\:{the}\:{equation}\:\frac{\mathrm{sin}\:\left(\alpha+\beta\right)}{\mathrm{cos}\:\beta+\frac{\mathrm{sin}\:\beta\:\mathrm{cos}\:\alpha}{\mathrm{sin}\:\alpha}}\:=\:\mathrm{sin}\:\alpha \\ $$$${then}\:\mathrm{sin}\:\left(\alpha+\beta\right)\:=\:\mathrm{sin}\:\alpha\left(\mathrm{cos}\:\beta+\frac{\mathrm{sin}\:\beta\:\mathrm{cos}\:\alpha}{\mathrm{sin}\:\alpha}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{sin}\:\alpha\:\mathrm{cos}\:\beta\:+\:\mathrm{sin}\:\beta\:\mathrm{cos}\:\alpha\: \\ $$
Commented by liberty last updated on 29/Dec/20
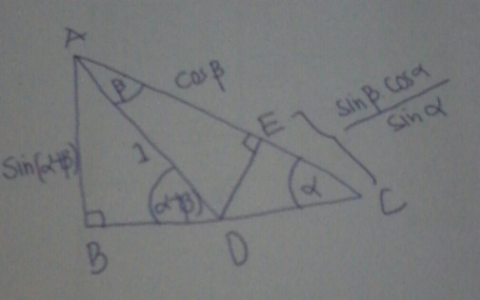
Answered by MJS_new last updated on 29/Dec/20
![sin α =((e^(iα) −e^(−iα) )/(2i))∧cos α =((e^(iα) +e^(−iα) )/2) sin (x+y) =((e^(i(x+y)) −e^(−i(x+y)) )/(2i))=((e^(ix) e^(iy) −e^(−ix) e^(−iy) )/(2i))= [let e^(ix) =u∧e^(iy) =v] =((uv−(1/(uv)))/(2i))=((2uv−(2/(uv)))/(4i))= =((2uv+(u/v)−(u/v)+(v/u)−(v/u)−(2/(uv)))/(4i))= =((uv+(u/v)−(v/u)−(1/(uv)))/(4i))+((uv−(u/v)+(v/u)−(1/(uv)))/(4i))= (((u−(1/u))(v+(1/v)))/(4i))+(((u+(1/u))(v−(1/v)))/(4i))= [u=e^(ix) ∧v=e^(iy) ] =((e^(ix) −e^(−ix) )/(2i))×((e^(iy) +e^(−iy) )/2)+((e^(ix) +e^(−ix) )/2)×((e^(iy) −e^(−iy) )/(2i))= =sin x cos y +cos x sin y](https://www.tinkutara.com/question/Q127440.png)
$$\mathrm{sin}\:\alpha\:=\frac{\mathrm{e}^{\mathrm{i}\alpha} −\mathrm{e}^{−\mathrm{i}\alpha} }{\mathrm{2i}}\wedge\mathrm{cos}\:\alpha\:=\frac{\mathrm{e}^{\mathrm{i}\alpha} +\mathrm{e}^{−\mathrm{i}\alpha} }{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{sin}\:\left({x}+{y}\right)\:=\frac{\mathrm{e}^{\mathrm{i}\left({x}+{y}\right)} −\mathrm{e}^{−\mathrm{i}\left({x}+{y}\right)} }{\mathrm{2i}}=\frac{\mathrm{e}^{\mathrm{i}{x}} \mathrm{e}^{\mathrm{i}{y}} −\mathrm{e}^{−\mathrm{i}{x}} \mathrm{e}^{−\mathrm{i}{y}} }{\mathrm{2i}}= \\ $$$$\:\:\:\:\:\left[\mathrm{let}\:\mathrm{e}^{\mathrm{i}{x}} ={u}\wedge\mathrm{e}^{\mathrm{i}{y}} ={v}\right] \\ $$$$=\frac{{uv}−\frac{\mathrm{1}}{{uv}}}{\mathrm{2i}}=\frac{\mathrm{2}{uv}−\frac{\mathrm{2}}{{uv}}}{\mathrm{4i}}= \\ $$$$=\frac{\mathrm{2}{uv}+\frac{{u}}{{v}}−\frac{{u}}{{v}}+\frac{{v}}{{u}}−\frac{{v}}{{u}}−\frac{\mathrm{2}}{{uv}}}{\mathrm{4i}}= \\ $$$$=\frac{{uv}+\frac{{u}}{{v}}−\frac{{v}}{{u}}−\frac{\mathrm{1}}{{uv}}}{\mathrm{4i}}+\frac{{uv}−\frac{{u}}{{v}}+\frac{{v}}{{u}}−\frac{\mathrm{1}}{{uv}}}{\mathrm{4i}}= \\ $$$$\frac{\left({u}−\frac{\mathrm{1}}{{u}}\right)\left({v}+\frac{\mathrm{1}}{{v}}\right)}{\mathrm{4i}}+\frac{\left({u}+\frac{\mathrm{1}}{{u}}\right)\left({v}−\frac{\mathrm{1}}{{v}}\right)}{\mathrm{4i}}= \\ $$$$\:\:\:\:\:\left[{u}=\mathrm{e}^{\mathrm{i}{x}} \wedge{v}=\mathrm{e}^{\mathrm{i}{y}} \right] \\ $$$$=\frac{\mathrm{e}^{\mathrm{i}{x}} −\mathrm{e}^{−\mathrm{i}{x}} }{\mathrm{2i}}×\frac{\mathrm{e}^{\mathrm{i}{y}} +\mathrm{e}^{−\mathrm{i}{y}} }{\mathrm{2}}+\frac{\mathrm{e}^{\mathrm{i}{x}} +\mathrm{e}^{−\mathrm{i}{x}} }{\mathrm{2}}×\frac{\mathrm{e}^{\mathrm{i}{y}} −\mathrm{e}^{−\mathrm{i}{y}} }{\mathrm{2i}}= \\ $$$$=\mathrm{sin}\:{x}\:\mathrm{cos}\:{y}\:+\mathrm{cos}\:{x}\:\mathrm{sin}\:{y} \\ $$