Question Number 161706 by mnjuly1970 last updated on 21/Dec/21
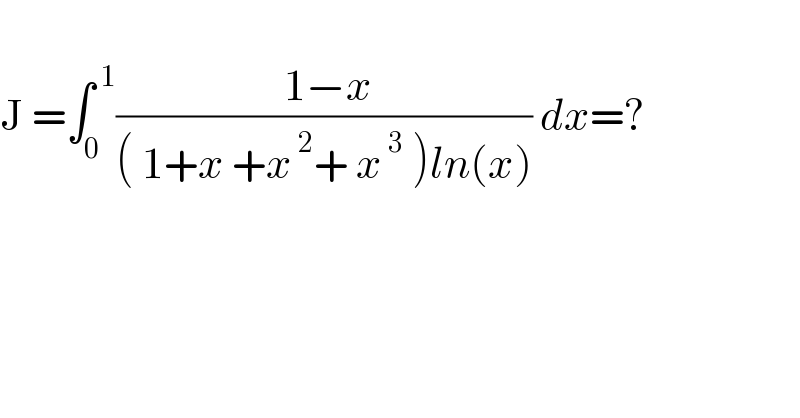
$$\: \\ $$$$\mathrm{J}\:=\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\:\mathrm{1}−{x}}{\left(\:\mathrm{1}+{x}\:+{x}^{\:\mathrm{2}} +\:{x}^{\:\mathrm{3}} \:\right){ln}\left({x}\right)}\:{dx}=? \\ $$$$ \\ $$
Answered by Ar Brandon last updated on 21/Dec/21
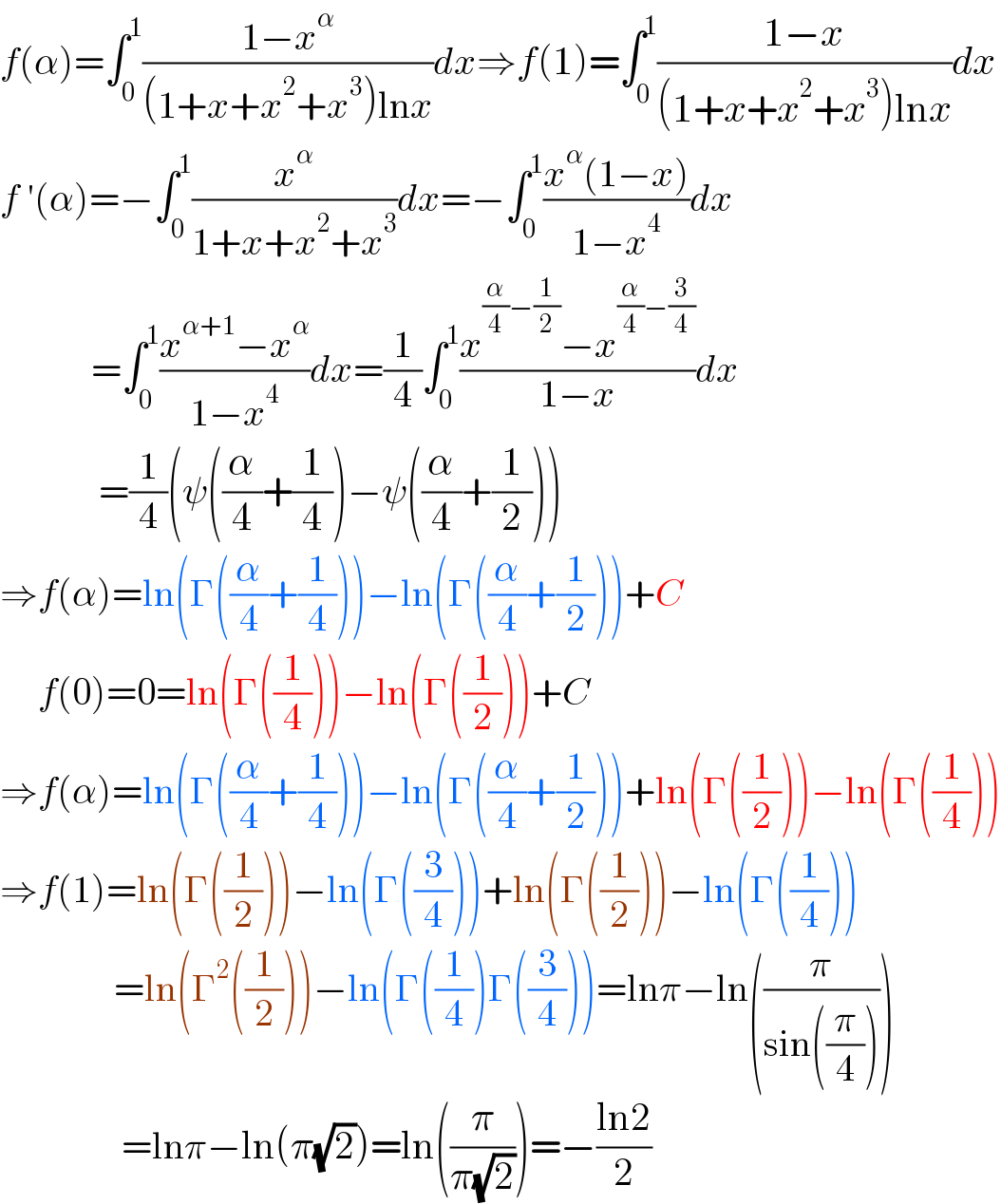
$${f}\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}−{x}^{\alpha} }{\left(\mathrm{1}+{x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} \right)\mathrm{ln}{x}}{dx}\Rightarrow{f}\left(\mathrm{1}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}−{x}}{\left(\mathrm{1}+{x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} \right)\mathrm{ln}{x}}{dx} \\ $$$${f}\:'\left(\alpha\right)=−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\alpha} }{\mathrm{1}+{x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} }{dx}=−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\alpha} \left(\mathrm{1}−{x}\right)}{\mathrm{1}−{x}^{\mathrm{4}} }{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\alpha+\mathrm{1}} −{x}^{\alpha} }{\mathrm{1}−{x}^{\mathrm{4}} }{dx}=\frac{\mathrm{1}}{\mathrm{4}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\frac{\alpha}{\mathrm{4}}−\frac{\mathrm{1}}{\mathrm{2}}} −{x}^{\frac{\alpha}{\mathrm{4}}−\frac{\mathrm{3}}{\mathrm{4}}} }{\mathrm{1}−{x}}{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{4}}\left(\psi\left(\frac{\alpha}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{4}}\right)−\psi\left(\frac{\alpha}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}\right)\right) \\ $$$$\Rightarrow{f}\left(\alpha\right)=\mathrm{ln}\left(\Gamma\left(\frac{\alpha}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{4}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\alpha}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}\right)\right)+{C} \\ $$$$\:\:\:\:\:{f}\left(\mathrm{0}\right)=\mathrm{0}=\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)+{C} \\ $$$$\Rightarrow{f}\left(\alpha\right)=\mathrm{ln}\left(\Gamma\left(\frac{\alpha}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{4}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\alpha}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}\right)\right)+\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\right) \\ $$$$\Rightarrow{f}\left(\mathrm{1}\right)=\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)\right)+\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{ln}\left(\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)\right)−\mathrm{ln}\left(\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)\right)=\mathrm{ln}\pi−\mathrm{ln}\left(\frac{\pi}{\mathrm{sin}\left(\frac{\pi}{\mathrm{4}}\right)}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{ln}\pi−\mathrm{ln}\left(\pi\sqrt{\mathrm{2}}\right)=\mathrm{ln}\left(\frac{\pi}{\pi\sqrt{\mathrm{2}}}\right)=−\frac{\mathrm{ln2}}{\mathrm{2}} \\ $$
Commented by mnjuly1970 last updated on 21/Dec/21
$$\:\:\:\:\:{very}\:{nice}\:{solution}\:{mr}\:{brandon} \\ $$$${thank}\:{you}\:{so}\:{much} \\ $$
Commented by Ar Brandon last updated on 21/Dec/21
You're welcome Sir.
