Menu Close

k-p-4-3-2-k-2-9-p-

Tinku Tara 0 Comments
Pin




Question Number 121919 by talminator2856791 last updated on 12/Nov/20
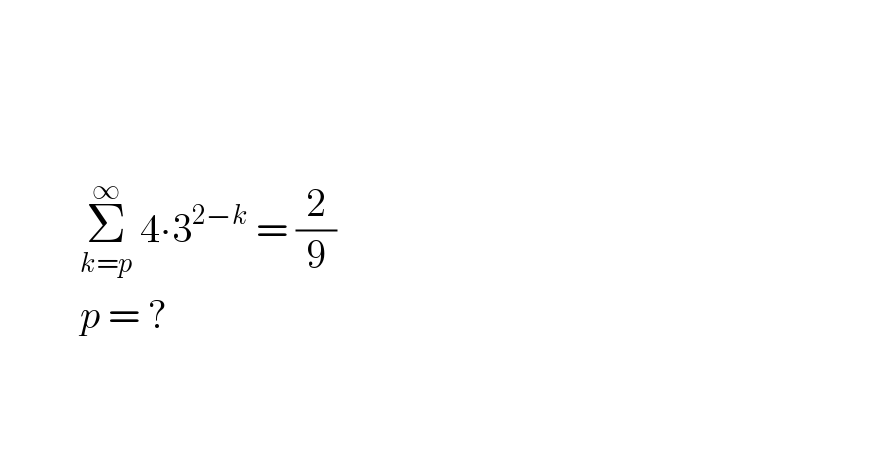
Σ_(k=p) ^∞ 4∙3^(2−k) = (2/9) p = ?
$$\: \\ $$$$\: \\ $$$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\underset{{k}={p}} {\overset{\infty} {\sum}}\:\mathrm{4}\centerdot\mathrm{3}^{\mathrm{2}−{k}} \:=\:\frac{\mathrm{2}}{\mathrm{9}} \\ $$$$\:\:\:\:\:\:\:\:\:\:{p}\:=\:? \\ $$$$\: \\ $$$$\: \\ $$
Answered by Dwaipayan Shikari last updated on 12/Nov/20
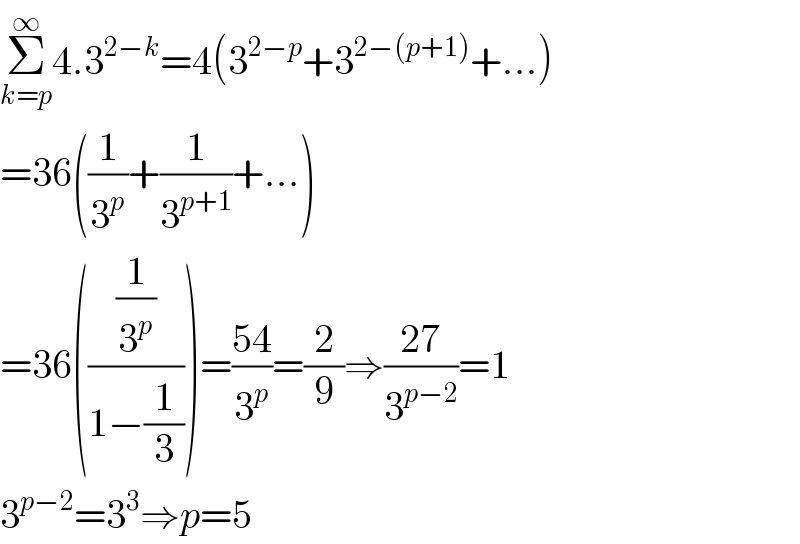
Σ_(k=p) ^∞ 4.3^(2−k) =4(3^(2−p) +3^(2−(p+1)) +...) =36((1/3^p )+(1/3^(p+1) )+...) =36(((1/3^p )/(1−(1/3))))=((54)/3^p )=(2/9)⇒((27)/3^(p−2) )=1 3^(p−2) =3^3 ⇒p=5
$$\underset{{k}={p}} {\overset{\infty} {\sum}}\mathrm{4}.\mathrm{3}^{\mathrm{2}−{k}} =\mathrm{4}\left(\mathrm{3}^{\mathrm{2}−{p}} +\mathrm{3}^{\mathrm{2}−\left({p}+\mathrm{1}\right)} +…\right) \\ $$$$=\mathrm{36}\left(\frac{\mathrm{1}}{\mathrm{3}^{{p}} }+\frac{\mathrm{1}}{\mathrm{3}^{{p}+\mathrm{1}} }+…\right) \\ $$$$=\mathrm{36}\left(\frac{\frac{\mathrm{1}}{\mathrm{3}^{{p}} }}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}}\right)=\frac{\mathrm{54}}{\mathrm{3}^{{p}} }=\frac{\mathrm{2}}{\mathrm{9}}\Rightarrow\frac{\mathrm{27}}{\mathrm{3}^{{p}−\mathrm{2}} }=\mathrm{1} \\ $$$$\mathrm{3}^{{p}−\mathrm{2}} =\mathrm{3}^{\mathrm{3}} \Rightarrow{p}=\mathrm{5} \\ $$
Answered by geoplitz last updated on 12/Nov/20
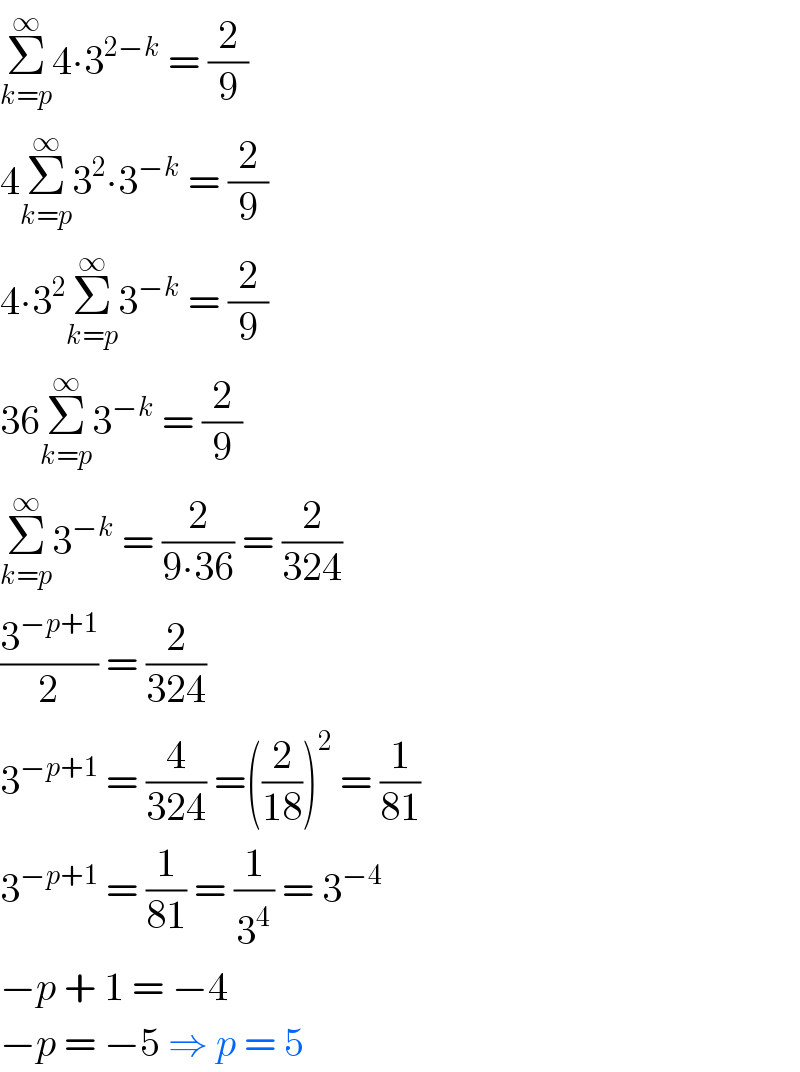
Σ_(k=p) ^∞ 4∙3^(2−k) = (2/9) 4Σ_(k=p) ^∞ 3^2 ∙3^(−k) = (2/9) 4∙3^2 Σ_(k=p) ^∞ 3^(−k) = (2/9) 36Σ_(k=p) ^∞ 3^(−k) = (2/9) Σ_(k=p) ^∞ 3^(−k) = (2/(9∙36)) = (2/(324)) (3^(−p+1) /2) = (2/(324)) 3^(−p+1) = (4/(324)) =((2/(18)))^2 = (1/(81)) 3^(−p+1) = (1/(81)) = (1/3^4 ) = 3^(−4) −p + 1 = −4 −p = −5 ⇒ p = 5
$$\underset{{k}={p}} {\overset{\infty} {\sum}}\mathrm{4}\centerdot\mathrm{3}^{\mathrm{2}−{k}} \:=\:\frac{\mathrm{2}}{\mathrm{9}} \\ $$$$\mathrm{4}\underset{{k}={p}} {\overset{\infty} {\sum}}\mathrm{3}^{\mathrm{2}} \centerdot\mathrm{3}^{−{k}} \:=\:\frac{\mathrm{2}}{\mathrm{9}} \\ $$$$\mathrm{4}\centerdot\mathrm{3}^{\mathrm{2}} \underset{{k}={p}} {\overset{\infty} {\sum}}\mathrm{3}^{−{k}} \:=\:\frac{\mathrm{2}}{\mathrm{9}} \\ $$$$\mathrm{36}\underset{{k}={p}} {\overset{\infty} {\sum}}\mathrm{3}^{−{k}} \:=\:\frac{\mathrm{2}}{\mathrm{9}} \\ $$$$\underset{{k}={p}} {\overset{\infty} {\sum}}\mathrm{3}^{−{k}} \:=\:\frac{\mathrm{2}}{\mathrm{9}\centerdot\mathrm{36}}\:=\:\frac{\mathrm{2}}{\mathrm{324}} \\ $$$$\frac{\mathrm{3}^{−{p}+\mathrm{1}} }{\mathrm{2}}\:=\:\frac{\mathrm{2}}{\mathrm{324}} \\ $$$$\mathrm{3}^{−{p}+\mathrm{1}} \:=\:\frac{\mathrm{4}}{\mathrm{324}}\:=\left(\frac{\mathrm{2}}{\mathrm{18}}\right)^{\mathrm{2}} \:=\:\frac{\mathrm{1}}{\mathrm{81}} \\ $$$$\mathrm{3}^{−{p}+\mathrm{1}} \:=\:\frac{\mathrm{1}}{\mathrm{81}}\:=\:\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{4}} }\:=\:\mathrm{3}^{−\mathrm{4}} \\ $$$$−{p}\:+\:\mathrm{1}\:=\:−\mathrm{4} \\ $$$$−{p}\:=\:−\mathrm{5}\:\Rightarrow\:{p}\:=\:\mathrm{5} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *