K-x-3-cos-x-5-4sin-x-max-K-x-min-K-x-

Question Number 163700 by blackmamba last updated on 09/Jan/22

K (x) = \frac{3 \cos x}{5 + 4 \sin x}

\begin{matrix} m a x K (x) \\ m i n K (x) \end{matrix}} = ?

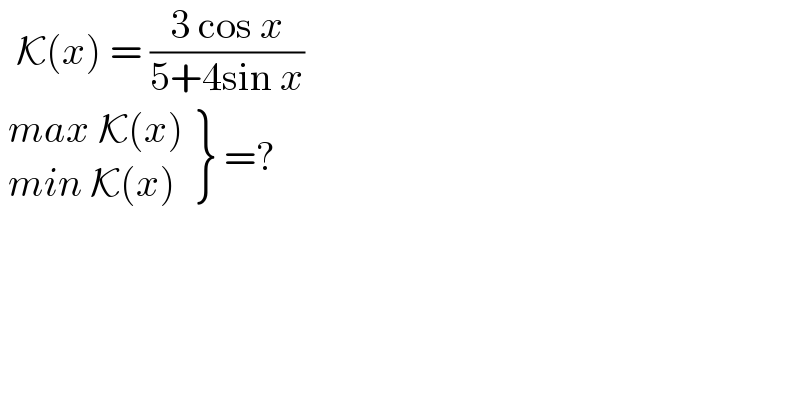
Answered by mr W last updated on 10/Jan/22

\frac{3 \cos x}{5 + 4 \sin x} = k

3 \cos x - 4 k \sin x = 5 k

\frac{3}{\sqrt{3^{2} + {(4 k)}^{2}}} \cos x - \frac{4 k}{\sqrt{3^{2} + {(4 k)}^{2}}} \sin x = \frac{5 k}{\sqrt{3^{2} + {(4 k)}^{2}}}

\cos α \cos x - \sin α \sin x = \frac{5 k}{\sqrt{3^{2} + {(4 k)}^{2}}}

\cos (x + α) = \frac{5 k}{\sqrt{3^{2} + {(4 k)}^{2}}}

\frac{5 k}{\sqrt{3^{2} + {(4 k)}^{2}}} ⩽ 1

25 k^{2} ⩽ 9 + 16 k^{2}

k^{2} ⩽ 1

- 1 ⩽ k ⩽ 1

k {(x)}_{m i n} = - 1

k {(x)}_{m a x} = 1

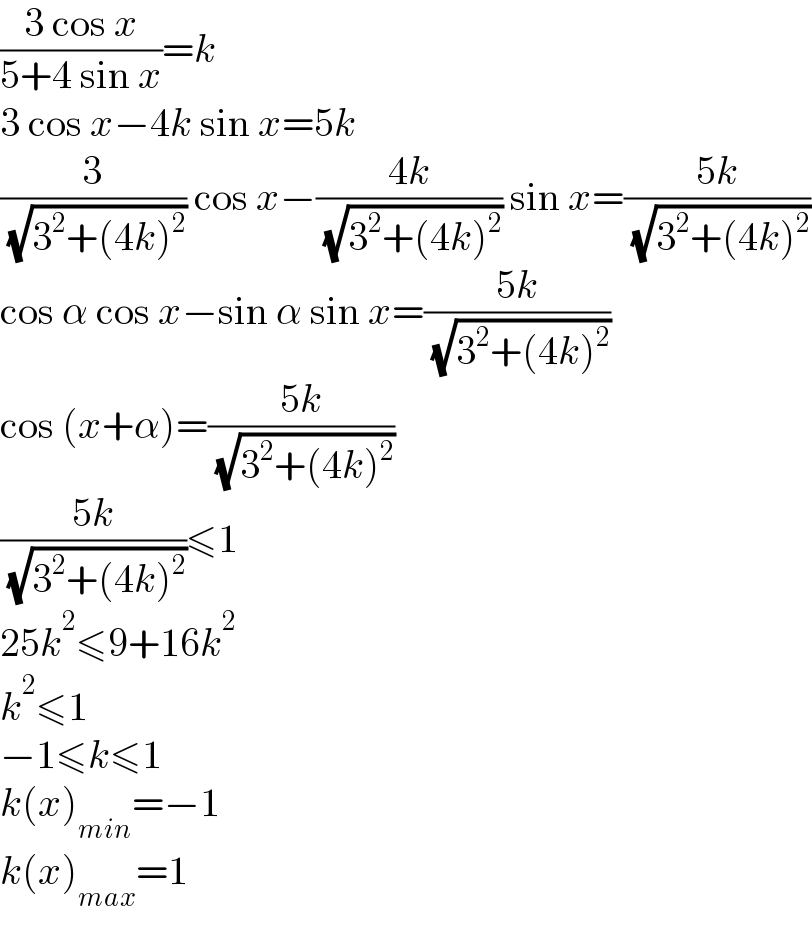
Answered by MJS_new last updated on 09/Jan/22

\frac{3 c}{5 + 4 s} = \pm \frac{3 \sqrt{1 - s^{2}}}{5 + 4 s}

\pm \frac{d}{d s} [\frac{3 \sqrt{1 - s^{2}}}{5 + 4 s}] = 0

\mp \frac{3 (5 s + 4)}{{(4 s + 5)}^{2} \sqrt{1 - s^{2}}} = 0 \Rightarrow s = - \frac{4}{5} \Rightarrow - 1 ⩽ K ⩽ 1

Commented by blackmamba last updated on 09/Jan/22

y e s

Answered by cortano1 last updated on 09/Jan/22

(Q) G i v e n K (x) = \frac{3 \cos x}{5 + 4 \sin x}

F i n d m a x & m i n o f K (x)

L e t y = K (x) = \frac{3 \cos x}{5 + 4 \sin x}

5 y + 4 y \sin x = 3 \cos x

5 y = 3 \cos x - 4 y \sin x \dots (i)

B y C a u c h y - S c h w a r z

∣ 3 \cos x - 4 y \sin x ∣ ⩽ \sqrt{{(3)}^{2} + {(- 4 y)}^{2}}

∣ 5 y ∣ ⩽ \sqrt{9 + 16 y^{2}}

\Leftrightarrow 25 y^{2} ⩽ 9 + 16 y^{2}

\Leftrightarrow 9 (y^{2} - 1) ⩽ 0

\Leftrightarrow - 1 ⩽ y ⩽ 1

∴ - 1 ⩽ K (x) ⩽ 1 \Rightarrow \begin{matrix} m a x K (x) = 1 \\ m i n K (x) = - 1 \end{matrix}}

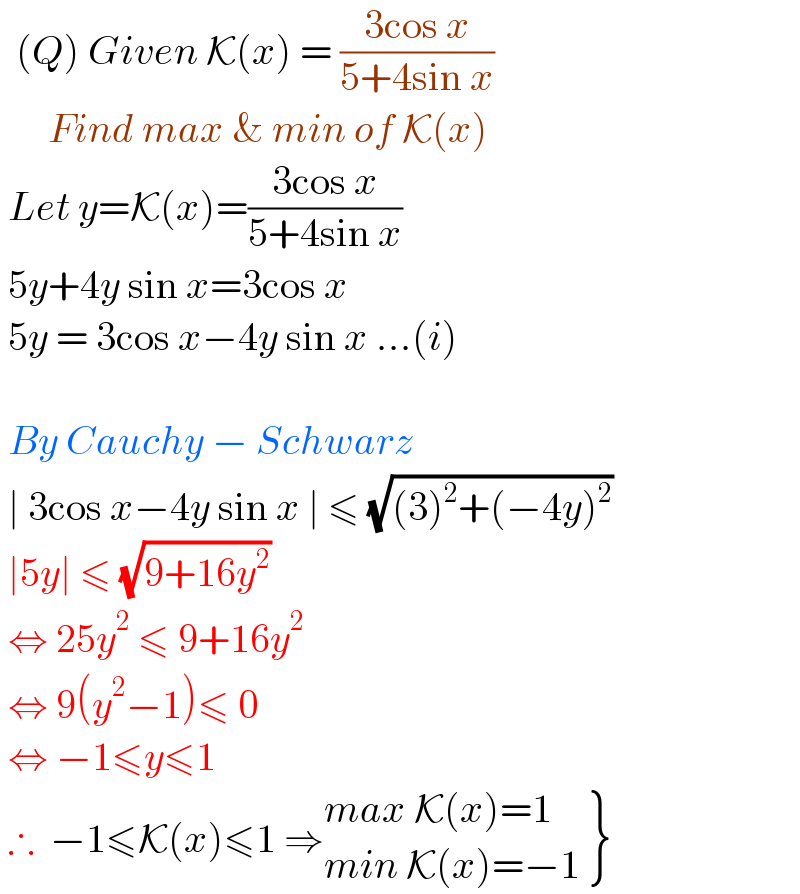
Commented by blackmamba last updated on 09/Jan/22

y e s