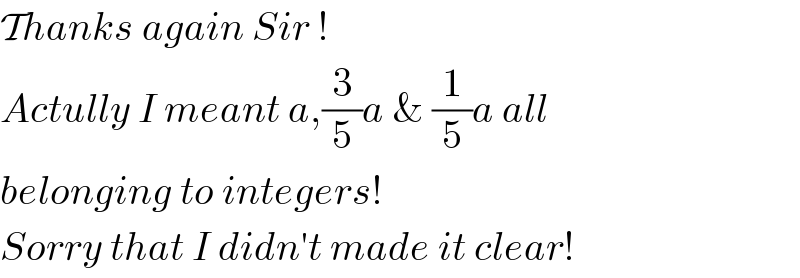Question Number 94025 by Rasheed.Sindhi last updated on 16/May/20
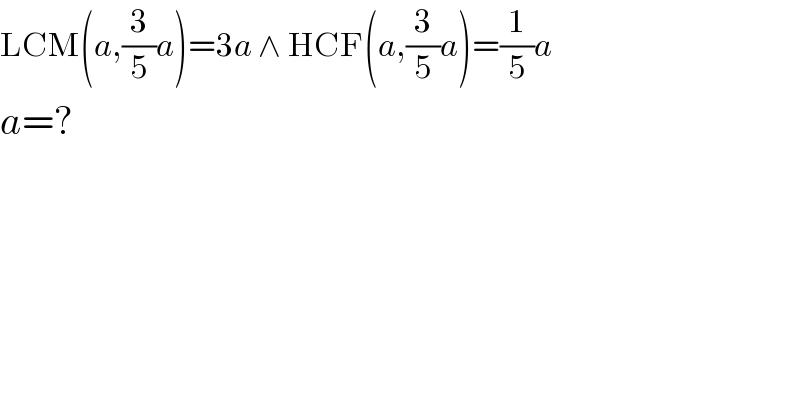
Commented by som(math1967) last updated on 16/May/20
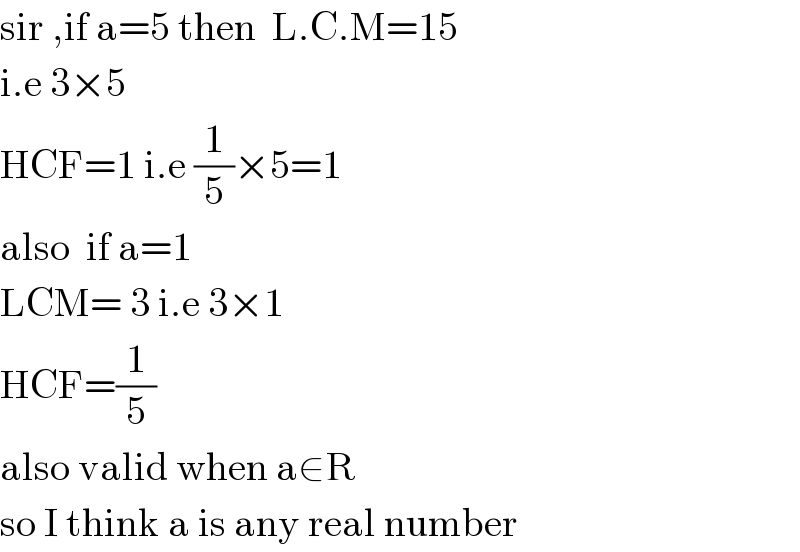
Commented by Rasheed.Sindhi last updated on 16/May/20

Commented by Rasheed.Sindhi last updated on 16/May/20

Commented by som(math1967) last updated on 17/May/20
![If a=−3 [−3∈Z] ∴ LCM of −3,((−9)/5) is −9 i.e 3×−3 HCF is((−3)/5) i.e−3×(1/5) ∴true if a∈Z also we know Z∈R](https://www.tinkutara.com/question/Q94131.png)
Commented by Rasheed.Sindhi last updated on 17/May/20