Question Number 101713 by I want to learn more last updated on 04/Jul/20
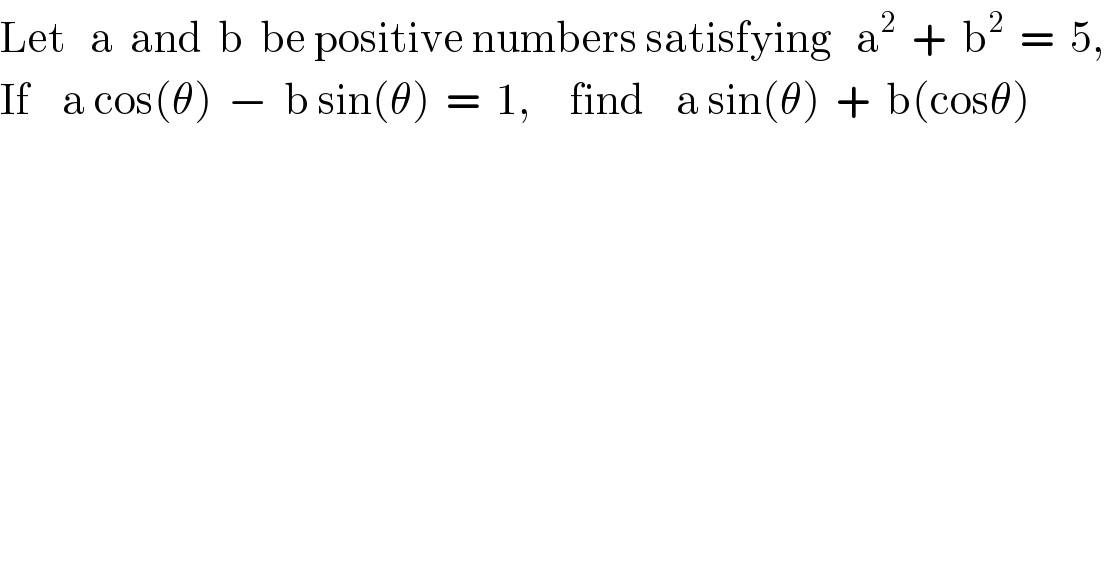
$$\mathrm{Let}\:\:\:\mathrm{a}\:\:\mathrm{and}\:\:\mathrm{b}\:\:\mathrm{be}\:\mathrm{positive}\:\mathrm{numbers}\:\mathrm{satisfying}\:\:\:\mathrm{a}^{\mathrm{2}} \:\:+\:\:\mathrm{b}^{\mathrm{2}} \:\:=\:\:\mathrm{5}, \\ $$$$\mathrm{If}\:\:\:\:\mathrm{a}\:\mathrm{cos}\left(\theta\right)\:\:−\:\:\mathrm{b}\:\mathrm{sin}\left(\theta\right)\:\:=\:\:\mathrm{1},\:\:\:\:\:\mathrm{find}\:\:\:\:\mathrm{a}\:\mathrm{sin}\left(\theta\right)\:\:+\:\:\mathrm{b}\left(\mathrm{cos}\theta\right) \\ $$
Answered by mr W last updated on 04/Jul/20
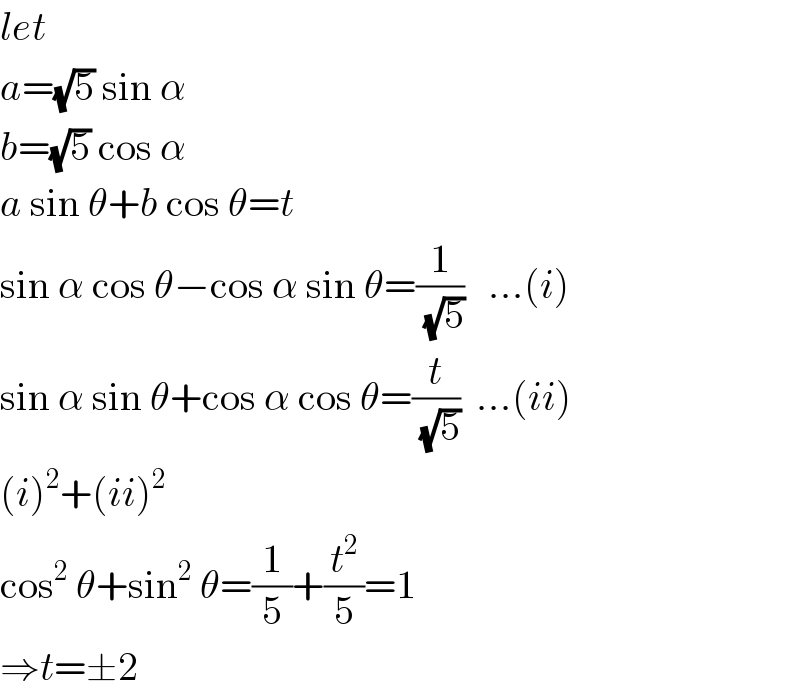
$${let} \\ $$$${a}=\sqrt{\mathrm{5}}\:\mathrm{sin}\:\alpha \\ $$$${b}=\sqrt{\mathrm{5}}\:\mathrm{cos}\:\alpha \\ $$$${a}\:\mathrm{sin}\:\theta+{b}\:\mathrm{cos}\:\theta={t} \\ $$$$\mathrm{sin}\:\alpha\:\mathrm{cos}\:\theta−\mathrm{cos}\:\alpha\:\mathrm{sin}\:\theta=\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}\:\:\:…\left({i}\right) \\ $$$$\mathrm{sin}\:\alpha\:\mathrm{sin}\:\theta+\mathrm{cos}\:\alpha\:\mathrm{cos}\:\theta=\frac{{t}}{\:\sqrt{\mathrm{5}}}\:\:…\left({ii}\right) \\ $$$$\left({i}\right)^{\mathrm{2}} +\left({ii}\right)^{\mathrm{2}} \\ $$$$\mathrm{cos}^{\mathrm{2}} \:\theta+\mathrm{sin}^{\mathrm{2}} \:\theta=\frac{\mathrm{1}}{\mathrm{5}}+\frac{{t}^{\mathrm{2}} }{\mathrm{5}}=\mathrm{1} \\ $$$$\Rightarrow{t}=\pm\mathrm{2} \\ $$
Commented by I want to learn more last updated on 04/Jul/20

$$\mathrm{Thanks}\:\mathrm{sir} \\ $$
Answered by Dwaipayan Shikari last updated on 04/Jul/20

$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} =\mathrm{1}+{x}^{\mathrm{2}} \\ $$$${x}=\pm\mathrm{2} \\ $$
Commented by Dwaipayan Shikari last updated on 04/Jul/20

$${Thanking}\:{you} \\ $$
Commented by mr W last updated on 04/Jul/20

$${good}\:{sir}! \\ $$
Commented by I want to learn more last updated on 04/Jul/20

$$\mathrm{Thanks}\:\mathrm{sir} \\ $$
Answered by john santu last updated on 04/Jul/20
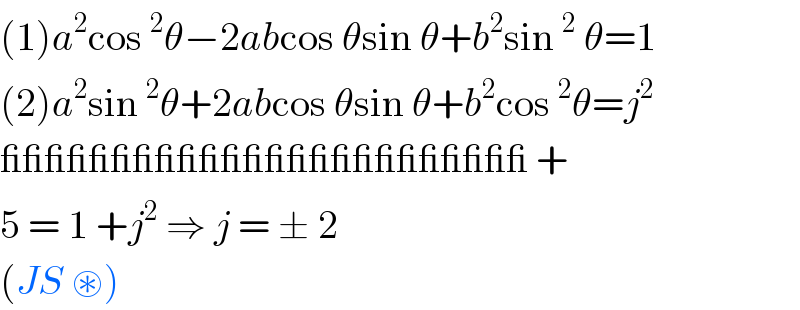
$$\left(\mathrm{1}\right){a}^{\mathrm{2}} \mathrm{cos}\:^{\mathrm{2}} \theta−\mathrm{2}{ab}\mathrm{cos}\:\theta\mathrm{sin}\:\theta+{b}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \:\theta=\mathrm{1} \\ $$$$\left(\mathrm{2}\right){a}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \theta+\mathrm{2}{ab}\mathrm{cos}\:\theta\mathrm{sin}\:\theta+{b}^{\mathrm{2}} \mathrm{cos}\:^{\mathrm{2}} \theta={j}^{\mathrm{2}} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\:+ \\ $$$$\mathrm{5}\:=\:\mathrm{1}\:+{j}^{\mathrm{2}} \:\Rightarrow\:{j}\:=\:\pm\:\mathrm{2}\: \\ $$$$\left({JS}\:\circledast\right) \\ $$
Commented by I want to learn more last updated on 04/Jul/20

$$\mathrm{Thanks}\:\mathrm{sir} \\ $$
