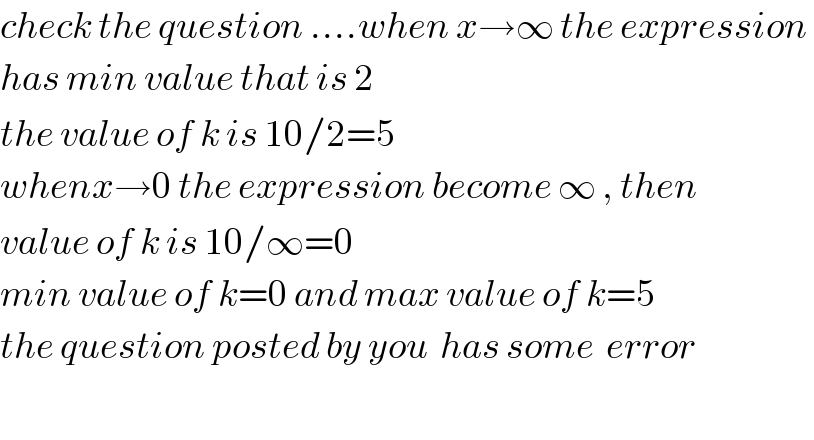Menu Close

Let-a-b-are-positive-real-numbers-such-that-a-b-10-then-the-smallest-value-of-the-constant-k-for-which-x-2-ax-x-2-bx-lt-k-for-all-x-gt-0-is-

Tinku Tara 0 Comments
Pin




Question Number 33940 by rahul 19 last updated on 28/Apr/18
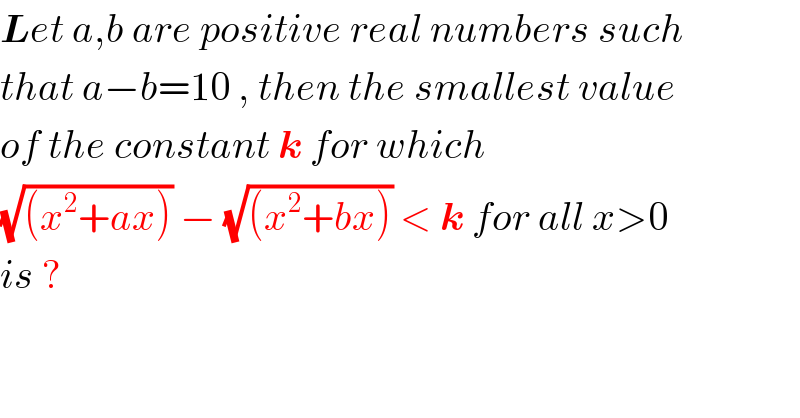
Let a,b are positive real numbers such that a−b=10 , then the smallest value of the constant k for which (√((x^2 +ax))) − (√((x^2 +bx))) < k for all x>0 is ?
$$\boldsymbol{{L}}{et}\:{a},{b}\:{are}\:{positive}\:{real}\:{numbers}\:{such} \\ $$$${that}\:{a}−{b}=\mathrm{10}\:,\:{then}\:{the}\:{smallest}\:{value} \\ $$$${of}\:{the}\:{constant}\:\boldsymbol{{k}}\:{for}\:{which}\: \\ $$$$\sqrt{\left({x}^{\mathrm{2}} +{ax}\right)}\:−\:\sqrt{\left({x}^{\mathrm{2}} +{bx}\right)}\:<\:\boldsymbol{{k}}\:{for}\:{all}\:{x}>\mathrm{0}\: \\ $$$${is}\:? \\ $$
Answered by MJS last updated on 28/Apr/18
a=r+5 b=r−5 (√(x^2 +rx+5x))−(√(x^2 +rx−5x))<k we have to show that lim_(x→∞) (√(x^2 +rx+5x))−(√(x^2 +rx−5x))=5 sorry I have no time right now... (√(x^2 +rx+5x))−(√(x^2 +rx−5x))=k (I.) [x=y ⇒ (1/x)=(1/y)] (1/( (√(x^2 +rx+5x))−(√(x^2 +rx−5x))))=(1/k) [(1/(u−v))=((u+v)/((u−v)(u+v)))=((u+v)/(u^2 −v^2 ))] (((√(x^2 +rx+5x))+(√(x^2 +rx−5x)))/(x^2 +rx+5x−(x^2 +rx−5x)))=(1/k) (((√(x^2 +rx+5x))+(√(x^2 +rx−5x)))/(10x))=(1/k) (√(x^2 +rx+5x))+(√(x^2 +rx−5x))=((10x)/k) (II.) 2(√(x^2 +rx+5x))=k+((10x)/k) (I.+II.) 4(x^2 +rx+5x)=k^2 +20x+((100x^2 )/k^2 ) (4−((100)/k^2 ))x^2 +4rx−k^2 =0 x^2 +((k^2 r)/(k^2 −25))x−(k^4 /(4(k^2 −25)))=0 x=−((k^2 r)/(2(k^2 −25)))±(k^2 /(2(k^2 −25)))(√(k^2 +r^2 −25))= =((r±(√(k^2 +r^2 −25)))/(2(k^2 −25)))k^2 (1/x)=((2(k^2 −25))/(r±(√(k^2 +r^2 −25))))×(1/k^2 ) x→∞ ⇒ k^2 −25=0 ⇒ k=±5 (√(x^2 +rx+5x))−(√(x^2 +rx−5x))>0 ⇒ k=5
$${a}={r}+\mathrm{5} \\ $$$${b}={r}−\mathrm{5} \\ $$$$\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}−\sqrt{{x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}}<{k} \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{to}\:\mathrm{show}\:\mathrm{that} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}−\sqrt{{x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}}=\mathrm{5} \\ $$$$\mathrm{sorry}\:\mathrm{I}\:\mathrm{have}\:\mathrm{no}\:\mathrm{time}\:\mathrm{right}\:\mathrm{now}… \\ $$$$ \\ $$$$ \\ $$$$\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}−\sqrt{{x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}}={k}\:\:\:\:\:\left({I}.\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\left[{x}={y}\:\Rightarrow\:\frac{\mathrm{1}}{{x}}=\frac{\mathrm{1}}{{y}}\right] \\ $$$$\frac{\mathrm{1}}{\:\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}−\sqrt{{x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}}}=\frac{\mathrm{1}}{{k}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\left[\frac{\mathrm{1}}{{u}−{v}}=\frac{{u}+{v}}{\left({u}−{v}\right)\left({u}+{v}\right)}=\frac{{u}+{v}}{{u}^{\mathrm{2}} −{v}^{\mathrm{2}} }\right] \\ $$$$\frac{\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}+\sqrt{{x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}}}{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}−\left({x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}\right)}=\frac{\mathrm{1}}{{k}} \\ $$$$\frac{\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}+\sqrt{{x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}}}{\mathrm{10}{x}}=\frac{\mathrm{1}}{{k}} \\ $$$$\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}+\sqrt{{x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}}=\frac{\mathrm{10}{x}}{{k}}\:\:\:\:\:\left({II}.\right) \\ $$$$\mathrm{2}\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}={k}+\frac{\mathrm{10}{x}}{{k}}\:\:\:\:\:\left({I}.+{II}.\right) \\ $$$$\mathrm{4}\left({x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}\right)={k}^{\mathrm{2}} +\mathrm{20}{x}+\frac{\mathrm{100}{x}^{\mathrm{2}} }{{k}^{\mathrm{2}} } \\ $$$$\left(\mathrm{4}−\frac{\mathrm{100}}{{k}^{\mathrm{2}} }\right){x}^{\mathrm{2}} +\mathrm{4}{rx}−{k}^{\mathrm{2}} =\mathrm{0} \\ $$$${x}^{\mathrm{2}} +\frac{{k}^{\mathrm{2}} {r}}{{k}^{\mathrm{2}} −\mathrm{25}}{x}−\frac{{k}^{\mathrm{4}} }{\mathrm{4}\left({k}^{\mathrm{2}} −\mathrm{25}\right)}=\mathrm{0} \\ $$$${x}=−\frac{{k}^{\mathrm{2}} {r}}{\mathrm{2}\left({k}^{\mathrm{2}} −\mathrm{25}\right)}\pm\frac{{k}^{\mathrm{2}} }{\mathrm{2}\left({k}^{\mathrm{2}} −\mathrm{25}\right)}\sqrt{{k}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{25}}= \\ $$$$=\frac{{r}\pm\sqrt{{k}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{25}}}{\mathrm{2}\left({k}^{\mathrm{2}} −\mathrm{25}\right)}{k}^{\mathrm{2}} \\ $$$$\frac{\mathrm{1}}{{x}}=\frac{\mathrm{2}\left({k}^{\mathrm{2}} −\mathrm{25}\right)}{{r}\pm\sqrt{{k}^{\mathrm{2}} +{r}^{\mathrm{2}} −\mathrm{25}}}×\frac{\mathrm{1}}{{k}^{\mathrm{2}} } \\ $$$${x}\rightarrow\infty\:\Rightarrow\:{k}^{\mathrm{2}} −\mathrm{25}=\mathrm{0}\:\Rightarrow\:{k}=\pm\mathrm{5} \\ $$$$\sqrt{{x}^{\mathrm{2}} +{rx}+\mathrm{5}{x}}−\sqrt{{x}^{\mathrm{2}} +{rx}−\mathrm{5}{x}}>\mathrm{0}\:\Rightarrow\:{k}=\mathrm{5} \\ $$
Commented by rahul 19 last updated on 28/Apr/18
No problem sir. btw, how you came to know it should be equal to 5?
$${No}\:\mathrm{problem}\:\mathrm{sir}.\: \\ $$$$\mathrm{btw},\:{how}\:{you}\:{came}\:{to}\:{know}\:{it}\:{should} \\ $$$${be}\:{equal}\:{to}\:\mathrm{5}? \\ $$
Commented by MJS last updated on 28/Apr/18
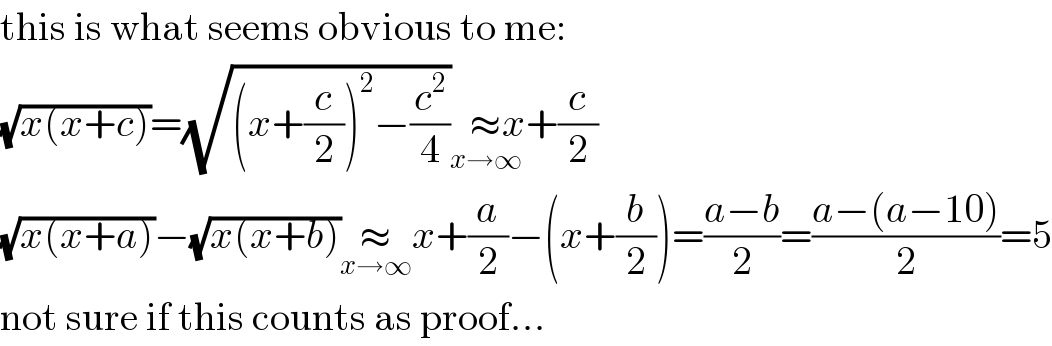
this is what seems obvious to me: (√(x(x+c)))=(√((x+(c/2))^2 −(c^2 /4)))≈_(x→∞) x+(c/2) (√(x(x+a)))−(√(x(x+b)))≈_(x→∞) x+(a/2)−(x+(b/2))=((a−b)/2)=((a−(a−10))/2)=5 not sure if this counts as proof...
$$\mathrm{this}\:\mathrm{is}\:\mathrm{what}\:\mathrm{seems}\:\mathrm{obvious}\:\mathrm{to}\:\mathrm{me}: \\ $$$$\sqrt{{x}\left({x}+{c}\right)}=\sqrt{\left({x}+\frac{{c}}{\mathrm{2}}\right)^{\mathrm{2}} −\frac{{c}^{\mathrm{2}} }{\mathrm{4}}}\underset{{x}\rightarrow\infty} {\approx}{x}+\frac{{c}}{\mathrm{2}} \\ $$$$\sqrt{{x}\left({x}+{a}\right)}−\sqrt{{x}\left({x}+{b}\right)}\underset{{x}\rightarrow\infty} {\approx}{x}+\frac{{a}}{\mathrm{2}}−\left({x}+\frac{{b}}{\mathrm{2}}\right)=\frac{{a}−{b}}{\mathrm{2}}=\frac{{a}−\left({a}−\mathrm{10}\right)}{\mathrm{2}}=\mathrm{5} \\ $$$$\mathrm{not}\:\mathrm{sure}\:\mathrm{if}\:\mathrm{this}\:\mathrm{counts}\:\mathrm{as}\:\mathrm{proof}… \\ $$
Commented by rahul 19 last updated on 28/Apr/18
Thank you sir .
$$\mathscr{T}{hank}\:\mathrm{you}\:\mathrm{sir}\:. \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 28/Apr/18
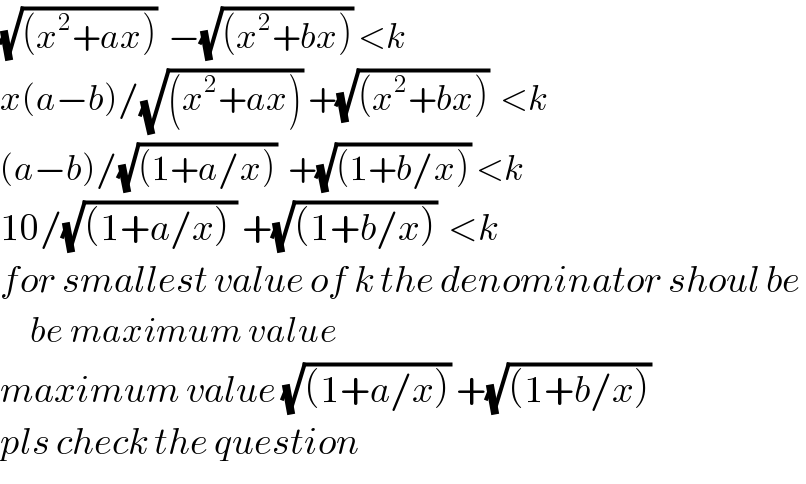
(√((x^2 +ax))) −(√((x^2 +bx))) <k x(a−b)/(√((x_ ^2 +ax))) +(√((x^2 +bx))) <k (a−b)/(√((1+a/x))) +(√((1+b/x))) <k 10/(√((1+a/x) )) +(√((1+b/x))) <k for smallest value of k the denominator shoul be be maximum value maximum value (√((1+a/x))) +(√((1+b/x))) pls check the question
$$\sqrt{\left({x}^{\mathrm{2}} +{ax}\right)}\:\:−\sqrt{\left({x}^{\mathrm{2}} +{bx}\right)}\:<{k} \\ $$$${x}\left({a}−{b}\right)/\sqrt{\left({x}_{} ^{\mathrm{2}} +{ax}\right)}\:+\sqrt{\left({x}^{\mathrm{2}} +{bx}\right)}\:\:<{k} \\ $$$$\left({a}−{b}\right)/\sqrt{\left(\mathrm{1}+{a}/{x}\right)}\:\:+\sqrt{\left(\mathrm{1}+{b}/{x}\right)}\:<{k} \\ $$$$\mathrm{10}/\sqrt{\left(\mathrm{1}+{a}/{x}\right)\:}\:+\sqrt{\left(\mathrm{1}+{b}/{x}\right)}\:\:<{k} \\ $$$${for}\:{smallest}\:{value}\:{of}\:{k}\:{the}\:{denominator}\:{shoul}\:{be}\: \\ $$$$\:\:\:\:\:{be}\:{maximum}\:{value} \\ $$$${maximum}\:{value}\:\sqrt{\left(\mathrm{1}+{a}/{x}\right)}\:+\sqrt{\left(\mathrm{1}+{b}/{x}\right)} \\ $$$${pls}\:{check}\:{the}\:{question} \\ $$
Commented by rahul 19 last updated on 28/Apr/18
Wow! nice solution sir!
$${Wow}!\:{nice}\:{solution}\:{sir}! \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 28/Apr/18
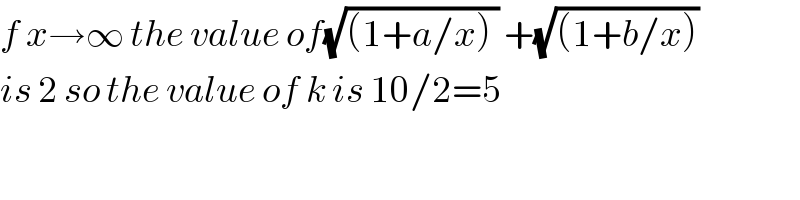
f x→∞ the value of(√((1+a/x) )) +(√((1+b/x))) is 2 so the value of k is 10/2=5
$${f}\:{x}\rightarrow\infty\:{the}\:{value}\:{of}\sqrt{\left(\mathrm{1}+{a}/{x}\right)\:}\:+\sqrt{\left(\mathrm{1}+{b}/{x}\right)} \\ $$$${is}\:\mathrm{2}\:{so}\:{the}\:{value}\:{of}\:{k}\:{is}\:\mathrm{10}/\mathrm{2}=\mathrm{5} \\ $$
Commented by rahul 19 last updated on 29/Apr/18
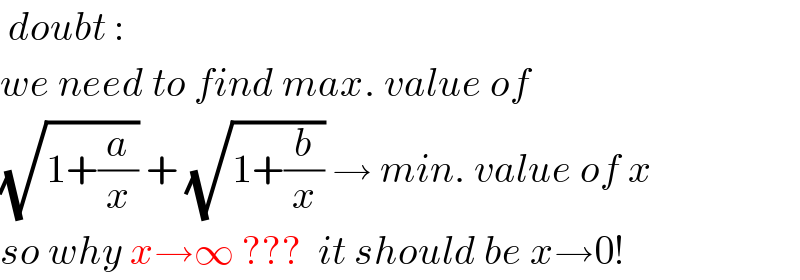
doubt : we need to find max. value of (√(1+(a/x))) + (√(1+(b/x))) → min. value of x so why x→∞ ??? it should be x→0!
$$\:{doubt}\:: \\ $$$${we}\:{need}\:{to}\:{find}\:{max}.\:{value}\:{of} \\ $$$$\sqrt{\mathrm{1}+\frac{{a}}{{x}}}\:+\:\sqrt{\mathrm{1}+\frac{{b}}{{x}}}\:\rightarrow\:{min}.\:{value}\:{of}\:{x} \\ $$$${so}\:{why}\:{x}\rightarrow\infty\:???\:\:{it}\:{should}\:{be}\:{x}\rightarrow\mathrm{0}! \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Apr/18
check the question ....when x→∞ the expression has min value that is 2 the value of k is 10/2=5 whenx→0 the expression become ∞ , then value of k is 10/∞=0 min value of k=0 and max value of k=5 the question posted by you has some error
$${check}\:{the}\:{question}\:….{when}\:{x}\rightarrow\infty\:{the}\:{expression}\: \\ $$$${has}\:{min}\:{value}\:{that}\:{is}\:\mathrm{2} \\ $$$${the}\:{value}\:{of}\:{k}\:{is}\:\mathrm{10}/\mathrm{2}=\mathrm{5} \\ $$$${whenx}\rightarrow\mathrm{0}\:{the}\:{expression}\:{become}\:\infty\:,\:{then}\: \\ $$$${value}\:{of}\:{k}\:{is}\:\mathrm{10}/\infty=\mathrm{0}\: \\ $$$${min}\:{value}\:{of}\:{k}=\mathrm{0}\:{and}\:{max}\:{value}\:{of}\:{k}=\mathrm{5} \\ $$$${the}\:{question}\:{posted}\:{by}\:{you}\:\:{has}\:{some}\:\:{error} \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *