Question Number 110223 by mohammad17 last updated on 27/Aug/20
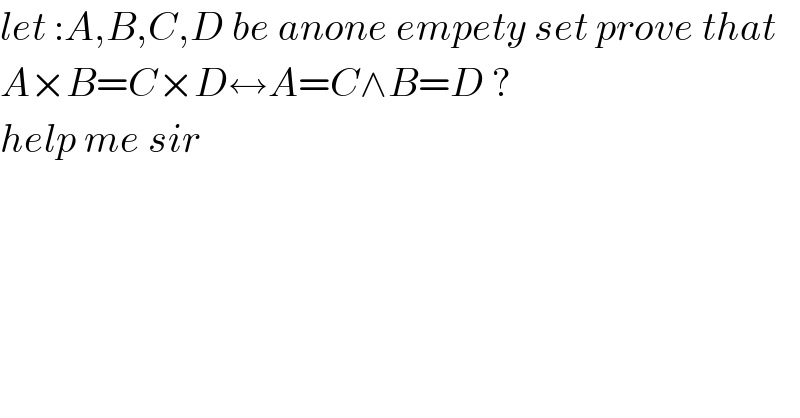
Commented by kaivan.ahmadi last updated on 27/Aug/20
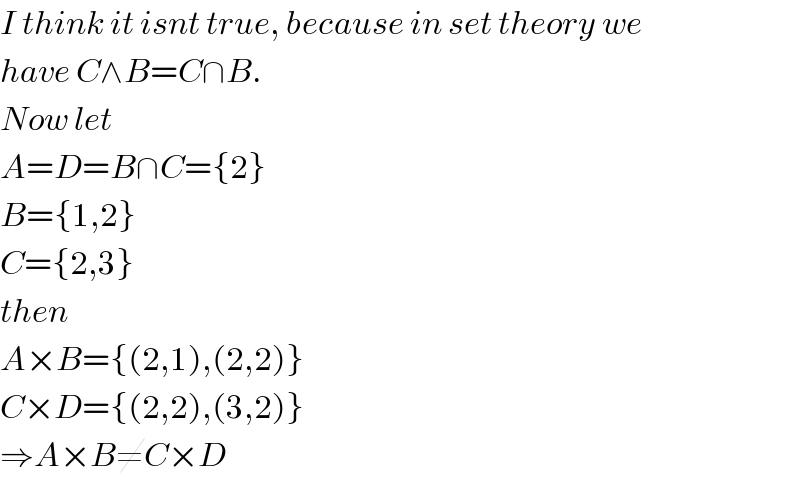
Commented by mohammad17 last updated on 28/Aug/20
Commented by kaivan.ahmadi last updated on 28/Aug/20
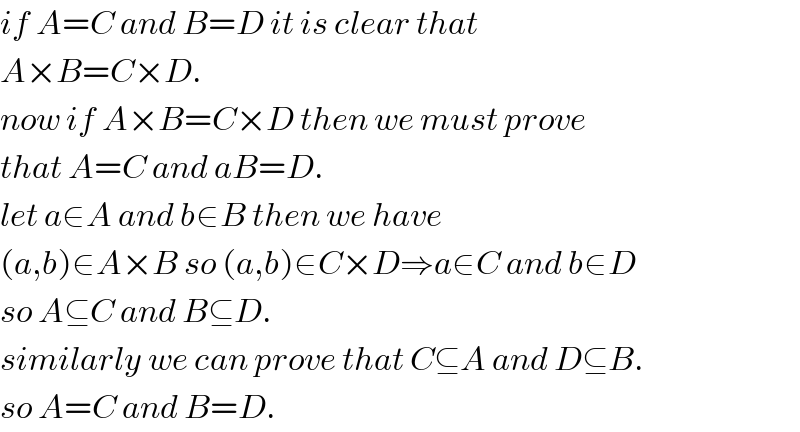
Commented by kaivan.ahmadi last updated on 28/Aug/20

