Question Number 158124 by HongKing last updated on 31/Oct/21
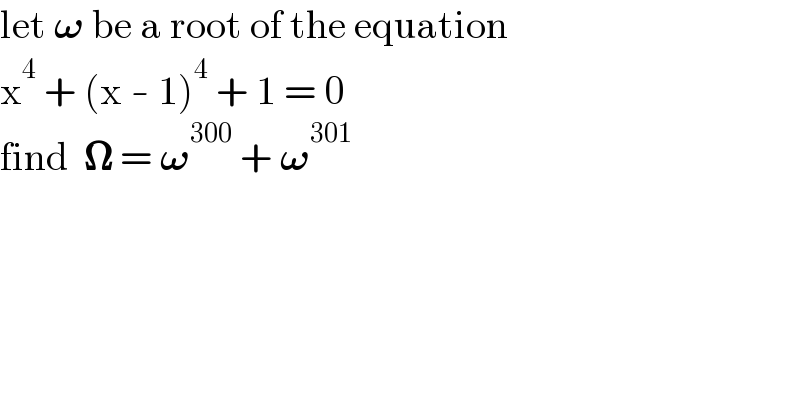
$$\mathrm{let}\:\boldsymbol{\omega}\:\mathrm{be}\:\mathrm{a}\:\mathrm{root}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\mathrm{x}^{\mathrm{4}} \:+\:\left(\mathrm{x}\:-\:\mathrm{1}\right)^{\mathrm{4}} \:+\:\mathrm{1}\:=\:\mathrm{0} \\ $$$$\mathrm{find}\:\:\boldsymbol{\Omega}\:=\:\boldsymbol{\omega}^{\mathrm{300}} \:+\:\boldsymbol{\omega}^{\mathrm{301}} \\ $$
Answered by Rasheed.Sindhi last updated on 31/Oct/21
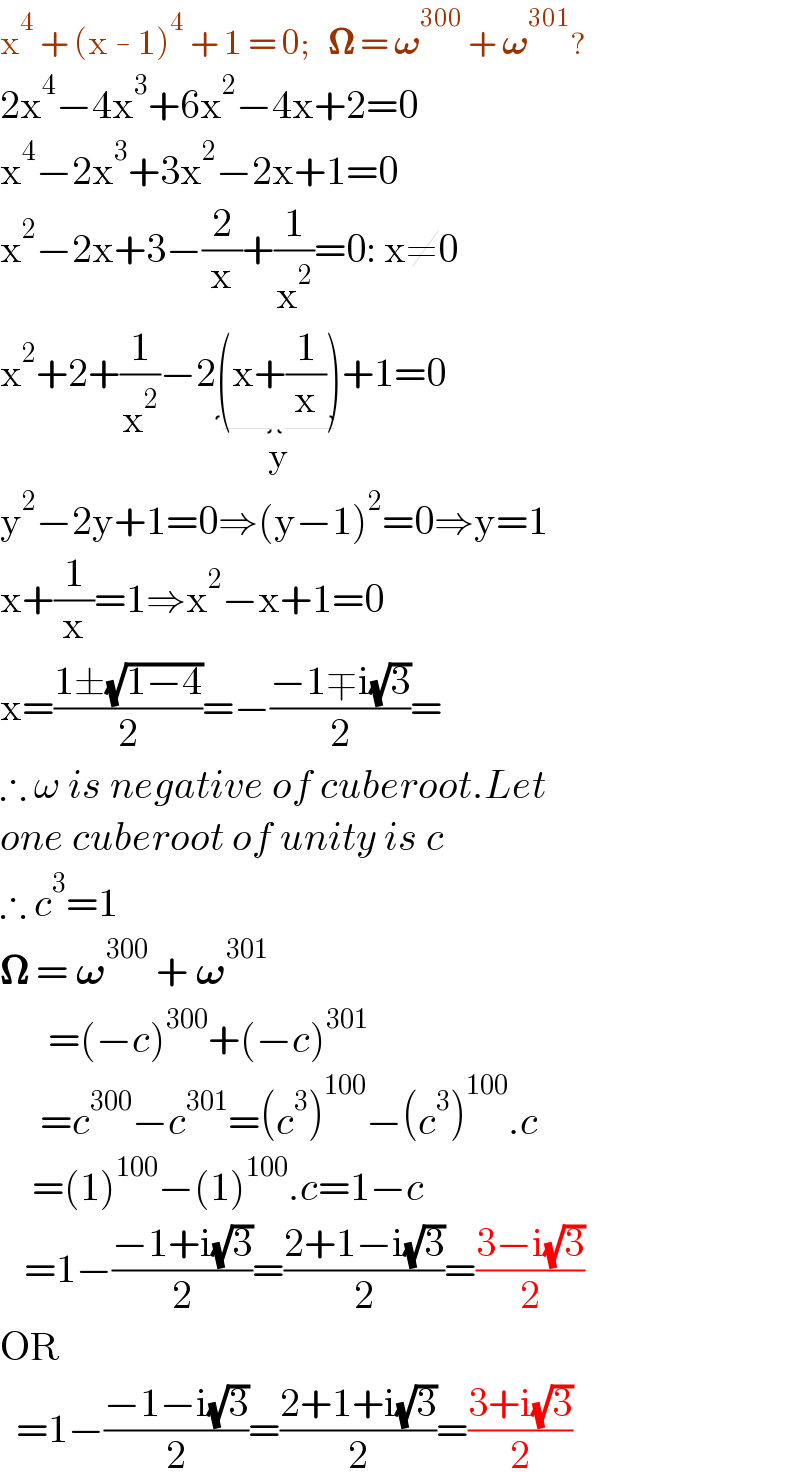
$$\mathrm{x}^{\mathrm{4}} \:+\:\left(\mathrm{x}\:-\:\mathrm{1}\right)^{\mathrm{4}} \:+\:\mathrm{1}\:=\:\mathrm{0};\:\:\:\boldsymbol{\Omega}\:=\:\boldsymbol{\omega}^{\mathrm{300}} \:+\:\boldsymbol{\omega}^{\mathrm{301}} ? \\ $$$$\mathrm{2x}^{\mathrm{4}} −\mathrm{4x}^{\mathrm{3}} +\mathrm{6x}^{\mathrm{2}} −\mathrm{4x}+\mathrm{2}=\mathrm{0} \\ $$$$\mathrm{x}^{\mathrm{4}} −\mathrm{2x}^{\mathrm{3}} +\mathrm{3x}^{\mathrm{2}} −\mathrm{2x}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{2x}+\mathrm{3}−\frac{\mathrm{2}}{\mathrm{x}}+\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }=\mathrm{0}:\:\mathrm{x}\neq\mathrm{0} \\ $$$$\mathrm{x}^{\mathrm{2}} +\mathrm{2}+\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }−\mathrm{2}\underset{\mathrm{y}} {\underbrace{\left(\mathrm{x}+\frac{\mathrm{1}}{\mathrm{x}}\right)}}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{y}^{\mathrm{2}} −\mathrm{2y}+\mathrm{1}=\mathrm{0}\Rightarrow\left(\mathrm{y}−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{0}\Rightarrow\mathrm{y}=\mathrm{1} \\ $$$$\mathrm{x}+\frac{\mathrm{1}}{\mathrm{x}}=\mathrm{1}\Rightarrow\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{x}=\frac{\mathrm{1}\pm\sqrt{\mathrm{1}−\mathrm{4}}}{\mathrm{2}}=−\frac{−\mathrm{1}\mp\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}= \\ $$$$\therefore\:\omega\:{is}\:{negative}\:{of}\:{cuberoot}.{Let} \\ $$$${one}\:{cuberoot}\:{of}\:{unity}\:{is}\:{c} \\ $$$$\therefore\:{c}^{\mathrm{3}} =\mathrm{1} \\ $$$$\boldsymbol{\Omega}\:=\:\boldsymbol{\omega}^{\mathrm{300}} \:+\:\boldsymbol{\omega}^{\mathrm{301}} \:\: \\ $$$$\:\:\:\:\:\:=\left(−{c}\right)^{\mathrm{300}} +\left(−{c}\right)^{\mathrm{301}} \\ $$$$\:\:\:\:\:={c}^{\mathrm{300}} −{c}^{\mathrm{301}} =\left({c}^{\mathrm{3}} \right)^{\mathrm{100}} −\left({c}^{\mathrm{3}} \right)^{\mathrm{100}} .{c} \\ $$$$\:\:\:\:=\left(\mathrm{1}\right)^{\mathrm{100}} −\left(\mathrm{1}\right)^{\mathrm{100}} .{c}=\mathrm{1}−{c} \\ $$$$\:\:\:=\mathrm{1}−\frac{−\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}=\frac{\mathrm{2}+\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}=\frac{\mathrm{3}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\mathrm{OR}\: \\ $$$$\:\:=\mathrm{1}−\frac{−\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}=\frac{\mathrm{2}+\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}=\frac{\mathrm{3}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$
Commented by mr W last updated on 04/Nov/21

$$\omega^{\mathrm{3}} =−\mathrm{1}\:{is}\:{correct}. \\ $$$$\Omega=\frac{\mathrm{3}\pm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:{is}\:{correct}. \\ $$$${but}\:\Omega\neq\mathrm{0} \\ $$
Commented by HongKing last updated on 31/Oct/21
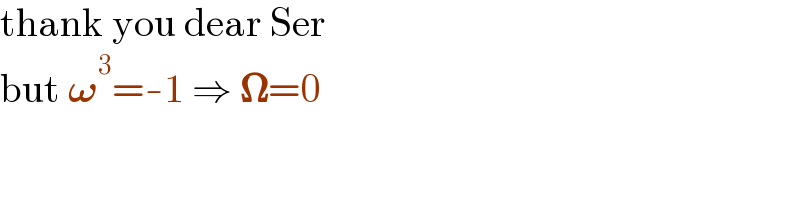
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{dear}\:\mathrm{Ser} \\ $$$$\mathrm{but}\:\boldsymbol{\omega}^{\mathrm{3}} =-\mathrm{1}\:\Rightarrow\:\boldsymbol{\Omega}=\mathrm{0} \\ $$
Commented by Rasheed.Sindhi last updated on 31/Oct/21
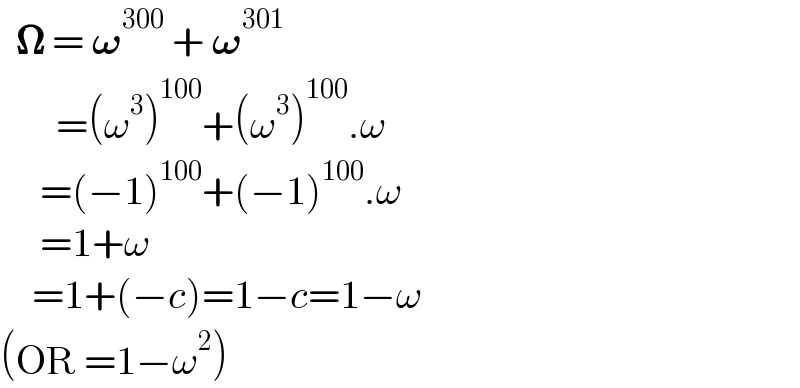
$$\:\:\boldsymbol{\Omega}\:=\:\boldsymbol{\omega}^{\mathrm{300}} \:+\:\boldsymbol{\omega}^{\mathrm{301}} \\ $$$$\:\:\:\:\:\:\:=\left(\omega^{\mathrm{3}} \right)^{\mathrm{100}} +\left(\omega^{\mathrm{3}} \right)^{\mathrm{100}} .\omega \\ $$$$\:\:\:\:\:=\left(−\mathrm{1}\right)^{\mathrm{100}} +\left(−\mathrm{1}\right)^{\mathrm{100}} .\omega \\ $$$$\:\:\:\:\:=\mathrm{1}+\omega \\ $$$$\:\:\:\:=\mathrm{1}+\left(−{c}\right)=\mathrm{1}−{c}=\mathrm{1}−\omega \\ $$$$\left(\mathrm{OR}\:=\mathrm{1}−\omega^{\mathrm{2}} \right) \\ $$
Commented by Rasheed.Sindhi last updated on 01/Nov/21

$$\mathrm{Agree}? \\ $$
Commented by HongKing last updated on 04/Nov/21
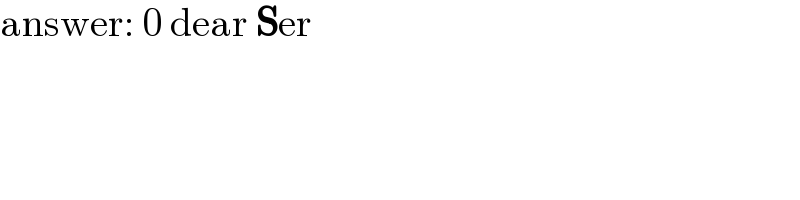
$$\mathrm{answer}:\:\mathrm{0}\:\mathrm{dear}\:\boldsymbol{\mathrm{S}}\mathrm{er} \\ $$
Commented by Rasheed.Sindhi last updated on 04/Nov/21

$${Answer}\:{you}\:{have}\:{may}\:{be}\:{wrong}.{The} \\ $$$${above}\:{comment}\:{is}\:{proof}. \\ $$
Commented by Rasheed.Sindhi last updated on 05/Nov/21

$$\mathbb{T}\mathrm{han}\Bbbk\mathrm{s}\:\mathrm{a}\:\mathbb{L}\mathrm{ot}\:\mathbb{S}\mathrm{ir}! \\ $$
