Question Number 43623 by math khazana by abdo last updated on 12/Sep/18
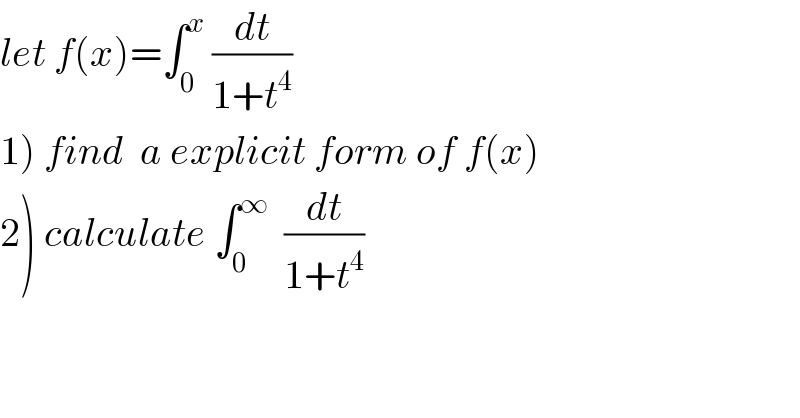
Commented by maxmathsup by imad last updated on 13/Sep/18
![let decompose F(t)=(1/(t^4 +1)) we have F(t)=(1/((t^2 +1)^2 −2t^2 )) =(1/((t^2 +1 −(√2)t)(t^2 +1+(√2)t))) =((at +b)/((t^2 −(√2)t +1))) +((ct +d)/(t^2 +(√2)t +1)) F(−t)=F(t) ⇒((−at +b)/(t^2 +(√2)t +1)) +((−ct +d)/(t^2 −(√2)t +1)) =F(t) ⇒c=−a and b=d ⇒ F(x)=((at +b)/(t^2 −(√2)t +1)) +((−at +b)/(t^2 +(√2)t +1)) F(0) =1 =2b ⇒b=0 ⇒F(t)=((at)/(t^2 −(√2)t +1)) −((at)/(t^2 +(√2)t +1)) F(1) =(1/2) = (a/(2−(√2))) −(a/(2+(√2))) =(((2+(√2)−2+(√2))/2))a =(√2)a ⇒a=(1/(2(√2))) ⇒ F(x)= (1/(2(√2))){ (t/(t^2 −(√2)t +1)) −(t/(t^2 +(√2)t +1))}⇒ ∫ (dt/(1+t^4 )) =(1/(4(√2))){ ∫ ((2t−(√2)+(√2))/(t^2 −(√2)t +1))dt −∫ ((2t +(√2)−(√2))/(t^2 +(√2)t +1))dt} ⇒4(√2) ∫ (dt/(1+t^4 )) =ln∣t^2 −(√2)t +1∣−ln∣t^2 +(√2)t+1∣ +(√2) ∫(dt/(t^2 −(√2)t +1)) −(√2)∫(dt/(t^2 +(√2)t +1)) but ∫ (dt/(t^2 −(√2)t +1)) = ∫ (dt/(t^2 −(2/( (√2)))t +(1/2) +(1/2))) =∫ (dt/((t−(1/( (√2))))^2 +(1/2))) =_(t−(1/( (√2))) =(u/( (√2)))) ∫ (2/(1+u^2 )) (du/( (√2))) =(√2)arctan(u)=(√2)arctan(t(√2)−1) also ∫ (dt/(t^2 +(√2)t +1)) = (√2)arctan(t(√2)+1) ⇒ 4(√2)∫ (dt/(1+t^4 )) =ln∣((t^2 −(√2)t+1)/(t^2 +(√2)t +1))∣ +2 arctan(t(√2)−1)−2 arctan(t(√2)+1) +c ⇒ ∫ (dt/(1+t^4 )) =(1/(4(√2)))ln∣((t^2 −(√2)t +1)/(t^2 +(√2)t +1))∣ +(1/(2(√2))){ arctan(t(√2)−1)−arctan(t(√2)+1)} +c⇒ f(x)= ∫_0 ^x (dt/(1+t^4 )) =[(1/(4(√2)))ln∣((t^2 −(√2)t +1)/(t^2 +(√2)t +1))∣_0 ^x +(1/(2(√2)))[arctan(t(√2)−1)−arctan(t(√2)+1)]_0 ^x f(x)=(1/(4(√2)))ln∣((x^2 −(√2)x +1)/(x^2 +(√2)x +1))∣ +(1/(2(√2))){ arctan(x(√2)−1)−arctan(x(√2)+1) +(π/2)}](https://www.tinkutara.com/question/Q43667.png)
Commented by maxmathsup by imad last updated on 13/Sep/18
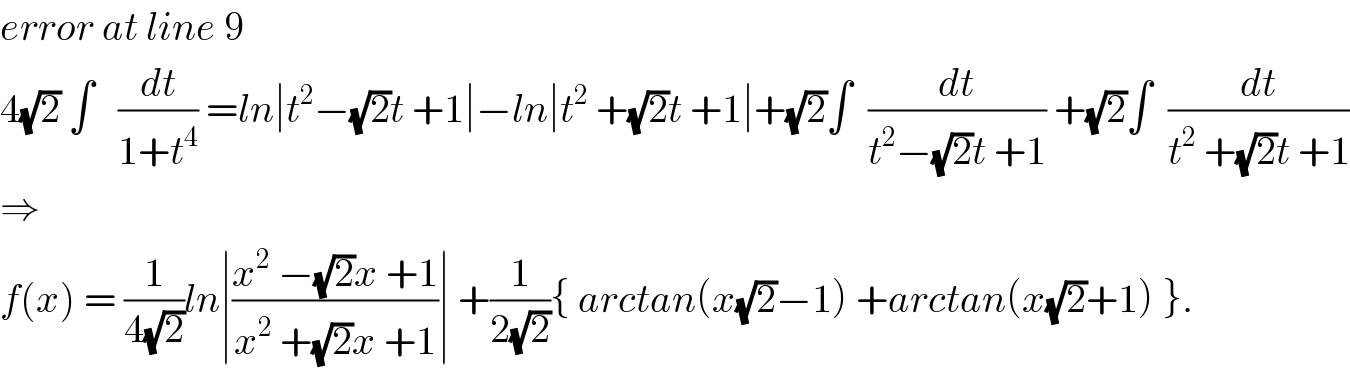
Commented by maxmathsup by imad last updated on 13/Sep/18
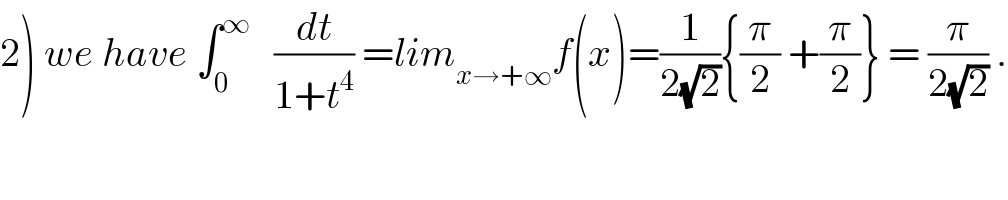
Answered by tanmay.chaudhury50@gmail.com last updated on 13/Sep/18
![∫(dt/(1+t^4 )) ∫((1/t^2 )/(t^2 +(1/t^2 )))dt (1/2)∫(((1+(1/t^2 ))−(1−(1/t^2 )))/(t^2 +(1/t^2 )))dt (1/2)∫((d(t−(1/t)))/((t−(1/t))^2 +2))−(1/2)∫((d(t+(1/t)))/((t+(1/t))^2 −2)) I_1 =(1/2)∫((d(t−(1/t)))/((t−(1/t))^2 +2)) =(1/2)×(1/( (√2) ))tan^(−1) (((t−(1/t))/( (√2))))+c I_2 =(1/2)∫((d(t+(1/t)))/((t+(1/t))^2 −((√2) )^2 )) formula∫(dy/(y^2 −a^2 ))=(1/(2a))∫(((y+a)−(y−a))/((y+a)y−a)))dy (1/(2a))[∫(dy/(y−a))−∫(dy/(y+a))] (1/(2a))ln∣((y−a)/(y+a))∣+c_2 I_2 =(1/2)×(1/(2(√2)))ln∣((t+(1/t)−(√2))/(t+(1/t)+(√2)))∣ so ans is I_1 −I_2 (1/(2(√2)))tan^(−1) (((t−(1/t))/( (√2))))−(1/(4(√2)))ln∣((t+(1/t)−(√2))/(t+(1/t)+(√2)))∣+c so ∫_0 ^x (dt/(1+t^4 )) [(1/(2(√2)))tan^(−1) (((x−(1/x))/( (√2))))−(1/(4(√2)))ln∣((x+(1/x)−(√2))/(x+(1/x)+(√2)))∣]− [(1/(2(√2)))tan^(−1) (−∞)−0 (1/(2(√2)))(−(Π/2)) ans is[ (1/(2(√2)))tan^(−1) (((x−(1/x))/( (√2))))−(1/(4(√2)))ln∣((x+(1/x)−(√2))/(x+(1/x)+(√2)))∣+(Π/(4(√2)))](https://www.tinkutara.com/question/Q43651.png)
