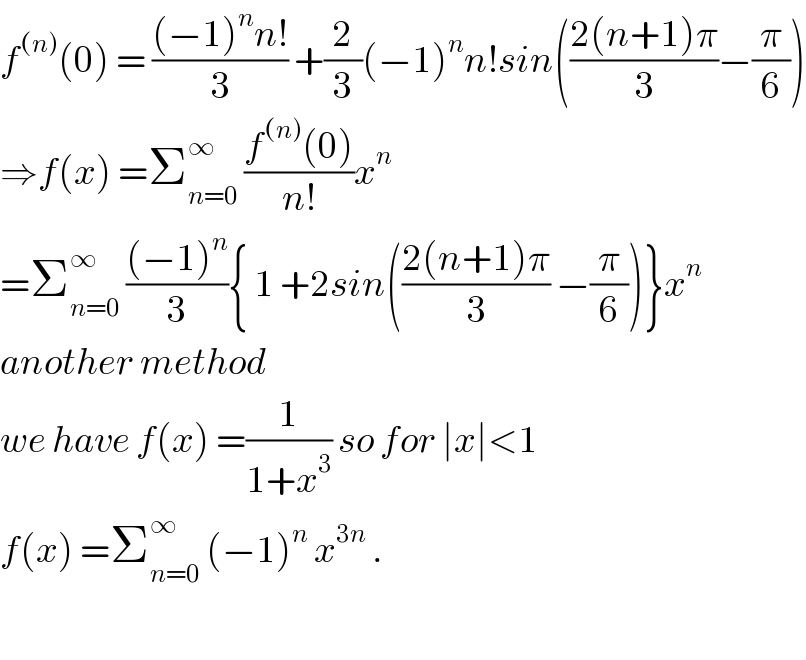Menu Close

let-f-x-1-1-x-3-1-calculate-f-n-x-2-developp-f-at-integr-serie-

Tinku Tara 0 Comments
Pin




Question Number 35987 by abdo mathsup 649 cc last updated on 26/May/18
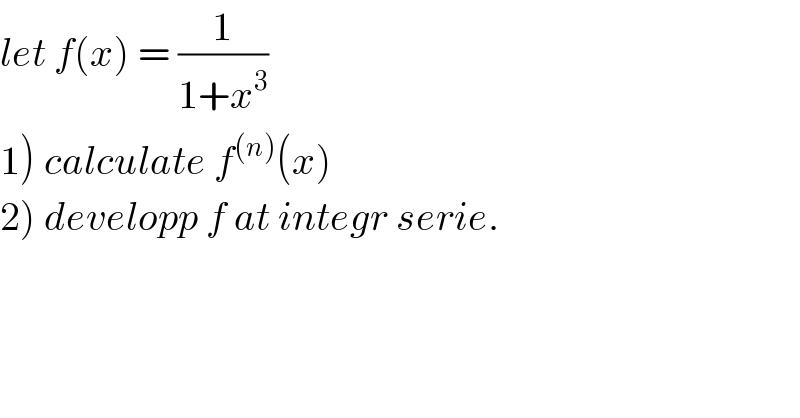
let f(x) = (1/(1+x^3 )) 1) calculate f^((n)) (x) 2) developp f at integr serie.
$${let}\:{f}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{3}} } \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}^{\left({n}\right)} \left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{developp}\:{f}\:{at}\:{integr}\:{serie}. \\ $$
Commented by prof Abdo imad last updated on 27/May/18
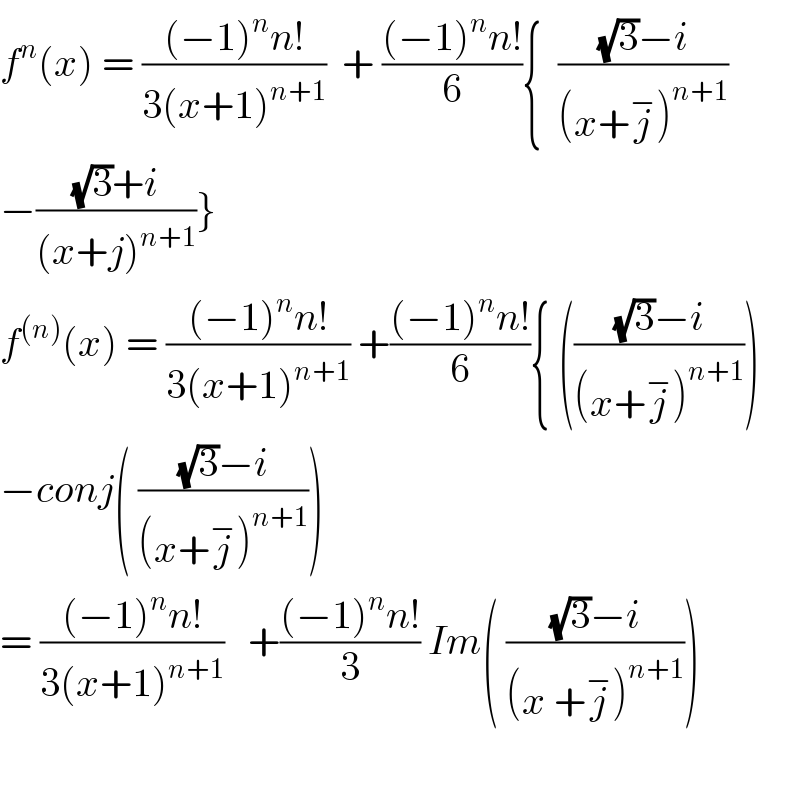
we have f(x)= (1/((x+1)(x^2 −x+1))) let decompose f inside C[x] roots of x^2 −x +1 Δ=1−4=−3=(i(√3))^2 ⇒x_1 =((1 +i(√3))/2) x_2 =((1−(√3))/2) or j =−(1/2) +i((√3)/2) ⇒x_2 =−j and x_1 =−j^− ⇒f(x)=(1/((x+1)(x+j)(x+j^− ))) =(a/(x+1)) +(b/(x+j)) +(c/(x+j^− )) a =lim_(x→−1) (x+1)f(x)= (1/3) b = lim_(x→−j) (x+j)f(x) = (1/((−j+1)(−j+j^− ))) = (1/((j−1)(j−j^− ))) =(1/( (√3)(−(3/2)+i((√3)/2)))) = (2/(3( −(√3) +i))) = ((2( −(√3)−i))/(3(3 +1))) = ((−(√3)−i)/6) c =lim_(x→−j^− ) (x+j^− )f(x) = (1/((−j^− +1)(j−j^− ))) = (1/( (√3)( ((1+i(√3))/2) +1))) = (1/( (√3)( (3/2) +i((√3)/2)))) = (2/(3((√3) +i))) =((2((√3)−i))/(3(4))) = (((√3)−i)/6) ⇒ f(x) = (1/(3(x+1))) −(((√3)+i)/(6(x+j))) +(((√3)−i)/(6(x+j^− ))) ⇒ f^((n)) (x) = (1/3) (((−1)^n n!)/((x+1)^(n+1) )) −(((√3)+i)/6) (((−1)^n n!)/((x+j)^(n+1) )) + (((√3)−i)/6) (((−1)^n n!)/((x+j^− )^(n+1) ))
$${we}\:{have}\:{f}\left({x}\right)=\:\frac{\mathrm{1}}{\left({x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} \:−{x}+\mathrm{1}\right)}\:{let}\:{decompose} \\ $$$${f}\:{inside}\:{C}\left[{x}\right]\:{roots}\:{of}\:{x}^{\mathrm{2}} \:−{x}\:+\mathrm{1} \\ $$$$\Delta=\mathrm{1}−\mathrm{4}=−\mathrm{3}=\left({i}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} \:\Rightarrow{x}_{\mathrm{1}} \:=\frac{\mathrm{1}\:+{i}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$${x}_{\mathrm{2}} \:=\frac{\mathrm{1}−\sqrt{\mathrm{3}}}{\mathrm{2}}\:{or}\:{j}\:=−\frac{\mathrm{1}}{\mathrm{2}}\:+{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:\Rightarrow{x}_{\mathrm{2}} \:=−{j}\:{and} \\ $$$${x}_{\mathrm{1}} \:=−\overset{−} {{j}}\:\:\Rightarrow{f}\left({x}\right)=\frac{\mathrm{1}}{\left({x}+\mathrm{1}\right)\left({x}+{j}\right)\left({x}+\overset{−} {{j}}\right)}\:=\frac{{a}}{{x}+\mathrm{1}}\:+\frac{{b}}{{x}+{j}}\:\:+\frac{{c}}{{x}+\overset{−} {{j}}} \\ $$$${a}\:={lim}_{{x}\rightarrow−\mathrm{1}} \left({x}+\mathrm{1}\right){f}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{3}} \\ $$$${b}\:=\:{lim}_{{x}\rightarrow−{j}} \left({x}+{j}\right){f}\left({x}\right)\:=\:\frac{\mathrm{1}}{\left(−{j}+\mathrm{1}\right)\left(−{j}+\overset{−} {{j}}\right)} \\ $$$$=\:\frac{\mathrm{1}}{\left({j}−\mathrm{1}\right)\left({j}−\overset{−} {{j}}\right)}\:=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}\left(−\frac{\mathrm{3}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)}\: \\ $$$$=\:\frac{\mathrm{2}}{\mathrm{3}\left(\:−\sqrt{\mathrm{3}}\:+{i}\right)}\:=\:\frac{\mathrm{2}\left(\:−\sqrt{\mathrm{3}}−{i}\right)}{\mathrm{3}\left(\mathrm{3}\:+\mathrm{1}\right)}\:=\:\frac{−\sqrt{\mathrm{3}}−{i}}{\mathrm{6}} \\ $$$${c}\:={lim}_{{x}\rightarrow−\overset{−} {{j}}\:} \:\left({x}+\overset{−} {{j}}\right){f}\left({x}\right)\:=\:\frac{\mathrm{1}}{\left(−\overset{−} {{j}}\:+\mathrm{1}\right)\left({j}−\overset{−} {{j}}\right)} \\ $$$$=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}\left(\:\frac{\mathrm{1}+{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:+\mathrm{1}\right)}\:=\:\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}\left(\:\frac{\mathrm{3}}{\mathrm{2}}\:+{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)} \\ $$$$=\:\frac{\mathrm{2}}{\mathrm{3}\left(\sqrt{\mathrm{3}}\:+{i}\right)}\:=\frac{\mathrm{2}\left(\sqrt{\mathrm{3}}−{i}\right)}{\mathrm{3}\left(\mathrm{4}\right)}\:=\:\frac{\sqrt{\mathrm{3}}−{i}}{\mathrm{6}}\:\Rightarrow \\ $$$${f}\left({x}\right)\:=\:\:\frac{\mathrm{1}}{\mathrm{3}\left({x}+\mathrm{1}\right)}\:\:\:−\frac{\sqrt{\mathrm{3}}+{i}}{\mathrm{6}\left({x}+{j}\right)}\:+\frac{\sqrt{\mathrm{3}}−{i}}{\mathrm{6}\left({x}+\overset{−} {{j}}\right)}\:\Rightarrow \\ $$$${f}^{\left({n}\right)} \left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{3}}\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\left({x}+\mathrm{1}\right)^{{n}+\mathrm{1}} }\:−\frac{\sqrt{\mathrm{3}}+{i}}{\mathrm{6}}\:\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\left({x}+{j}\right)^{{n}+\mathrm{1}} } \\ $$$$+\:\frac{\sqrt{\mathrm{3}}−{i}}{\mathrm{6}}\:\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\left({x}+\overset{−} {{j}}\right)^{{n}+\mathrm{1}} } \\ $$
Commented by prof Abdo imad last updated on 27/May/18
f^n (x) = (((−1)^n n!)/(3(x+1)^(n+1) )) + (((−1)^n n!)/6){ (((√3)−i)/((x+j^− )^(n+1) )) −(((√3)+i)/((x+j)^(n+1) ))} f^((n)) (x) = (((−1)^n n!)/(3(x+1)^(n+1) )) +(((−1)^n n!)/6){ ((((√3)−i)/((x+j^− )^(n+1) ))) −conj( (((√3)−i)/((x+j^− )^(n+1) ))) = (((−1)^n n!)/(3(x+1)^(n+1) )) +(((−1)^n n!)/3) Im( (((√3)−i)/((x +j^− )^(n+1) )))
$${f}^{{n}} \left({x}\right)\:=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}\left({x}+\mathrm{1}\right)^{{n}+\mathrm{1}} }\:\:+\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{6}}\left\{\:\:\frac{\sqrt{\mathrm{3}}−{i}}{\left({x}+\overset{−} {{j}}\right)^{{n}+\mathrm{1}} }\right. \\ $$$$\left.−\frac{\sqrt{\mathrm{3}}+{i}}{\left({x}+{j}\right)^{{n}+\mathrm{1}} }\right\} \\ $$$${f}^{\left({n}\right)} \left({x}\right)\:=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}\left({x}+\mathrm{1}\right)^{{n}+\mathrm{1}} }\:+\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{6}}\left\{\:\left(\frac{\sqrt{\mathrm{3}}−{i}}{\left({x}+\overset{−} {{j}}\right)^{{n}+\mathrm{1}} }\right)\right. \\ $$$$−{conj}\left(\:\frac{\sqrt{\mathrm{3}}−{i}}{\left({x}+\overset{−} {{j}}\right)^{{n}+\mathrm{1}} }\right) \\ $$$$=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}\left({x}+\mathrm{1}\right)^{{n}+\mathrm{1}} }\:\:\:+\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}}\:{Im}\left(\:\frac{\sqrt{\mathrm{3}}−{i}}{\left({x}\:+\overset{−} {{j}}\right)^{{n}+\mathrm{1}} }\right) \\ $$$$ \\ $$
Commented by prof Abdo imad last updated on 27/May/18
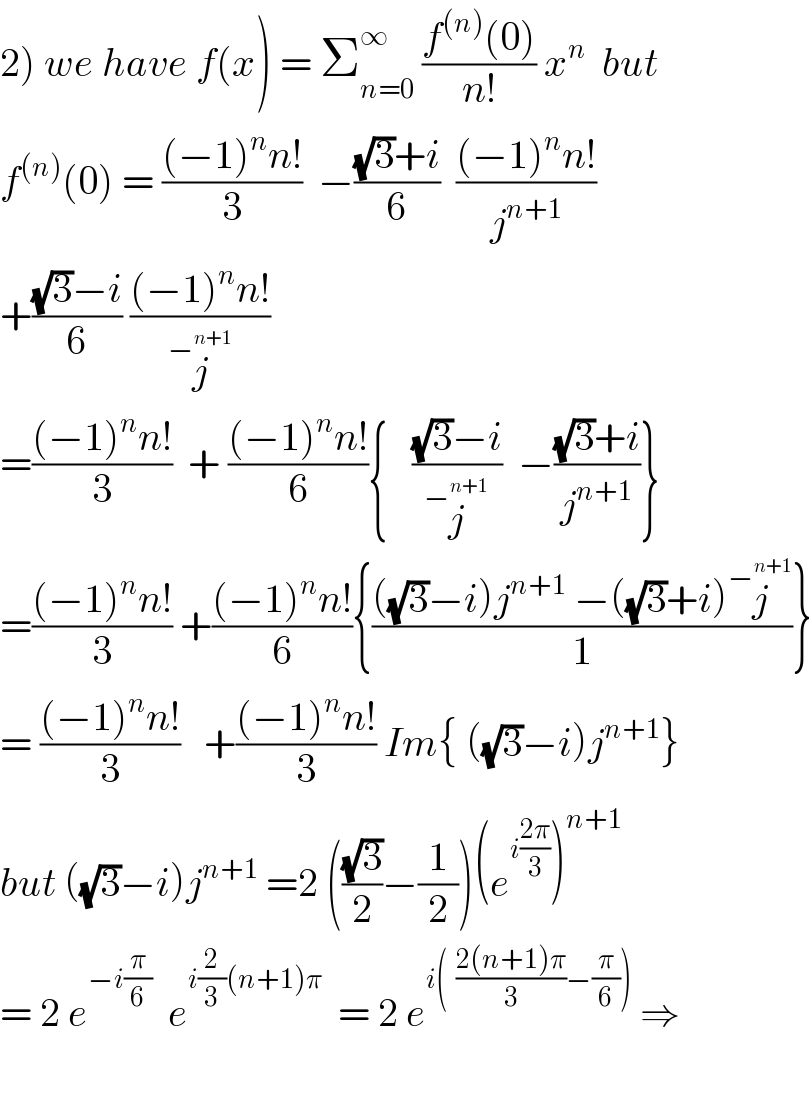
2) we have f(x) = Σ_(n=0) ^∞ ((f^((n)) (0))/(n!)) x^n but f^((n)) (0) = (((−1)^n n!)/3) −(((√3)+i)/6) (((−1)^n n!)/j^(n+1) ) +(((√3)−i)/6) (((−1)^n n!)/j^−^(n+1) ) =(((−1)^n n!)/3) + (((−1)^n n!)/6){ (((√3)−i)/j^−^(n+1) ) −(((√3)+i)/j^(n+1) )} =(((−1)^n n!)/3) +(((−1)^n n!)/6){((((√3)−i)j^(n+1) −((√3)+i)j^−^(n+1) )/1)} = (((−1)^n n!)/3) +(((−1)^n n!)/3) Im{ ((√3)−i)j^(n+1) } but ((√3)−i)j^(n+1) =2 (((√3)/2)−(1/2))(e^(i((2π)/3)) )^(n+1) = 2 e^(−i(π/6)) e^(i(2/3)(n+1)π) = 2 e^(i( ((2(n+1)π)/3)−(π/6))) ⇒
$$\left.\mathrm{2}\right)\:{we}\:{have}\:{f}\left({x}\right)\:=\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{{f}^{\left({n}\right)} \left(\mathrm{0}\right)}{{n}!}\:{x}^{{n}} \:\:{but}\: \\ $$$${f}^{\left({n}\right)} \left(\mathrm{0}\right)\:=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}}\:\:−\frac{\sqrt{\mathrm{3}}+{i}}{\mathrm{6}}\:\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{{j}^{{n}+\mathrm{1}} } \\ $$$$+\frac{\sqrt{\mathrm{3}}−{i}}{\mathrm{6}}\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\overset{−^{{n}+\mathrm{1}} } {{j}}} \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}}\:\:+\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{6}}\left\{\:\:\:\frac{\sqrt{\mathrm{3}}−{i}}{\overset{−^{{n}+\mathrm{1}} } {{j}}}\:\:−\frac{\sqrt{\mathrm{3}}+{i}}{{j}^{{n}+\mathrm{1}} }\right\} \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}}\:+\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{6}}\left\{\frac{\left(\sqrt{\mathrm{3}}−{i}\right){j}^{{n}+\mathrm{1}} \:−\left(\sqrt{\mathrm{3}}+{i}\right)\overset{−^{{n}+\mathrm{1}} } {{j}}}{\mathrm{1}}\right\} \\ $$$$=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}}\:\:\:+\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}}\:{Im}\left\{\:\left(\sqrt{\mathrm{3}}−{i}\right){j}^{{n}+\mathrm{1}} \right\} \\ $$$${but}\:\left(\sqrt{\mathrm{3}}−{i}\right){j}^{{n}+\mathrm{1}} \:=\mathrm{2}\:\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\right)\left({e}^{{i}\frac{\mathrm{2}\pi}{\mathrm{3}}} \right)^{{n}+\mathrm{1}} \\ $$$$=\:\mathrm{2}\:{e}^{−{i}\frac{\pi}{\mathrm{6}}} \:\:{e}^{{i}\frac{\mathrm{2}}{\mathrm{3}}\left({n}+\mathrm{1}\right)\pi} \:\:=\:\mathrm{2}\:{e}^{{i}\left(\:\:\frac{\mathrm{2}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}−\frac{\pi}{\mathrm{6}}\right)} \:\Rightarrow \\ $$$$ \\ $$
Commented by abdo.msup.com last updated on 27/May/18
f^((n)) (0) = (((−1)^n n!)/3) +(2/3)(−1)^n n!sin(((2(n+1)π)/3)−(π/6)) ⇒f(x) =Σ_(n=0) ^∞ ((f^((n)) (0))/(n!))x^n =Σ_(n=0) ^∞ (((−1)^n )/3){ 1 +2sin(((2(n+1)π)/3) −(π/6))}x^n another method we have f(x) =(1/(1+x^3 )) so for ∣x∣<1 f(x) =Σ_(n=0) ^∞ (−1)^n x^(3n) .
$${f}^{\left({n}\right)} \left(\mathrm{0}\right)\:=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{3}}\:+\frac{\mathrm{2}}{\mathrm{3}}\left(−\mathrm{1}\right)^{{n}} {n}!{sin}\left(\frac{\mathrm{2}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}−\frac{\pi}{\mathrm{6}}\right) \\ $$$$\Rightarrow{f}\left({x}\right)\:=\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{{f}^{\left({n}\right)} \left(\mathrm{0}\right)}{{n}!}{x}^{{n}} \\ $$$$=\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{3}}\left\{\:\mathrm{1}\:+\mathrm{2}{sin}\left(\frac{\mathrm{2}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}\:−\frac{\pi}{\mathrm{6}}\right)\right\}{x}^{{n}} \\ $$$${another}\:{method}\: \\ $$$${we}\:{have}\:{f}\left({x}\right)\:=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{3}} }\:{so}\:{for}\:\mid{x}\mid<\mathrm{1} \\ $$$${f}\left({x}\right)\:=\sum_{{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{{n}} \:{x}^{\mathrm{3}{n}} \:. \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *