Question Number 53212 by Joel578 last updated on 19/Jan/19
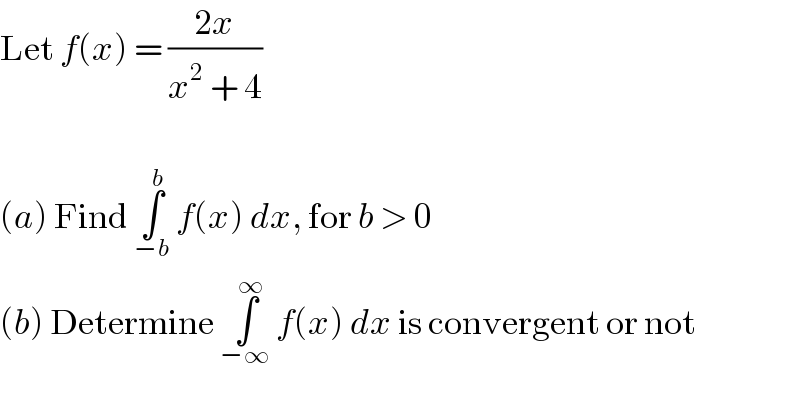
Commented by Joel578 last updated on 19/Jan/19
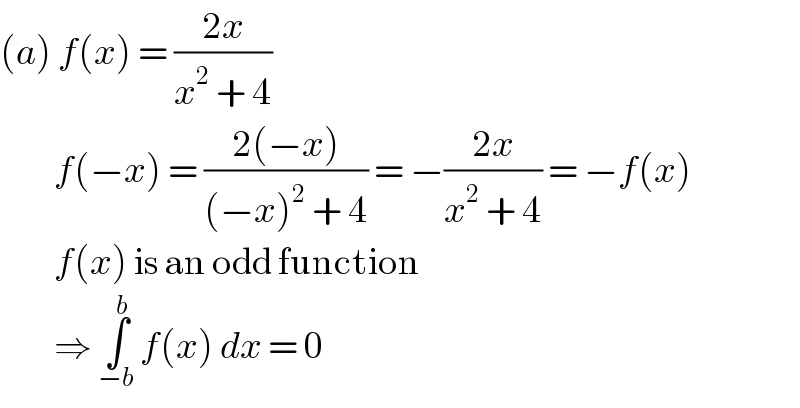
Commented by Joel578 last updated on 19/Jan/19
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented by afachri last updated on 19/Jan/19
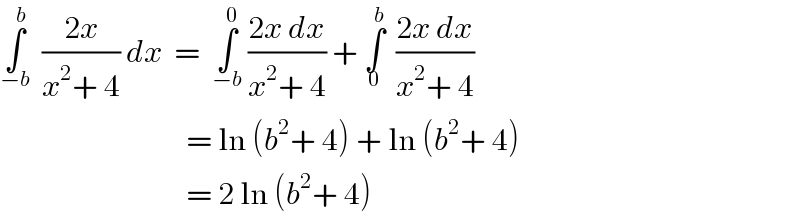
Commented by afachri last updated on 19/Jan/19
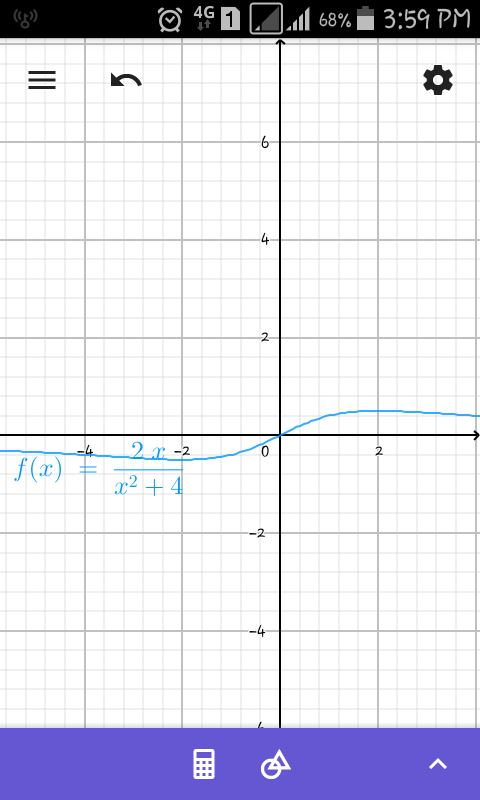
Commented by afachri last updated on 19/Jan/19
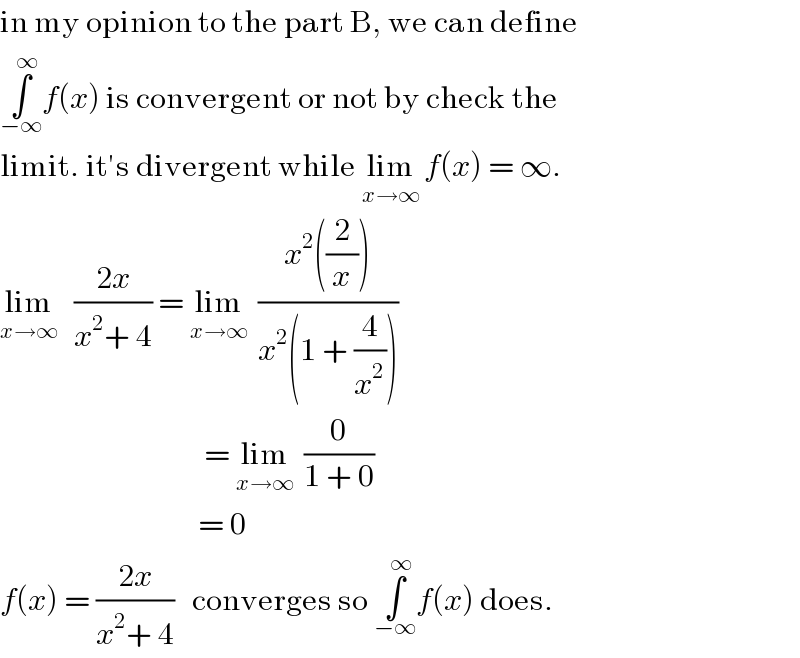
Commented by afachri last updated on 19/Jan/19
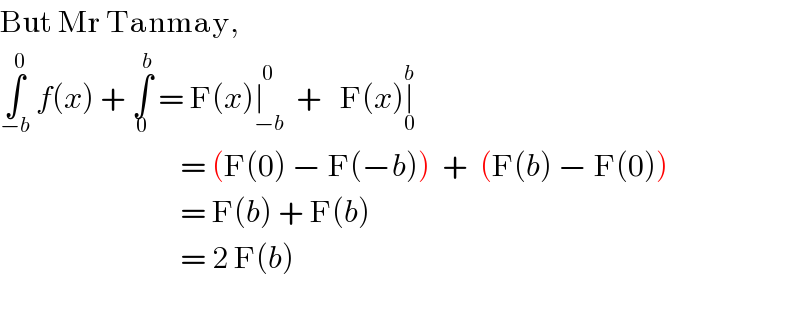
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19
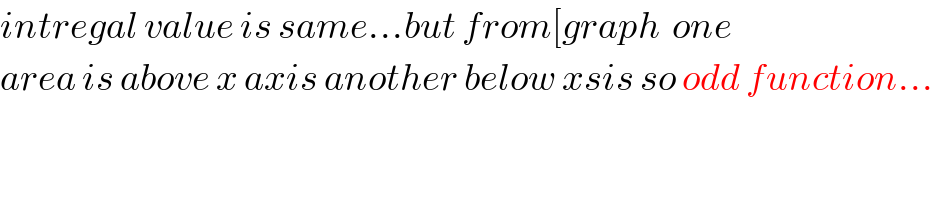
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19
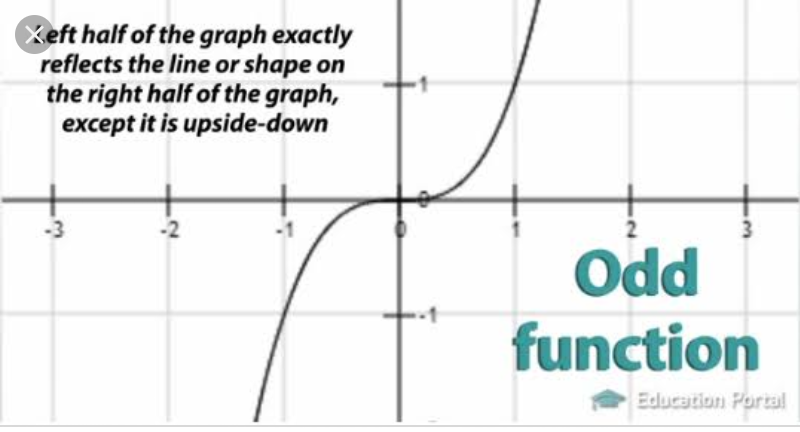
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented by afachri last updated on 19/Jan/19

Commented by afachri last updated on 19/Jan/19
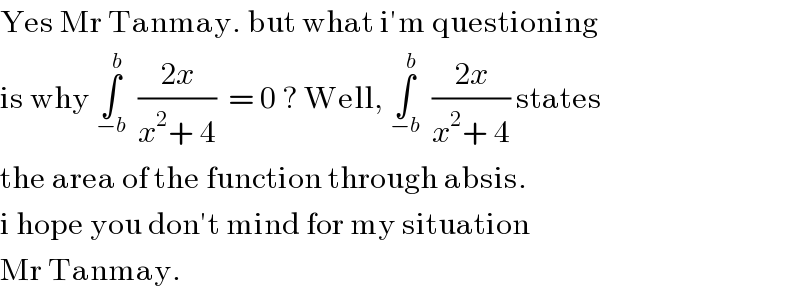
Commented by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19

Commented by afachri last updated on 19/Jan/19

Commented by afachri last updated on 19/Jan/19

Commented by JDamian last updated on 19/Jan/19
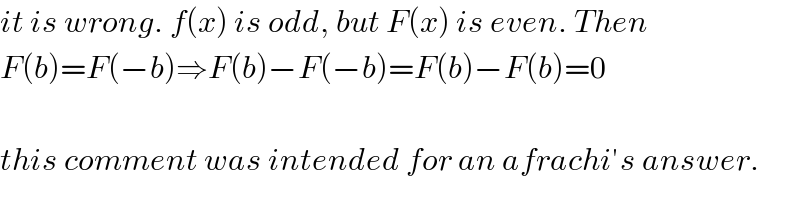
Commented by Joel578 last updated on 20/Jan/19
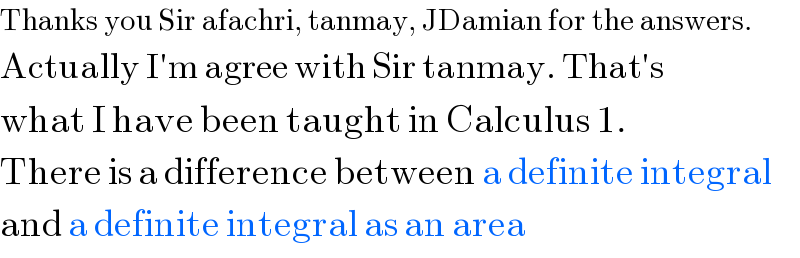
Commented by maxmathsup by imad last updated on 23/Jan/19
![for all odd function integrable on ]−a,a[ a∈R^− we have ∫_(−a) ^a f(x)dx =0.](https://www.tinkutara.com/question/Q53609.png)
Answered by MJS last updated on 20/Jan/19
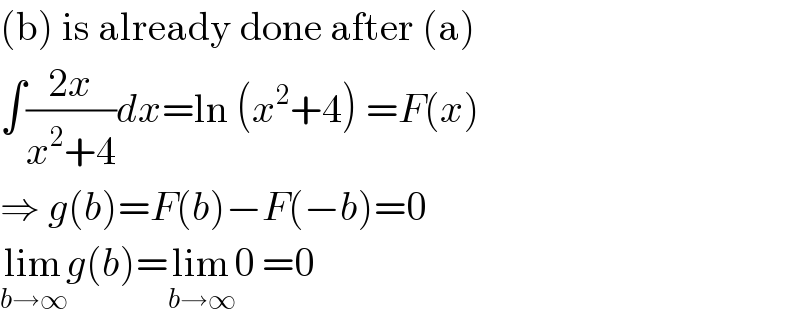
Commented by Joel578 last updated on 20/Jan/19
Answered by tanmay.chaudhury50@gmail.com last updated on 19/Jan/19
![b) it is my opinion... ∫_(−∞) ^(+∞) f(x)dx lim_(h→−∞) ∫_h ^0 f(x) dx=−ve A lim_(k→+∞) ∫_0 ^k f(x)dx=+ve A so ∫_(−∞) ^(+∞) ((2x)/(4+x^2 ))dx=0 anoother way... x=2tanθ dx=2sec^2 θdθ ∫_(−(π/2)) ^(π/2) ((2×2tanθ×2sec^2 θdθ)/(4+4tan^2 θ)) =∫_(−(π/2)) ^(π/2) ((8tanθsec^2 θ)/(4sec^2 θ))dθ =2∣lnsecθ∣_(−(π/2)) ^(π/2) =−2∣lncosθ∣_(−(π/2)) ^(π/2) =−2[lncos((π/2))−lncos(−(π/2))] =0 it is my opinion...let others comment...](https://www.tinkutara.com/question/Q53231.png)
Commented by Joel578 last updated on 20/Jan/19

