Let-f-x-x-1-2-for-all-x-1-If-g-x-is-the-function-whose-graph-is-the-reflection-of-the-graph-of-f-x-with-respect-to-the-line-y-x-then-g-x-is-equal-to-

Question Number 129622 by Ar Brandon last updated on 16/Jan/21

Let f (x) = {(x + 1)}^{2} for all x ⩾ - 1 . If g (x) is the

function whose graph is the reflection of the graph

of f (x) with respect to the line y = x, then g (x) is

equal to \dots

Commented by mr W last updated on 16/Jan/21

g (x) = f^{- 1} (x) = \sqrt{x} - 1

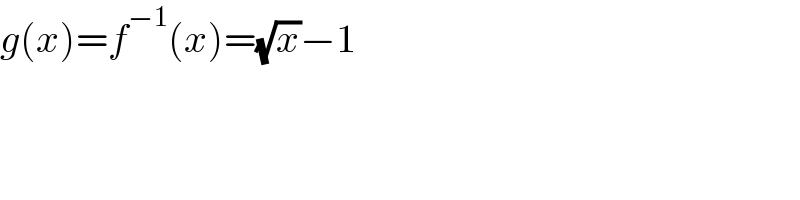
Commented by Ar Brandon last updated on 17/Jan/21

Thanks Sir

Answered by bemath last updated on 17/Jan/21

(\begin{matrix} x^{'} \\ y^{'} \end{matrix}) = (\begin{matrix} 0 1 \\ 1 0 \end{matrix}) (\begin{matrix} x \\ y \end{matrix}) \Rightarrow (\begin{matrix} x \\ y \end{matrix}) = {(\begin{matrix} 0 1 \\ 1 0 \end{matrix})}^{- 1} (\begin{matrix} x^{'} \\ y^{'} \end{matrix})

(\begin{matrix} x \\ y \end{matrix}) = \frac{1}{0 - 1} (\begin{matrix} 0 - 1 \\ - 1 0 \end{matrix}) (\begin{matrix} x^{'} \\ y^{'} \end{matrix})

(\begin{matrix} x \\ y \end{matrix}) = (\begin{matrix} 0 1 \\ 1 0 \end{matrix}) (\begin{matrix} x^{'} \\ y^{'} \end{matrix}) = (\begin{matrix} y^{'} \\ x^{'} \end{matrix})

f (x) = {(x + 1)}^{2} \Rightarrow y = {(x + 1)}^{2}

x^{'} = {(y^{'} + 1)}^{2}; y + 1 = \sqrt{x}; y = \sqrt{x} - 1

g (x) = \sqrt{x} - 1 .

Commented by Ar Brandon last updated on 17/Jan/21

Thanks bro