Question Number 98428 by mathmax by abdo last updated on 13/Jun/20
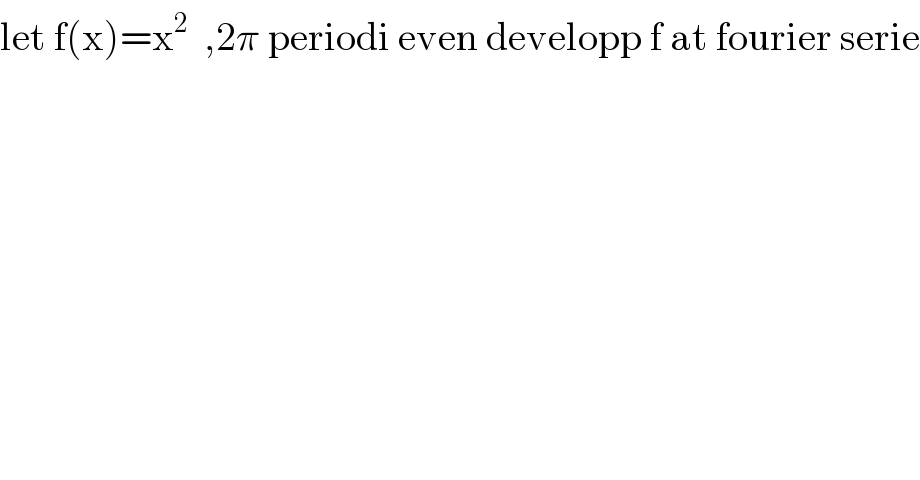
$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} \:\:,\mathrm{2}\pi\:\mathrm{periodi}\:\mathrm{even}\:\mathrm{developp}\:\mathrm{f}\:\mathrm{at}\:\mathrm{fourier}\:\mathrm{serie} \\ $$
Answered by mathmax by abdo last updated on 14/Jun/20
![f is even ⇒f(x) =(a_0 /2) +Σ_(n=1) ^∞ a_n cos(nx) with a_n =(2/T) ∫_([T]) x^2 cos(nx)dx =(2/(2π)) ∫_(−π) ^π x^2 cos(nx)dx =(2/π) ∫_0 ^π x^2 cos(nx)dx ⇒(π/2)a_n =∫_0 ^π x^2 cos(nx)dx =_(by parts) [(x^2 /n)sin(nx)]_0 ^π −∫_0 ^π 2x×((sin(nx))/n)dx =−(2/n) ∫_0 ^π xsin(nx)dx =−(2/n){ [−(x/n)cos(nx)]_0 ^π +(1/n)∫_0 ^π cos(nx)dx} =−(2/n){ −(π/n)(−1)^n +(1/n^2 )[sin(nx)]_0 ^π } =((2π(−1)^n )/n^2 ) ⇒(π/2)a_n =((2π(−1)^n )/n^2 ) ⇒ a_n =((4(−1)^n )/n^2 ) we have a_0 =(2/π) ∫_0 ^π x^2 dx =(2/π)[(1/3)x^3 ]_0 ^π =(2/(3π))×π^3 =((2π^2 )/3) ⇒ x^2 =(π^2 /3) +Σ_(n=1) ^∞ ((4(−1)^n )/n^2 )cos(nx) ⇒x^2 =(π^2 /3) +4 Σ_(n=1) ^∞ (((−1)^n )/n^2 )cos(nx)](https://www.tinkutara.com/question/Q98536.png)
$$\mathrm{f}\:\mathrm{is}\:\mathrm{even}\:\Rightarrow\mathrm{f}\left(\mathrm{x}\right)\:=\frac{\mathrm{a}_{\mathrm{0}} }{\mathrm{2}}\:+\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\mathrm{a}_{\mathrm{n}} \:\mathrm{cos}\left(\mathrm{nx}\right)\:\mathrm{with} \\ $$$$\mathrm{a}_{\mathrm{n}} =\frac{\mathrm{2}}{\mathrm{T}}\:\int_{\left[\mathrm{T}\right]} \:\:\mathrm{x}^{\mathrm{2}} \:\mathrm{cos}\left(\mathrm{nx}\right)\mathrm{dx}\:=\frac{\mathrm{2}}{\mathrm{2}\pi}\:\int_{−\pi} ^{\pi} \:\mathrm{x}^{\mathrm{2}} \:\mathrm{cos}\left(\mathrm{nx}\right)\mathrm{dx} \\ $$$$=\frac{\mathrm{2}}{\pi}\:\int_{\mathrm{0}} ^{\pi} \:\mathrm{x}^{\mathrm{2}} \:\mathrm{cos}\left(\mathrm{nx}\right)\mathrm{dx}\:\Rightarrow\frac{\pi}{\mathrm{2}}\mathrm{a}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\pi} \:\mathrm{x}^{\mathrm{2}} \:\mathrm{cos}\left(\mathrm{nx}\right)\mathrm{dx}\: \\ $$$$=_{\mathrm{by}\:\mathrm{parts}} \:\:\:\left[\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{n}}\mathrm{sin}\left(\mathrm{nx}\right)\right]_{\mathrm{0}} ^{\pi} \:−\int_{\mathrm{0}} ^{\pi} \:\mathrm{2x}×\frac{\mathrm{sin}\left(\mathrm{nx}\right)}{\mathrm{n}}\mathrm{dx} \\ $$$$=−\frac{\mathrm{2}}{\mathrm{n}}\:\int_{\mathrm{0}} ^{\pi} \mathrm{xsin}\left(\mathrm{nx}\right)\mathrm{dx}\:=−\frac{\mathrm{2}}{\mathrm{n}}\left\{\:\left[−\frac{\mathrm{x}}{\mathrm{n}}\mathrm{cos}\left(\mathrm{nx}\right)\right]_{\mathrm{0}} ^{\pi} \:+\frac{\mathrm{1}}{\mathrm{n}}\int_{\mathrm{0}} ^{\pi} \:\mathrm{cos}\left(\mathrm{nx}\right)\mathrm{dx}\right\} \\ $$$$=−\frac{\mathrm{2}}{\mathrm{n}}\left\{\:−\frac{\pi}{\mathrm{n}}\left(−\mathrm{1}\right)^{\mathrm{n}} \:+\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} }\left[\mathrm{sin}\left(\mathrm{nx}\right)\right]_{\mathrm{0}} ^{\pi} \right\}\:=\frac{\mathrm{2}\pi\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{n}^{\mathrm{2}} }\:\Rightarrow\frac{\pi}{\mathrm{2}}\mathrm{a}_{\mathrm{n}} =\frac{\mathrm{2}\pi\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{n}^{\mathrm{2}} }\:\Rightarrow \\ $$$$\mathrm{a}_{\mathrm{n}} =\frac{\mathrm{4}\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{n}^{\mathrm{2}} }\:\:\mathrm{we}\:\mathrm{have}\:\mathrm{a}_{\mathrm{0}} =\frac{\mathrm{2}}{\pi}\:\int_{\mathrm{0}} ^{\pi} \:\mathrm{x}^{\mathrm{2}} \:\mathrm{dx}\:=\frac{\mathrm{2}}{\pi}\left[\frac{\mathrm{1}}{\mathrm{3}}\mathrm{x}^{\mathrm{3}} \right]_{\mathrm{0}} ^{\pi} \:=\frac{\mathrm{2}}{\mathrm{3}\pi}×\pi^{\mathrm{3}} \:=\frac{\mathrm{2}\pi^{\mathrm{2}} }{\mathrm{3}}\:\Rightarrow \\ $$$$\mathrm{x}^{\mathrm{2}} \:=\frac{\pi^{\mathrm{2}} }{\mathrm{3}}\:+\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{4}\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{n}^{\mathrm{2}} }\mathrm{cos}\left(\mathrm{nx}\right)\:\Rightarrow\mathrm{x}^{\mathrm{2}} \:=\frac{\pi^{\mathrm{2}} }{\mathrm{3}}\:+\mathrm{4}\:\sum_{\mathrm{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{n}^{\mathrm{2}} }\mathrm{cos}\left(\mathrm{nx}\right) \\ $$
