Question Number 42688 by prof Abdo imad last updated on 31/Aug/18
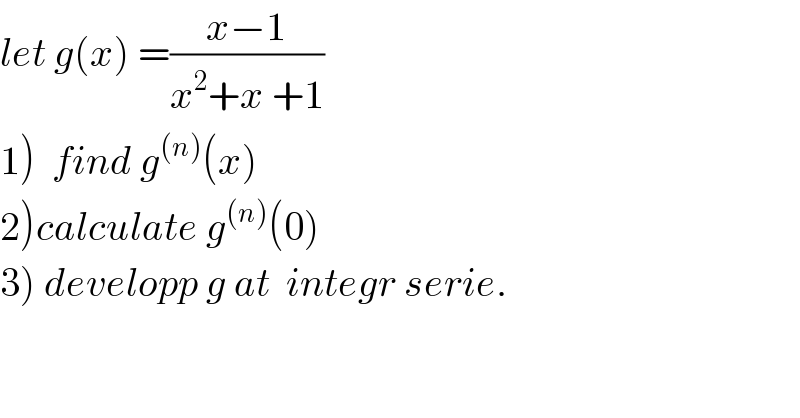
Commented by maxmathsup by imad last updated on 02/Sep/18

Commented by maxmathsup by imad last updated on 02/Sep/18

Commented by maxmathsup by imad last updated on 02/Sep/18

