Question Number 35438 by prof Abdo imad last updated on 19/May/18
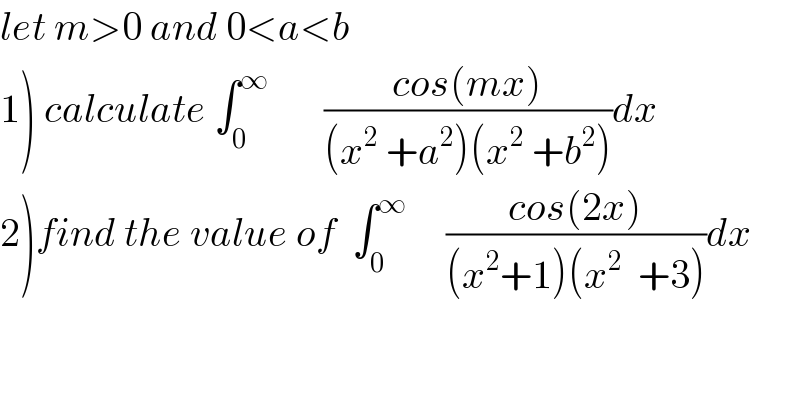
$${let}\:{m}>\mathrm{0}\:{and}\:\mathrm{0}<{a}<{b}\: \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\:\:\frac{{cos}\left({mx}\right)}{\left({x}^{\mathrm{2}} \:+{a}^{\mathrm{2}} \right)\left({x}^{\mathrm{2}} \:+{b}^{\mathrm{2}} \right)}{dx} \\ $$$$\left.\mathrm{2}\right){find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\frac{{cos}\left(\mathrm{2}{x}\right)}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}^{\mathrm{2}} \:\:+\mathrm{3}\right)}{dx} \\ $$
Commented by prof Abdo imad last updated on 21/May/18
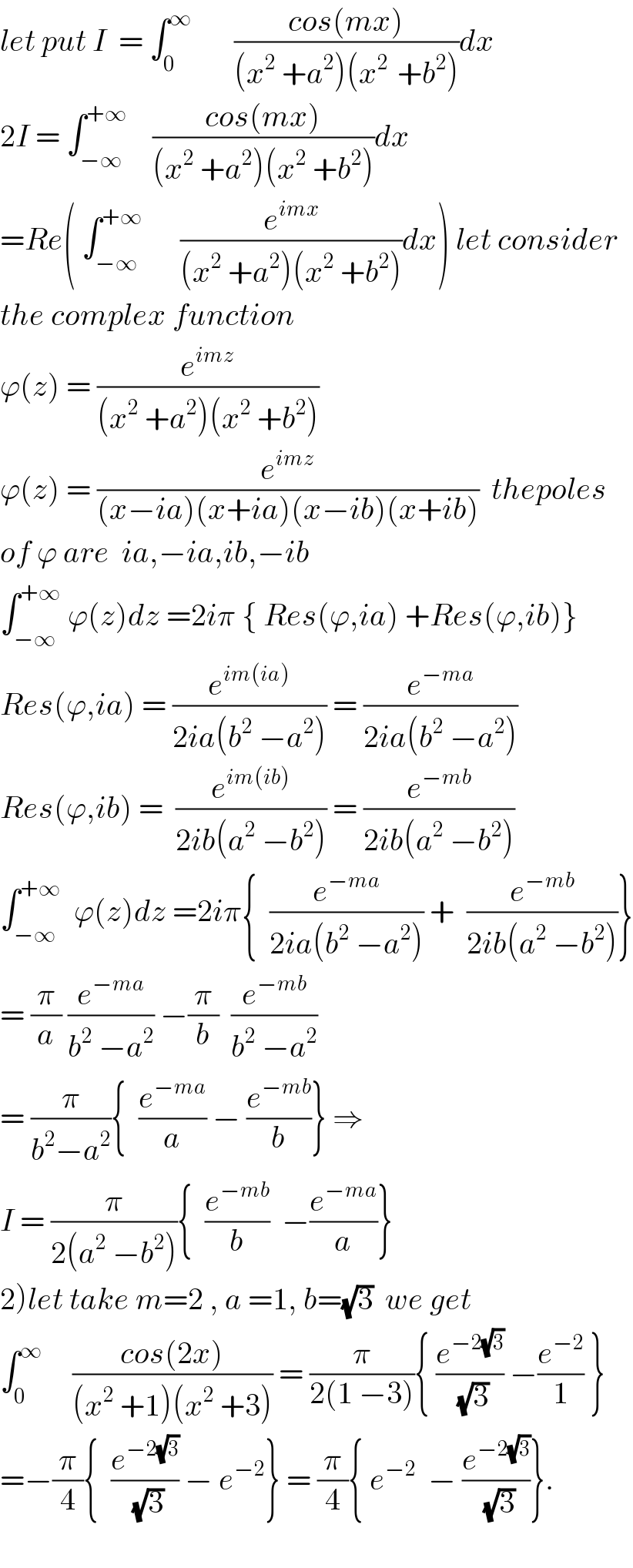
$${let}\:{put}\:{I}\:\:=\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\:\:\frac{{cos}\left({mx}\right)}{\left({x}^{\mathrm{2}} \:+{a}^{\mathrm{2}} \right)\left({x}^{\mathrm{2}\:} \:+{b}^{\mathrm{2}} \right)}{dx} \\ $$$$\mathrm{2}{I}\:=\:\int_{−\infty} ^{+\infty} \:\:\:\:\frac{{cos}\left({mx}\right)}{\left({x}^{\mathrm{2}} \:+{a}^{\mathrm{2}} \right)\left({x}^{\mathrm{2}} \:+{b}^{\mathrm{2}} \right)}{dx} \\ $$$$={Re}\left(\:\int_{−\infty} ^{+\infty} \:\:\:\:\:\:\frac{{e}^{{imx}} }{\left({x}^{\mathrm{2}} \:+{a}^{\mathrm{2}} \right)\left({x}^{\mathrm{2}} \:+{b}^{\mathrm{2}} \right)}{dx}\right)\:{let}\:{consider} \\ $$$${the}\:{complex}\:{function} \\ $$$$\varphi\left({z}\right)\:=\:\frac{{e}^{{imz}} }{\left({x}^{\mathrm{2}} \:+{a}^{\mathrm{2}} \right)\left({x}^{\mathrm{2}} \:+{b}^{\mathrm{2}} \right)} \\ $$$$\varphi\left({z}\right)\:=\:\frac{{e}^{{imz}} }{\left({x}−{ia}\right)\left({x}+{ia}\right)\left({x}−{ib}\right)\left({x}+{ib}\right)}\:\:{thepoles} \\ $$$${of}\:\varphi\:{are}\:\:{ia},−{ia},{ib},−{ib} \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:\left\{\:{Res}\left(\varphi,{ia}\right)\:+{Res}\left(\varphi,{ib}\right)\right\} \\ $$$${Res}\left(\varphi,{ia}\right)\:=\:\frac{{e}^{{im}\left({ia}\right)} }{\mathrm{2}{ia}\left({b}^{\mathrm{2}} \:−{a}^{\mathrm{2}} \right)}\:=\:\frac{{e}^{−{ma}} }{\mathrm{2}{ia}\left({b}^{\mathrm{2}} \:−{a}^{\mathrm{2}} \right)} \\ $$$${Res}\left(\varphi,{ib}\right)\:=\:\:\frac{{e}^{{im}\left({ib}\right)} }{\mathrm{2}{ib}\left({a}^{\mathrm{2}} \:−{b}^{\mathrm{2}} \right)}\:=\:\frac{{e}^{−{mb}} }{\mathrm{2}{ib}\left({a}^{\mathrm{2}} \:−{b}^{\mathrm{2}} \right)} \\ $$$$\int_{−\infty} ^{+\infty} \:\:\varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\left\{\:\:\frac{{e}^{−{ma}} }{\mathrm{2}{ia}\left({b}^{\mathrm{2}} \:−{a}^{\mathrm{2}} \right)}\:+\:\:\frac{{e}^{−{mb}} }{\mathrm{2}{ib}\left({a}^{\mathrm{2}} \:−{b}^{\mathrm{2}} \right)}\right\} \\ $$$$=\:\frac{\pi}{{a}}\:\frac{{e}^{−{ma}} }{{b}^{\mathrm{2}} \:−{a}^{\mathrm{2}} }\:−\frac{\pi}{{b}}\:\:\frac{{e}^{−{mb}} }{{b}^{\mathrm{2}} \:−{a}^{\mathrm{2}} } \\ $$$$=\:\frac{\pi}{{b}^{\mathrm{2}} −{a}^{\mathrm{2}} }\left\{\:\:\frac{{e}^{−{ma}} }{{a}}\:−\:\frac{{e}^{−{mb}} }{{b}}\right\}\:\Rightarrow \\ $$$${I}\:=\:\frac{\pi}{\mathrm{2}\left({a}^{\mathrm{2}} \:−{b}^{\mathrm{2}} \right)}\left\{\:\:\frac{{e}^{−{mb}} }{{b}}\:\:−\frac{{e}^{−{ma}} }{{a}}\right\} \\ $$$$\left.\mathrm{2}\right){let}\:{take}\:{m}=\mathrm{2}\:,\:{a}\:=\mathrm{1},\:{b}=\sqrt{\mathrm{3}}\:\:{we}\:{get} \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\frac{{cos}\left(\mathrm{2}{x}\right)}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\left({x}^{\mathrm{2}} \:+\mathrm{3}\right)}\:=\:\frac{\pi}{\mathrm{2}\left(\mathrm{1}\:−\mathrm{3}\right)}\left\{\:\frac{{e}^{−\mathrm{2}\sqrt{\mathrm{3}}} }{\:\sqrt{\mathrm{3}}}\:−\frac{{e}^{−\mathrm{2}} }{\mathrm{1}}\:\right\} \\ $$$$=−\frac{\pi}{\mathrm{4}}\left\{\:\:\frac{{e}^{−\mathrm{2}\sqrt{\mathrm{3}}} }{\:\sqrt{\mathrm{3}}}\:−\:{e}^{−\mathrm{2}} \right\}\:=\:\frac{\pi}{\mathrm{4}}\left\{\:{e}^{−\mathrm{2}} \:\:−\:\frac{{e}^{−\mathrm{2}\sqrt{\mathrm{3}}} }{\:\sqrt{\mathrm{3}}}\right\}. \\ $$$$ \\ $$
