Let-P-be-an-interior-point-of-a-triangle-ABC-and-AP-BP-CP-meet-the-sides-BC-CA-AB-in-D-E-F-respectively-Show-that-AP-PD-AF-FB-AE-EC-

Question Number 42196 by rahul 19 last updated on 20/Aug/18

Let P be an interior point of a triangle

ABC and AP, BP, CP meet the sides BC,

CA, AB in D, E, F respectively . Show

that \frac{AP}{PD} = \frac{AF}{FB} + \frac{AE}{EC} .

Commented by rahul 19 last updated on 20/Aug/18

Again do by concept of vectors only!

Commented by ajfour last updated on 20/Aug/18

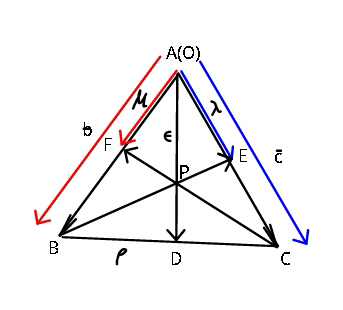
Commented by ajfour last updated on 21/Aug/18

t o p r o v e \frac{A F}{F B} + \frac{A E}{E C} = \frac{A P}{P D}

l e t A F = μ \bar{b}; A E = λ \bar{c}

A P = ϵ A D

\Rightarrow T o p r o v e : \frac{μ}{1 - μ} + \frac{λ}{1 - λ} = \frac{ϵ}{1 - ϵ}

\bar{p} = \bar{b} + l (λ \bar{c} - \bar{b})

= \bar{c} + m (μ \bar{b} - \bar{c})

= ϵ [\bar{b} + ρ (\bar{c} - \bar{b})]

\Rightarrow 1 - l = μ m = ϵ - ϵ ρ \dots (i)

& λ l = 1 - m = ϵ ρ \dots . (i i)

\Rightarrow 1 - \frac{ϵ ρ}{λ} = μ (1 - ϵ ρ) = ϵ - ϵ ρ

\Rightarrow ϵ ρ = \frac{1 - μ}{\frac{1}{λ} - μ} = \frac{1 - ϵ}{\frac{1}{λ} - 1}

\Rightarrow \frac{1}{λ} - 1 - \frac{μ}{λ} + μ = \frac{1}{λ} - μ - \frac{ϵ}{λ} + ϵ μ

\Rightarrow λ + μ - λ μ = λ μ + ϵ - λ ϵ μ

\Rightarrow ϵ = \frac{λ + μ - 2 λ μ}{1 - λ μ}

\frac{ϵ}{1 - ϵ} = \frac{λ + μ - 2 λ μ}{1 - λ - μ + λ μ}

= \frac{μ (1 - λ) + λ (1 - μ)}{(1 - λ) (1 - μ)}

\Rightarrow \frac{\boldsymbol ϵ}{1 - \boldsymbol ϵ} = \frac{\boldsymbol μ}{1 - \boldsymbol μ} + \frac{\boldsymbol λ}{1 - \boldsymbol λ} .

Commented by rahul 19 last updated on 21/Aug/18

thank you sir!�� I will see later .