Question Number 175624 by infinityaction last updated on 04/Sep/22
$$\mathrm{let}\:\mathrm{p}\left(\mathrm{x}\right)\:=\:\mathrm{x}^{\mathrm{6}} +\mathrm{ax}^{\mathrm{5}} +\mathrm{bx}^{\mathrm{4}} +\mathrm{cx}^{\mathrm{3}} +\mathrm{dx}^{\mathrm{2}} +\mathrm{ex}+\mathrm{f} \\ $$$$\:\:\mathrm{be}\:\mathrm{a}\:\mathrm{polynomial}\:\mathrm{function}\:\mathrm{such} \\ $$$$\:\:\mathrm{that}\:\:\mathrm{p}\left(\mathrm{1}\right)\:=\:\mathrm{1}\:;\:\mathrm{p}\left(\mathrm{2}\right)\:=\:\mathrm{2}\:;\:\:\mathrm{p}\left(\mathrm{3}\right)\:=\:\mathrm{3} \\ $$$$\:\:\mathrm{p}\left(\mathrm{4}\right)\:=\:\mathrm{4}\:;\:\mathrm{p}\left(\mathrm{5}\right)\:=\:\mathrm{5}\:;\:\mathrm{p}\left(\mathrm{6}\right)\:=\:\mathrm{6}\:\:\mathrm{then} \\ $$$$\:\:\mathrm{find}\:\:\mathrm{p}\left(\mathrm{7}\right)\:=\:? \\ $$
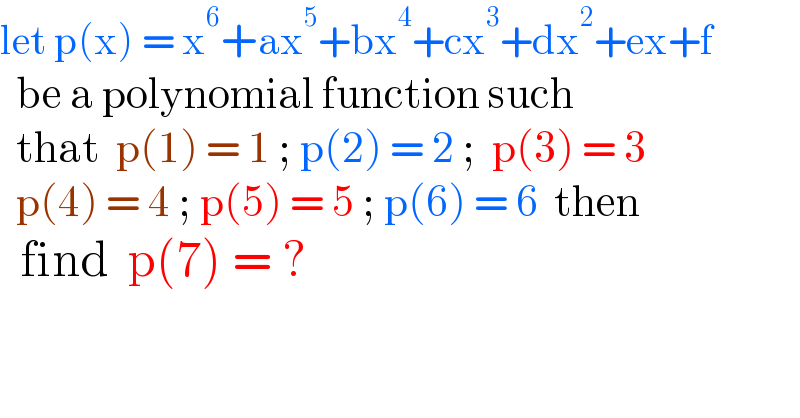
Answered by cortano1 last updated on 04/Sep/22
$$\mathrm{p}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{1}\right)\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{x}−\mathrm{3}\right)\left(\mathrm{x}−\mathrm{4}\right)\left(\mathrm{x}−\mathrm{5}\right)\left(\mathrm{x}−\mathrm{6}\right)+\mathrm{x} \\ $$$$\mathrm{p}\left(\mathrm{7}\right)=\mathrm{6}.\mathrm{5}.\mathrm{4}.\mathrm{3}.\mathrm{2}.\mathrm{1}+\mathrm{7}=\mathrm{6}!+\mathrm{7} \\ $$$$\:\:\:\:\:\:\:\:\:\:=\:\mathrm{727} \\ $$
Commented by BaliramKumar last updated on 05/Sep/22
![p(n) = ? [ n > 6 ]](https://www.tinkutara.com/question/Q175640.png)
$$\mathrm{p}\left(\mathrm{n}\right)\:=\:?\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left[\:{n}\:>\:\mathrm{6}\:\right] \\ $$
Answered by leodera last updated on 04/Sep/22
$$ \\ $$$${p}\left({x}\right)\:=\:\left({x}−\mathrm{1}\right)\left({x}−\mathrm{2}\right)\left({x}−\mathrm{3}\right)\left({x}−\mathrm{4}\right)\left({x}−\mathrm{5}\right)\left(\boldsymbol{{x}}−\mathrm{6}\right)+\boldsymbol{{x}} \\ $$$$\boldsymbol{{p}}\left(\mathrm{7}\right)\:=\:\mathrm{6}!\:+\:\mathrm{7}\:=\:\mathrm{720}+\mathrm{7}\:=\:\mathrm{727} \\ $$
Answered by floor(10²Eta[1]) last updated on 04/Sep/22
![p(1)=1⇒p(x)=(x−1)a(x)+1 p(2)=a(2)+1=2⇒a(2)=1⇒a(x)=(x−2)b(x)+1 p(x)=(x−1)((x−2)b(x)+1)+1 p(3)=2(b(3)+1)+1=3⇒b(3)=0⇒b(x)=(x−3)c(x) p(x)=(x−1)((x−2)(x−3)c(x)+1)+1 p(4)=3(2c(4)+1)+1=4⇒c(4)=0⇒c(x)=(x−4)d(x) ⇒p(x)=(x−1)[(x−2)(x−3)(x−4)d(x)+1)+1 p(5)=4[3.2d(5)+1]+1=5⇒d(5)=0⇒d(x)=(x−5)e(x) p(x)=(x−1)[(x−2)(x−3)(x−4)(x−5)e(x)+1]+1 p(6)=5[4.3.2e(6)+1]+1=6⇒e(6)=0⇒e(x)=(x−6)C ⇒p(x)=(x−1)[(x−2)(x−3)(x−4)(x−5)(x−6)C+1]+1 =(x−1)(x−2)(x−3)(x−4)(x−5)(x−6)C+x p(7)=6!C+7=720C+7, C∈R^∗ but C=1 because p(x)=x^6 +ax^5 +... ⇒p(7)=727](https://www.tinkutara.com/question/Q175643.png)
$$\mathrm{p}\left(\mathrm{1}\right)=\mathrm{1}\Rightarrow\mathrm{p}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{1}\right)\mathrm{a}\left(\mathrm{x}\right)+\mathrm{1} \\ $$$$\mathrm{p}\left(\mathrm{2}\right)=\mathrm{a}\left(\mathrm{2}\right)+\mathrm{1}=\mathrm{2}\Rightarrow\mathrm{a}\left(\mathrm{2}\right)=\mathrm{1}\Rightarrow\mathrm{a}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{2}\right)\mathrm{b}\left(\mathrm{x}\right)+\mathrm{1} \\ $$$$\mathrm{p}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{1}\right)\left(\left(\mathrm{x}−\mathrm{2}\right)\mathrm{b}\left(\mathrm{x}\right)+\mathrm{1}\right)+\mathrm{1} \\ $$$$\mathrm{p}\left(\mathrm{3}\right)=\mathrm{2}\left(\mathrm{b}\left(\mathrm{3}\right)+\mathrm{1}\right)+\mathrm{1}=\mathrm{3}\Rightarrow\mathrm{b}\left(\mathrm{3}\right)=\mathrm{0}\Rightarrow\mathrm{b}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{3}\right)\mathrm{c}\left(\mathrm{x}\right) \\ $$$$\mathrm{p}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{1}\right)\left(\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{x}−\mathrm{3}\right)\mathrm{c}\left(\mathrm{x}\right)+\mathrm{1}\right)+\mathrm{1} \\ $$$$\mathrm{p}\left(\mathrm{4}\right)=\mathrm{3}\left(\mathrm{2c}\left(\mathrm{4}\right)+\mathrm{1}\right)+\mathrm{1}=\mathrm{4}\Rightarrow\mathrm{c}\left(\mathrm{4}\right)=\mathrm{0}\Rightarrow\mathrm{c}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{4}\right)\mathrm{d}\left(\mathrm{x}\right) \\ $$$$\Rightarrow\mathrm{p}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{1}\right)\left[\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{x}−\mathrm{3}\right)\left(\mathrm{x}−\mathrm{4}\right)\mathrm{d}\left(\mathrm{x}\right)+\mathrm{1}\right)+\mathrm{1} \\ $$$$\mathrm{p}\left(\mathrm{5}\right)=\mathrm{4}\left[\mathrm{3}.\mathrm{2d}\left(\mathrm{5}\right)+\mathrm{1}\right]+\mathrm{1}=\mathrm{5}\Rightarrow\mathrm{d}\left(\mathrm{5}\right)=\mathrm{0}\Rightarrow\mathrm{d}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{5}\right)\mathrm{e}\left(\mathrm{x}\right) \\ $$$$\mathrm{p}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{1}\right)\left[\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{x}−\mathrm{3}\right)\left(\mathrm{x}−\mathrm{4}\right)\left(\mathrm{x}−\mathrm{5}\right)\mathrm{e}\left(\mathrm{x}\right)+\mathrm{1}\right]+\mathrm{1} \\ $$$$\mathrm{p}\left(\mathrm{6}\right)=\mathrm{5}\left[\mathrm{4}.\mathrm{3}.\mathrm{2e}\left(\mathrm{6}\right)+\mathrm{1}\right]+\mathrm{1}=\mathrm{6}\Rightarrow\mathrm{e}\left(\mathrm{6}\right)=\mathrm{0}\Rightarrow\mathrm{e}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{6}\right)\mathrm{C} \\ $$$$\Rightarrow\mathrm{p}\left(\mathrm{x}\right)=\left(\mathrm{x}−\mathrm{1}\right)\left[\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{x}−\mathrm{3}\right)\left(\mathrm{x}−\mathrm{4}\right)\left(\mathrm{x}−\mathrm{5}\right)\left(\mathrm{x}−\mathrm{6}\right)\mathrm{C}+\mathrm{1}\right]+\mathrm{1} \\ $$$$=\left(\mathrm{x}−\mathrm{1}\right)\left(\mathrm{x}−\mathrm{2}\right)\left(\mathrm{x}−\mathrm{3}\right)\left(\mathrm{x}−\mathrm{4}\right)\left(\mathrm{x}−\mathrm{5}\right)\left(\mathrm{x}−\mathrm{6}\right)\mathrm{C}+\mathrm{x} \\ $$$$\mathrm{p}\left(\mathrm{7}\right)=\mathrm{6}!\mathrm{C}+\mathrm{7}=\mathrm{720C}+\mathrm{7},\:\mathrm{C}\in\mathbb{R}^{\ast} \\ $$$$\mathrm{but}\:\mathrm{C}=\mathrm{1}\:\mathrm{because}\:\mathrm{p}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{6}} +\mathrm{ax}^{\mathrm{5}} +… \\ $$$$\Rightarrow\mathrm{p}\left(\mathrm{7}\right)=\mathrm{727} \\ $$
Commented by infinityaction last updated on 04/Sep/22
$${thanks}\:{sir} \\ $$