Question Number 178213 by Acem last updated on 14/Oct/22

Commented by Acem last updated on 14/Oct/22
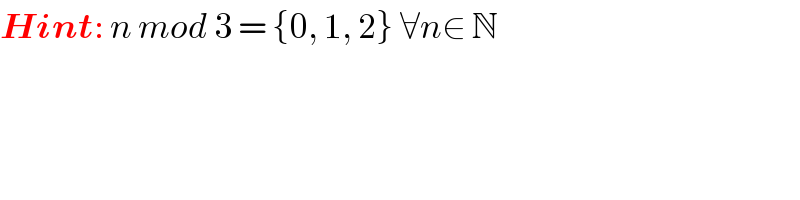
Answered by nikif99 last updated on 14/Oct/22

Commented by Acem last updated on 14/Oct/22

Commented by nikif99 last updated on 14/Oct/22

Commented by Tawa11 last updated on 15/Oct/22

