Let-S-1-2-3-4-5-6-7-If-we-multiply-atleast-2-numbers-from-this-set-with-each-other-what-are-the-chances-of-the-product-to-turn-out-to-be-divisible-by-3-

Question Number 182199 by depressiveshrek last updated on 05/Dec/22

L e t S = {1, 2, 3, 4, 5, 6, 7}

I f w e m u l t i p l y a t l e a s t 2 n u m b e r s

f r o m t h i s s e t w i t h e a c h o t h e r, w h a t

a r e t h e c h a n c e s o f t h e p r o d u c t t o

t u r n o u t t o b e d i v i s i b l e b y 3 ?

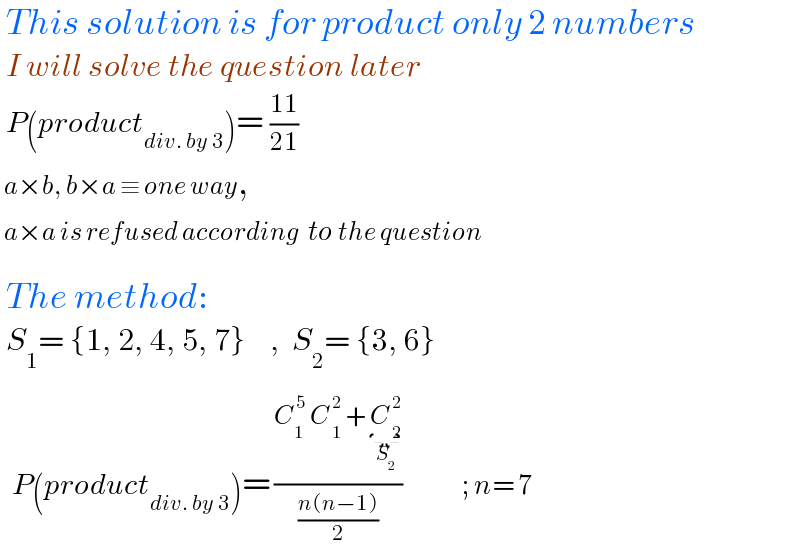
Answered by Acem last updated on 06/Dec/22

T h i s s o l u t i o n i s f o r p r o d u c t o n l y 2 n u m b e r s

I w i l l s o l v e t h e q u e s t i o n l a t e r

P ({p r o d u c t}_{d i v . b y 3}) = \frac{11}{21}

a \times b, b \times a \equiv o n e w a y,

a \times a i s r e f u s e d a c c o r d i n g t o t h e q u e s t i o n

T h e m e t h o d :

S_{1} = {1, 2, 4, 5, 7}, S_{2} = {3, 6}

P ({p r o d u c t}_{d i v . b y 3}) = \frac{C_{1}^{5} C_{1}^{2} + \underset{S_{2}}{\underset{⏟}{C_{2}^{2}}}}{\frac{n (n - 1)}{2}}; n = 7

Commented by mr W last updated on 06/Dec/22

t h e q u e s t i o n i s “ t a k e a t l e a s t t w o

{n u m b e r s}^{″}, n o t “ t a k e o n l y t w o

{n u m b e r s}^{″} a s y o u a s s u m e d .

Commented by Acem last updated on 06/Dec/22

Y e s, i {d i d n}^{'} t n o t i c e t h a t w e l l, t h a n k y o u S i r

f o r t h e c o r r e c t i o n

Answered by mr W last updated on 06/Dec/22

1) t a k e 7 n u m b e r s

t o t a l : 1 w a y

d i v i s i b l e b y 3 : 1 w a y

2) t a k e 6 n u m b e r s

t o t a l : 7 w a y s

d i v i s i b l e b y 3 : 7 w a y s

3) t a k e 5 n u m b e r s

t o t a l : C_{5}^{7} = 21 w a y s

d i v i s i b l e b y 3 : 1 \times C_{3}^{5} + 2 \times C_{4}^{5} = 20

4) t a k e 4 n u m b e r s

t o t a l : C_{4}^{7} = 35 w a y s

d i v i s i b l e b y 3 : 1 \times C_{2}^{5} + 2 \times C_{3}^{5} = 30

5) t a k e 3 n u m b e r s

t o t a l : C_{3}^{7} = 35 w a y s

d i v i s i b l e b y 3 : 1 \times C_{1}^{5} + 2 \times C_{2}^{5} = 25

6) t a k e 2 n u m b e r s

t o t a l : C_{2}^{7} = 21 w a y s

d i v i s i b l e b y 3 : 1 + 2 \times C_{1}^{5} = 11

t a k e a t l e a s t 2 n u m b e r s :

t o t a l : 1 + 7 + 21 + 35 + 35 + 21 = 120 w a y s

d i v i s i b l e b y 3 : 1 + 7 + 20 + 30 + 25 + 11 = 94 w a y s

p = \frac{94}{120} \approx 78 . 3 %

Facebook Tweet Pin