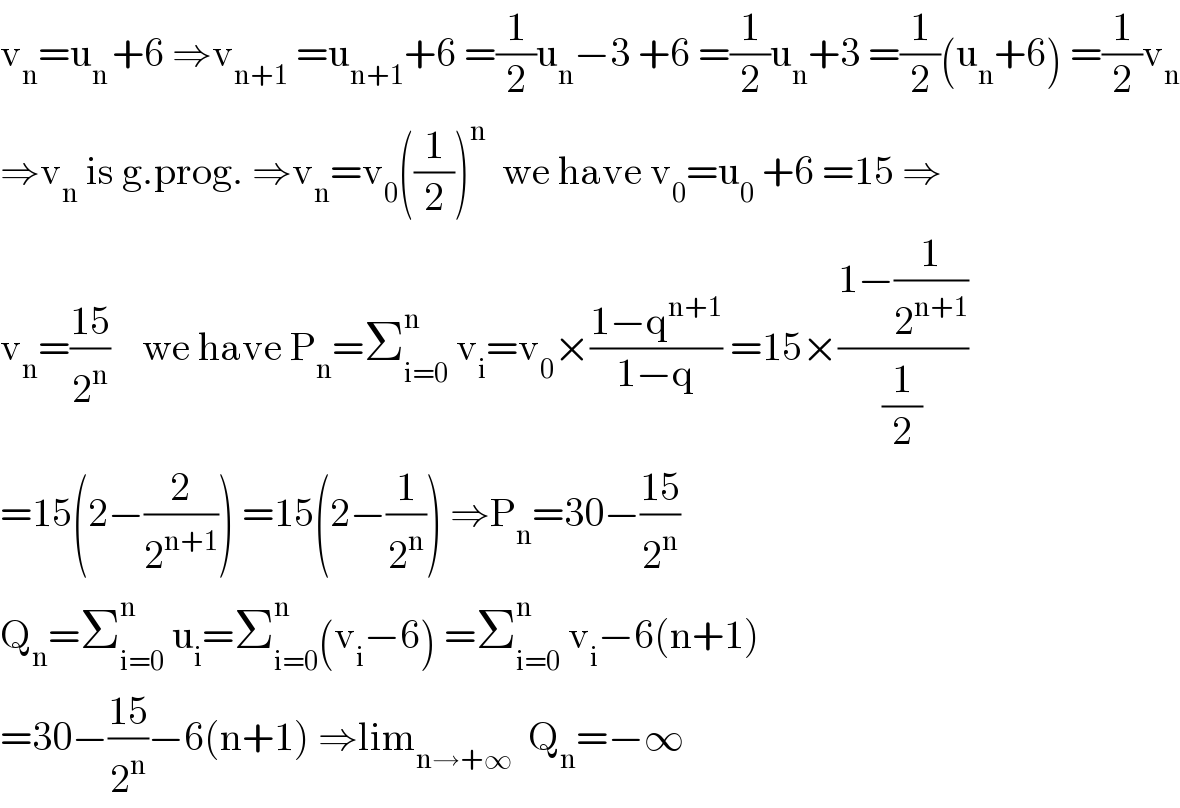Menu Close

let-u-n-and-v-n-be-sequences-defined-by-u-0-9-u-n-1-1-2-u-n-3-v-n-u-n-6-Calculate-P-n-i-0-n-V-i-in-terms-of-n-the-deduce-Q-n-i-0-n-u-i-using-the-above-expr

Tinku Tara 0 Comments
Pin




Question Number 98380 by Rio Michael last updated on 13/Jun/20
let {u_n } and {v_n } be sequences defined by u_0 = 9, u_(n+1) = (1/2)u_n −3. v_n = u_n + 6. Calculate P_n = Σ_(i=0) ^n V_i in terms of n, the deduce Q_n = Σ_(i=0) ^n u_i using the above expressions find lim_(x→∞) Q_n .
$$\mathrm{let}\:\left\{{u}_{{n}} \right\}\:\mathrm{and}\:\left\{{v}_{{n}} \right\}\:\mathrm{be}\:\mathrm{sequences}\:\mathrm{defined}\:\mathrm{by} \\ $$$$\:{u}_{\mathrm{0}} \:=\:\mathrm{9},\:{u}_{{n}+\mathrm{1}} \:=\:\frac{\mathrm{1}}{\mathrm{2}}{u}_{{n}} −\mathrm{3}. \\ $$$${v}_{{n}} \:=\:{u}_{{n}} \:+\:\mathrm{6}. \\ $$$$\mathrm{Calculate}\:{P}_{{n}} \:=\:\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}{V}_{{i}} \:\mathrm{in}\:\mathrm{terms}\:\mathrm{of}\:{n},\:\mathrm{the}\:\mathrm{deduce}\:{Q}_{{n}} \:=\:\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}{u}_{{i}} \\ $$$$\mathrm{using}\:\mathrm{the}\:\mathrm{above}\:\mathrm{expressions}\:\mathrm{find} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:{Q}_{{n}} \:. \\ $$
Answered by Aziztisffola last updated on 13/Jun/20
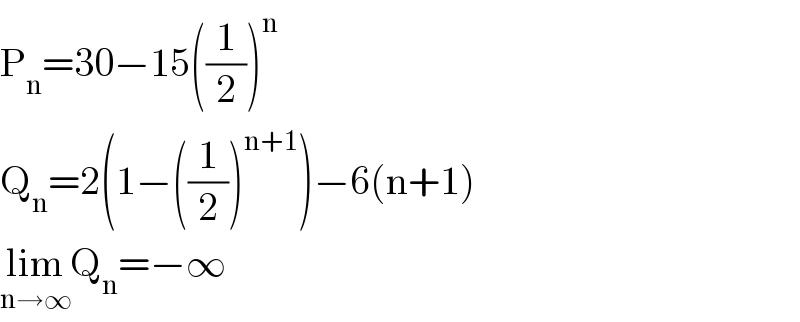
P_n =30−15((1/2))^n Q_n =2(1−((1/2))^(n+1) )−6(n+1) lim_(n→∞) Q_n =−∞
$$\mathrm{P}_{\mathrm{n}} =\mathrm{30}−\mathrm{15}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{n}} \\ $$$$\mathrm{Q}_{\mathrm{n}} =\mathrm{2}\left(\mathrm{1}−\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{n}+\mathrm{1}} \right)−\mathrm{6}\left(\mathrm{n}+\mathrm{1}\right) \\ $$$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}Q}_{\mathrm{n}} =−\infty \\ $$
Answered by mr W last updated on 13/Jun/20
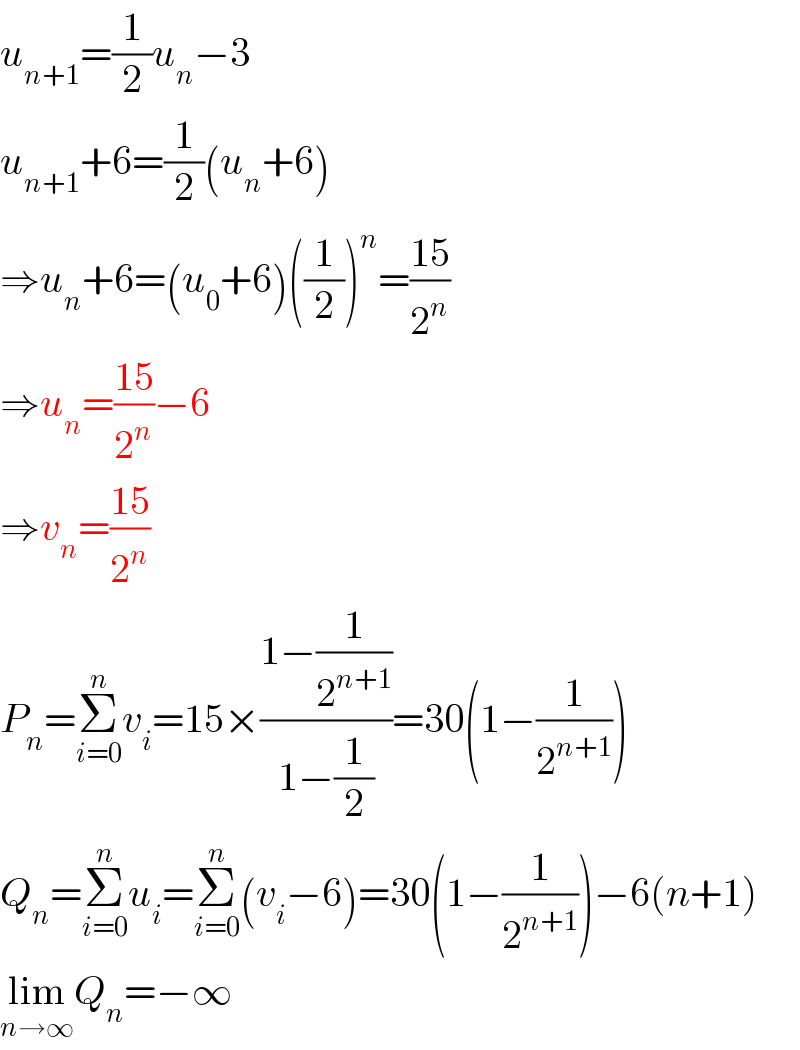
u_(n+1) =(1/2)u_n −3 u_(n+1) +6=(1/2)(u_n +6) ⇒u_n +6=(u_0 +6)((1/2))^n =((15)/2^n ) ⇒u_n =((15)/2^n )−6 ⇒v_n =((15)/2^n ) P_n =Σ_(i=0) ^n v_i =15×((1−(1/2^(n+1) ))/(1−(1/2)))=30(1−(1/2^(n+1) )) Q_n =Σ_(i=0) ^n u_i =Σ_(i=0) ^n (v_i −6)=30(1−(1/2^(n+1) ))−6(n+1) lim_(n→∞) Q_n =−∞
$${u}_{{n}+\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}}{u}_{{n}} −\mathrm{3} \\ $$$${u}_{{n}+\mathrm{1}} +\mathrm{6}=\frac{\mathrm{1}}{\mathrm{2}}\left({u}_{{n}} +\mathrm{6}\right) \\ $$$$\Rightarrow{u}_{{n}} +\mathrm{6}=\left({u}_{\mathrm{0}} +\mathrm{6}\right)\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} =\frac{\mathrm{15}}{\mathrm{2}^{{n}} } \\ $$$$\Rightarrow{u}_{{n}} =\frac{\mathrm{15}}{\mathrm{2}^{{n}} }−\mathrm{6} \\ $$$$\Rightarrow{v}_{{n}} =\frac{\mathrm{15}}{\mathrm{2}^{{n}} } \\ $$$${P}_{{n}} =\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}{v}_{{i}} =\mathrm{15}×\frac{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} }}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}}=\mathrm{30}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} }\right) \\ $$$${Q}_{{n}} =\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}{u}_{{i}} =\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}\left({v}_{{i}} −\mathrm{6}\right)=\mathrm{30}\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} }\right)−\mathrm{6}\left({n}+\mathrm{1}\right) \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}{Q}_{{n}} =−\infty \\ $$
Commented by Rio Michael last updated on 13/Jun/20
perfect now sir thanks
$$\mathrm{perfect}\:\mathrm{now}\:\mathrm{sir}\:\mathrm{thanks} \\ $$
Commented by Rio Michael last updated on 13/Jun/20
sir that is correct. thanks to you both. my worry is: why (n +1) ? i know ΣV_i = Σu_i + Σ6 ⇒ Σu_i = ΣV_i −Σ6 why will it not equal 30[1−((1/2))^n ]−6n instead it equals 30 [1−((1/2))^(n+1) ]−6n−6
$$\mathrm{sir}\:\mathrm{that}\:\mathrm{is}\:\mathrm{correct}.\:\mathrm{thanks}\:\mathrm{to}\:\mathrm{you}\:\mathrm{both}. \\ $$$$\mathrm{my}\:\mathrm{worry}\:\mathrm{is}:\:\mathrm{why}\:\left({n}\:+\mathrm{1}\right)\:? \\ $$$$\mathrm{i}\:\mathrm{know}\:\Sigma{V}_{{i}} \:=\:\Sigma{u}_{{i}} \:+\:\Sigma\mathrm{6}\:\Rightarrow\:\Sigma{u}_{{i}} \:=\:\Sigma{V}_{{i}} \:−\Sigma\mathrm{6}\: \\ $$$$\mathrm{why}\:\mathrm{will}\:\mathrm{it}\:\mathrm{not}\:\mathrm{equal}\:\mathrm{30}\left[\mathrm{1}−\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} \right]−\mathrm{6}{n}\:\:\mathrm{instead}\:\mathrm{it}\:\mathrm{equals} \\ $$$$\:\mathrm{30}\:\left[\mathrm{1}−\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}+\mathrm{1}} \right]−\mathrm{6}{n}−\mathrm{6} \\ $$
Commented by mr W last updated on 13/Jun/20
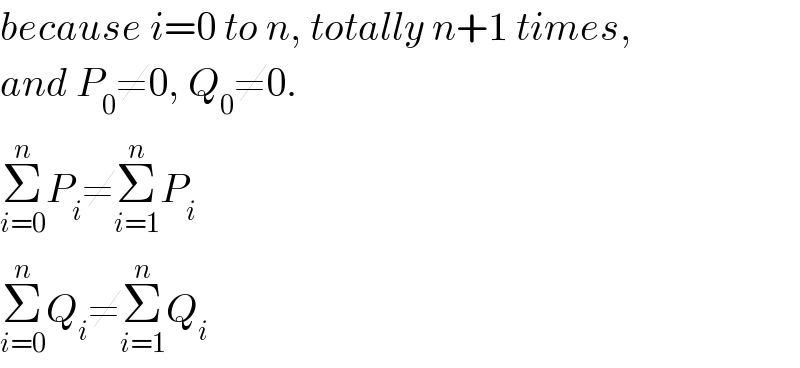
because i=0 to n, totally n+1 times, and P_0 ≠0, Q_0 ≠0. Σ_(i=0) ^n P_i ≠Σ_(i=1) ^n P_i Σ_(i=0) ^n Q_i ≠Σ_(i=1) ^n Q_i
$${because}\:{i}=\mathrm{0}\:{to}\:{n},\:{totally}\:{n}+\mathrm{1}\:{times}, \\ $$$${and}\:{P}_{\mathrm{0}} \neq\mathrm{0},\:{Q}_{\mathrm{0}} \neq\mathrm{0}. \\ $$$$\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}{P}_{{i}} \neq\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{P}_{{i}} \\ $$$$\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}{Q}_{{i}} \neq\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{Q}_{{i}} \\ $$
Answered by mathmax by abdo last updated on 13/Jun/20
v_n =u_(n ) +6 ⇒v_(n+1) =u_(n+1) +6 =(1/2)u_n −3 +6 =(1/2)u_n +3 =(1/2)(u_n +6) =(1/2)v_n ⇒v_n is g.prog. ⇒v_n =v_0 ((1/2))^n we have v_0 =u_0 +6 =15 ⇒ v_n =((15)/2^n ) we have P_n =Σ_(i=0) ^n v_i =v_0 ×((1−q^(n+1) )/(1−q)) =15×((1−(1/2^(n+1) ))/(1/2)) =15(2−(2/2^(n+1) )) =15(2−(1/2^n )) ⇒P_n =30−((15)/2^n ) Q_n =Σ_(i=0) ^n u_i =Σ_(i=0) ^n (v_i −6) =Σ_(i=0) ^n v_i −6(n+1) =30−((15)/2^n )−6(n+1) ⇒lim_(n→+∞) Q_n =−∞
$$\mathrm{v}_{\mathrm{n}} =\mathrm{u}_{\mathrm{n}\:} +\mathrm{6}\:\Rightarrow\mathrm{v}_{\mathrm{n}+\mathrm{1}} \:=\mathrm{u}_{\mathrm{n}+\mathrm{1}} +\mathrm{6}\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{u}_{\mathrm{n}} −\mathrm{3}\:+\mathrm{6}\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{u}_{\mathrm{n}} +\mathrm{3}\:=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{u}_{\mathrm{n}} +\mathrm{6}\right)\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{v}_{\mathrm{n}} \\ $$$$\Rightarrow\mathrm{v}_{\mathrm{n}} \:\mathrm{is}\:\mathrm{g}.\mathrm{prog}.\:\Rightarrow\mathrm{v}_{\mathrm{n}} =\mathrm{v}_{\mathrm{0}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{n}} \:\:\mathrm{we}\:\mathrm{have}\:\mathrm{v}_{\mathrm{0}} =\mathrm{u}_{\mathrm{0}} \:+\mathrm{6}\:=\mathrm{15}\:\Rightarrow \\ $$$$\mathrm{v}_{\mathrm{n}} =\frac{\mathrm{15}}{\mathrm{2}^{\mathrm{n}} }\:\:\:\:\mathrm{we}\:\mathrm{have}\:\mathrm{P}_{\mathrm{n}} =\sum_{\mathrm{i}=\mathrm{0}} ^{\mathrm{n}} \:\mathrm{v}_{\mathrm{i}} =\mathrm{v}_{\mathrm{0}} ×\frac{\mathrm{1}−\mathrm{q}^{\mathrm{n}+\mathrm{1}} }{\mathrm{1}−\mathrm{q}}\:=\mathrm{15}×\frac{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{n}+\mathrm{1}} }}{\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$=\mathrm{15}\left(\mathrm{2}−\frac{\mathrm{2}}{\mathrm{2}^{\mathrm{n}+\mathrm{1}} }\right)\:=\mathrm{15}\left(\mathrm{2}−\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{n}} }\right)\:\Rightarrow\mathrm{P}_{\mathrm{n}} =\mathrm{30}−\frac{\mathrm{15}}{\mathrm{2}^{\mathrm{n}} } \\ $$$$\mathrm{Q}_{\mathrm{n}} =\sum_{\mathrm{i}=\mathrm{0}} ^{\mathrm{n}} \:\mathrm{u}_{\mathrm{i}} =\sum_{\mathrm{i}=\mathrm{0}} ^{\mathrm{n}} \left(\mathrm{v}_{\mathrm{i}} −\mathrm{6}\right)\:=\sum_{\mathrm{i}=\mathrm{0}} ^{\mathrm{n}} \:\mathrm{v}_{\mathrm{i}} −\mathrm{6}\left(\mathrm{n}+\mathrm{1}\right) \\ $$$$=\mathrm{30}−\frac{\mathrm{15}}{\mathrm{2}^{\mathrm{n}} }−\mathrm{6}\left(\mathrm{n}+\mathrm{1}\right)\:\Rightarrow\mathrm{lim}_{\mathrm{n}\rightarrow+\infty} \:\:\mathrm{Q}_{\mathrm{n}} =−\infty \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *