Question Number 107320 by pticantor last updated on 10/Aug/20
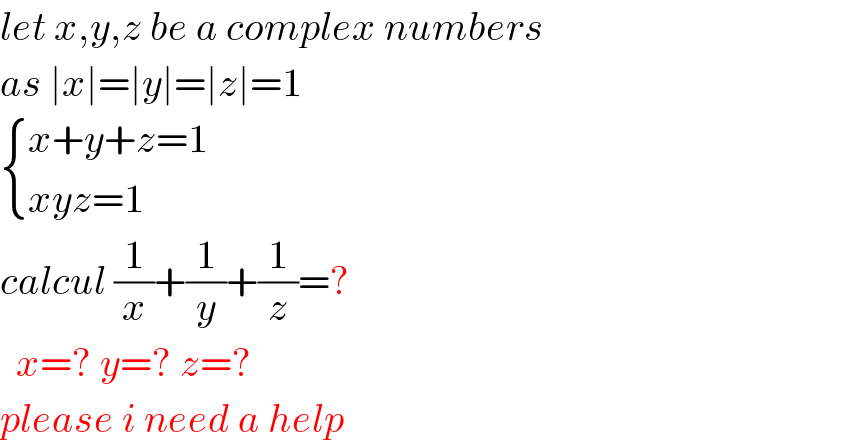
Commented by bemath last updated on 10/Aug/20
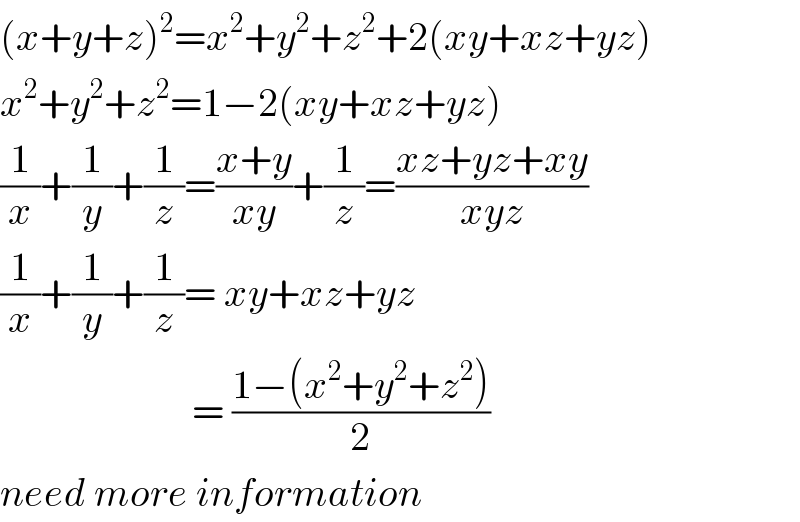
Commented by pticantor last updated on 10/Aug/20
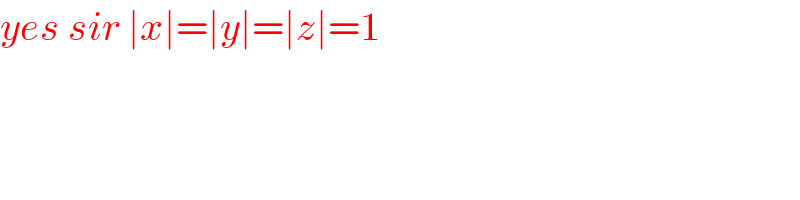
Commented by bemath last updated on 10/Aug/20
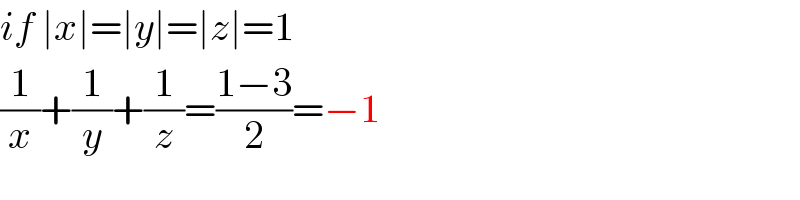
Commented by pticantor last updated on 10/Aug/20

Commented by bemath last updated on 10/Aug/20
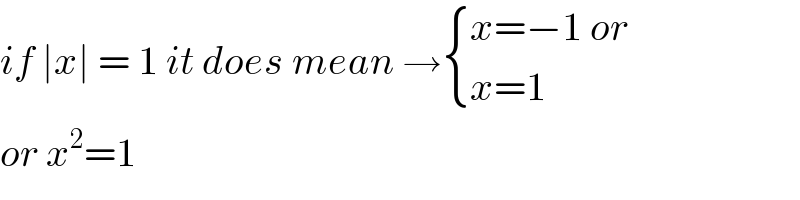
Commented by Her_Majesty last updated on 10/Aug/20
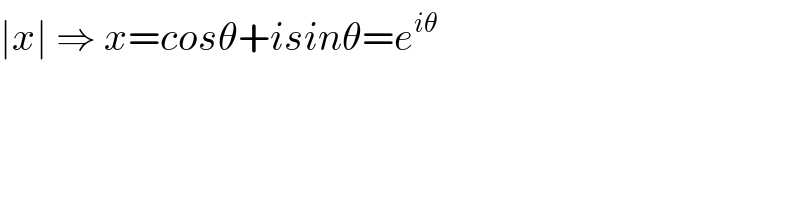
Answered by Her_Majesty last updated on 10/Aug/20
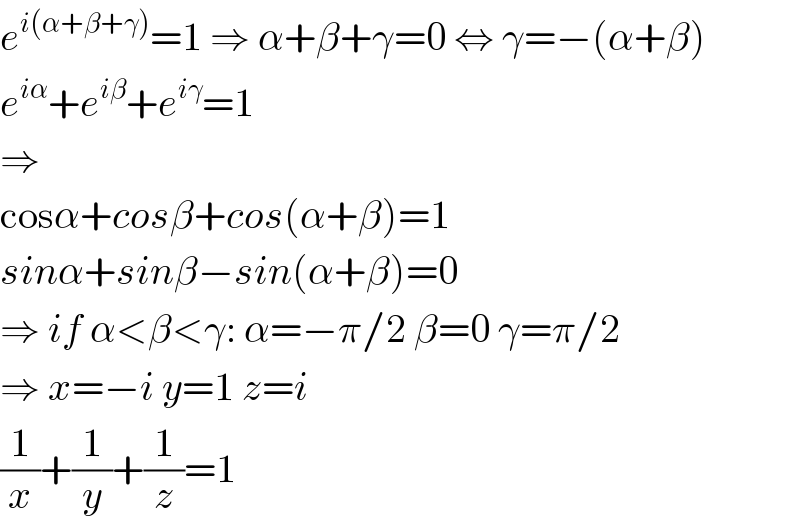
Commented by bemath last updated on 10/Aug/20
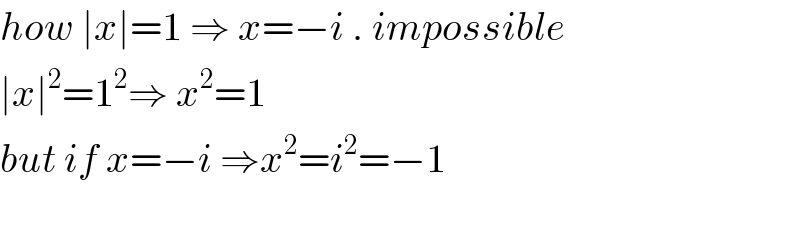
Commented by Her_Majesty last updated on 10/Aug/20
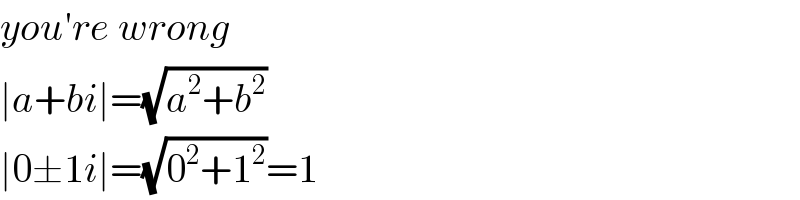
Commented by Her_Majesty last updated on 10/Aug/20

Commented by bemath last updated on 10/Aug/20
wow .... I don't know who did that action.
