Menu Close

lim-h-0-sin-h-2-sin-2-cos-h-2-sin-h-cos-2-sin-

Tinku Tara 0 Comments
Pin




Question Number 101422 by john santu last updated on 02/Jul/20
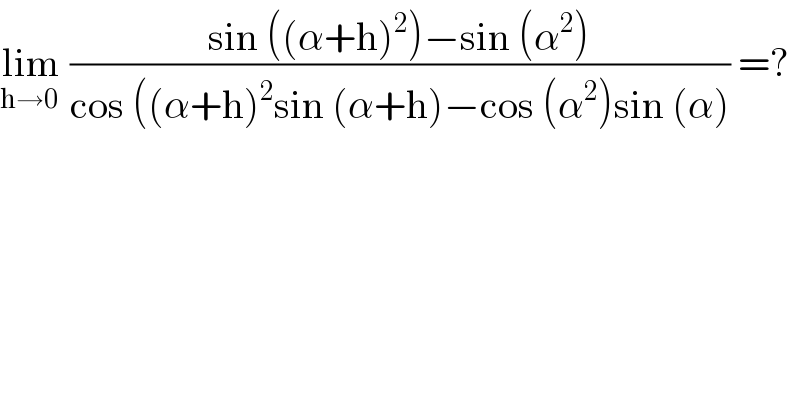
lim_(h→0 ) ((sin ((α+h)^2 )−sin (α^2 ))/(cos ((α+h)^2 sin (α+h)−cos (α^2 )sin (α))) =?
$$\underset{\mathrm{h}\rightarrow\mathrm{0}\:} {\mathrm{lim}}\:\frac{\mathrm{sin}\:\left(\left(\alpha+\mathrm{h}\right)^{\mathrm{2}} \right)−\mathrm{sin}\:\left(\alpha^{\mathrm{2}} \right)}{\mathrm{cos}\:\left(\left(\alpha+\mathrm{h}\right)^{\mathrm{2}} \mathrm{sin}\:\left(\alpha+\mathrm{h}\right)−\mathrm{cos}\:\left(\alpha^{\mathrm{2}} \right)\mathrm{sin}\:\left(\alpha\right)\right.}\:=? \\ $$
Commented by john santu last updated on 02/Jul/20
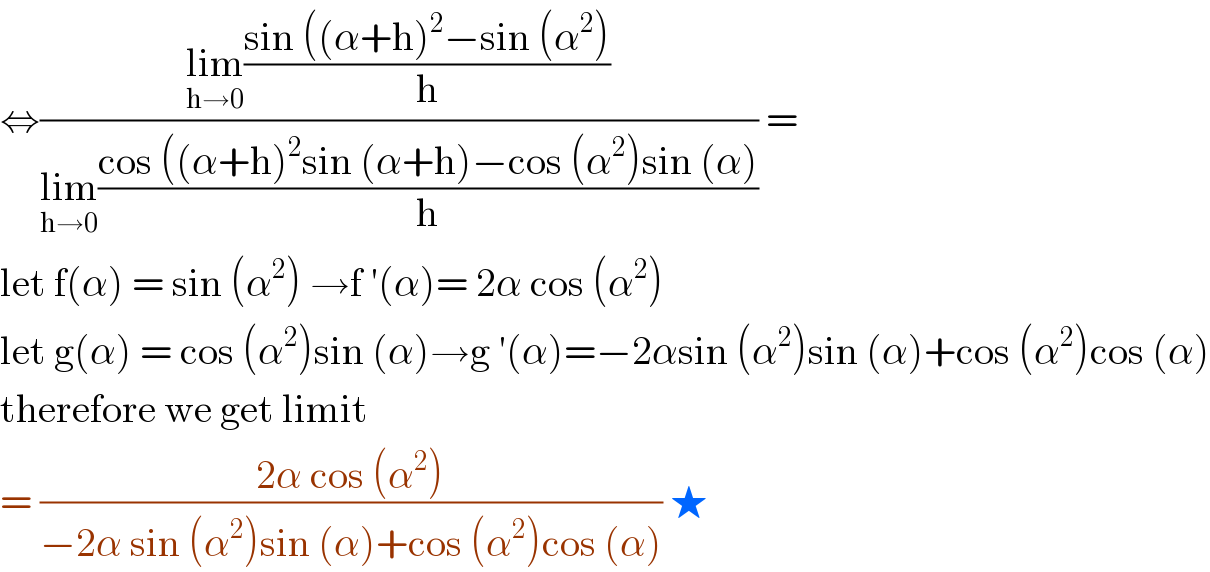
⇔((lim_(h→0) ((sin ((α+h)^2 −sin (α^2 ))/h))/(lim_(h→0) ((cos ((α+h)^2 sin (α+h)−cos (α^2 )sin (α))/h))) = let f(α) = sin (α^2 ) →f ′(α)= 2α cos (α^2 ) let g(α) = cos (α^2 )sin (α)→g ′(α)=−2αsin (α^2 )sin (α)+cos (α^2 )cos (α) therefore we get limit = ((2α cos (α^2 ))/(−2α sin (α^2 )sin (α)+cos (α^2 )cos (α))) ★
$$\Leftrightarrow\frac{\underset{\mathrm{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sin}\:\left(\left(\alpha+\mathrm{h}\right)^{\mathrm{2}} −\mathrm{sin}\:\left(\alpha^{\mathrm{2}} \right)\right.}{\mathrm{h}}}{\underset{\mathrm{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{cos}\:\left(\left(\alpha+\mathrm{h}\right)^{\mathrm{2}} \mathrm{sin}\:\left(\alpha+\mathrm{h}\right)−\mathrm{cos}\:\left(\alpha^{\mathrm{2}} \right)\mathrm{sin}\:\left(\alpha\right)\right.}{\mathrm{h}}}\:= \\ $$$$\mathrm{let}\:\mathrm{f}\left(\alpha\right)\:=\:\mathrm{sin}\:\left(\alpha^{\mathrm{2}} \right)\:\rightarrow\mathrm{f}\:'\left(\alpha\right)=\:\mathrm{2}\alpha\:\mathrm{cos}\:\left(\alpha^{\mathrm{2}} \right) \\ $$$$\mathrm{let}\:\mathrm{g}\left(\alpha\right)\:=\:\mathrm{cos}\:\left(\alpha^{\mathrm{2}} \right)\mathrm{sin}\:\left(\alpha\right)\rightarrow\mathrm{g}\:'\left(\alpha\right)=−\mathrm{2}\alpha\mathrm{sin}\:\left(\alpha^{\mathrm{2}} \right)\mathrm{sin}\:\left(\alpha\right)+\mathrm{cos}\:\left(\alpha^{\mathrm{2}} \right)\mathrm{cos}\:\left(\alpha\right) \\ $$$$\mathrm{therefore}\:\mathrm{we}\:\mathrm{get}\:\mathrm{limit} \\ $$$$=\:\frac{\mathrm{2}\alpha\:\mathrm{cos}\:\left(\alpha^{\mathrm{2}} \right)}{−\mathrm{2}\alpha\:\mathrm{sin}\:\left(\alpha^{\mathrm{2}} \right)\mathrm{sin}\:\left(\alpha\right)+\mathrm{cos}\:\left(\alpha^{\mathrm{2}} \right)\mathrm{cos}\:\left(\alpha\right)}\:\bigstar \\ $$
Commented by Dwaipayan Shikari last updated on 02/Jul/20
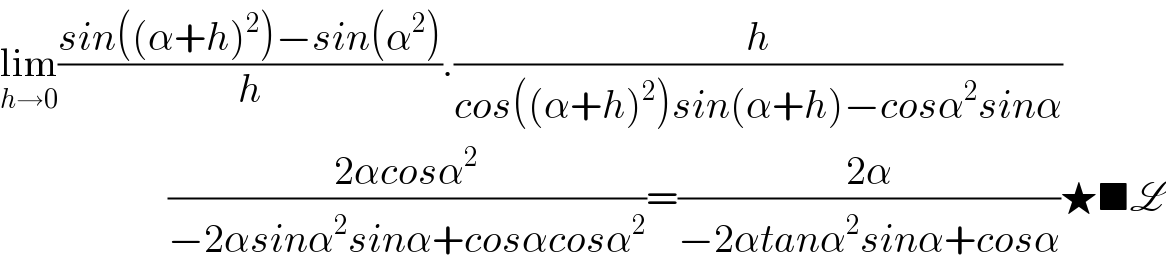
lim_(h→0) ((sin((α+h)^2 )−sin(α^2 ))/h).(h/(cos((α+h)^2 )sin(α+h)−cosα^2 sinα)) ((2αcosα^2 )/(−2αsinα^2 sinα+cosαcosα^2 ))=((2α)/(−2αtanα^2 sinα+cosα))★■L
$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{sin}\left(\left(\alpha+{h}\right)^{\mathrm{2}} \right)−{sin}\left(\alpha^{\mathrm{2}} \right)}{{h}}.\frac{{h}}{{cos}\left(\left(\alpha+{h}\right)^{\mathrm{2}} \right){sin}\left(\alpha+{h}\right)−{cos}\alpha^{\mathrm{2}} {sin}\alpha} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{2}\alpha{cos}\alpha^{\mathrm{2}} }{−\mathrm{2}\alpha{sin}\alpha^{\mathrm{2}} {sin}\alpha+{cos}\alpha{cos}\alpha^{\mathrm{2}} }=\frac{\mathrm{2}\alpha}{−\mathrm{2}\alpha{tan}\alpha^{\mathrm{2}} {sin}\alpha+{cos}\alpha}\bigstar\blacksquare\mathscr{L} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *