Question Number 149505 by mathdanisur last updated on 05/Aug/21
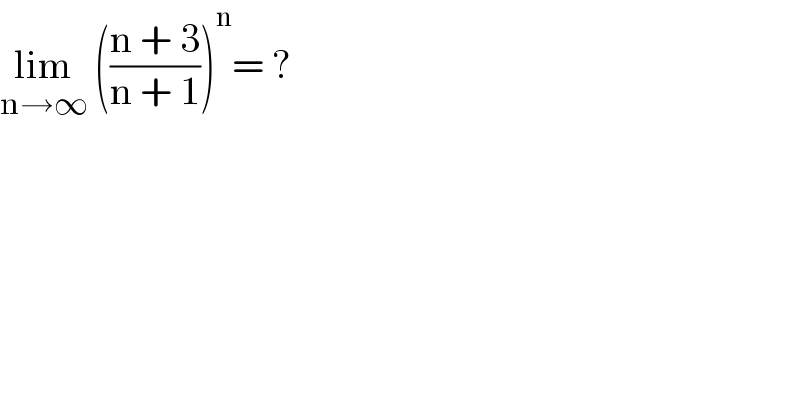
$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\:\left(\frac{\mathrm{n}\:+\:\mathrm{3}}{\mathrm{n}\:+\:\mathrm{1}}\right)^{\mathrm{n}} =\:? \\ $$
Commented by EDWIN88 last updated on 06/Aug/21
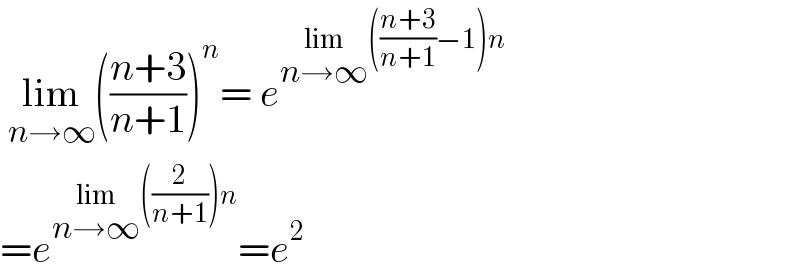
$$\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\frac{{n}+\mathrm{3}}{{n}+\mathrm{1}}\right)^{{n}} =\:{e}^{\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\frac{{n}+\mathrm{3}}{{n}+\mathrm{1}}−\mathrm{1}\right){n}} \\ $$$$={e}^{\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\frac{\mathrm{2}}{{n}+\mathrm{1}}\right){n}} ={e}^{\mathrm{2}} \\ $$
Commented by mathdanisur last updated on 06/Aug/21

$$\mathrm{Thankyou}\:\boldsymbol{\mathrm{Ser}} \\ $$
Answered by Ar Brandon last updated on 06/Aug/21
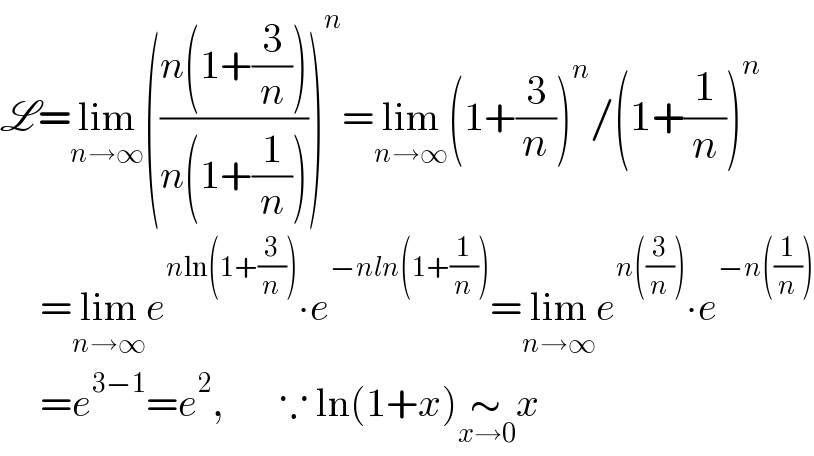
$$\mathscr{L}=\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\frac{{n}\left(\mathrm{1}+\frac{\mathrm{3}}{{n}}\right)}{{n}\left(\mathrm{1}+\frac{\mathrm{1}}{{n}}\right)}\right)^{{n}} =\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\mathrm{1}+\frac{\mathrm{3}}{{n}}\right)^{{n}} /\left(\mathrm{1}+\frac{\mathrm{1}}{{n}}\right)^{{n}} \\ $$$$\:\:\:\:\:=\underset{{n}\rightarrow\infty} {\mathrm{lim}}{e}^{{n}\mathrm{ln}\left(\mathrm{1}+\frac{\mathrm{3}}{{n}}\right)} \centerdot{e}^{−{nln}\left(\mathrm{1}+\frac{\mathrm{1}}{{n}}\right)} =\underset{{n}\rightarrow\infty} {\mathrm{lim}}{e}^{{n}\left(\frac{\mathrm{3}}{{n}}\right)} \centerdot{e}^{−{n}\left(\frac{\mathrm{1}}{{n}}\right)} \\ $$$$\:\:\:\:\:={e}^{\mathrm{3}−\mathrm{1}} ={e}^{\mathrm{2}} ,\:\:\:\:\:\:\:\because\:\mathrm{ln}\left(\mathrm{1}+{x}\right)\underset{{x}\rightarrow\mathrm{0}} {\sim}{x} \\ $$
Commented by mathdanisur last updated on 05/Aug/21

$$\boldsymbol{\mathrm{Ser}},\:\mathrm{thank}\:\mathrm{you} \\ $$
Commented by mindispower last updated on 06/Aug/21

$$\left(\mathrm{1}+\frac{{a}}{{n}}\right)^{{n}} \rightarrow{e}^{{a}} \\ $$
Commented by Ar Brandon last updated on 06/Aug/21

$$\mathrm{Oh}\:\mathrm{thanks}\:! \\ $$
Commented by mathdanisur last updated on 06/Aug/21

$$\mathrm{Thankyou}\:\boldsymbol{\mathrm{Ser}} \\ $$
Answered by ArielVyny last updated on 06/Aug/21
![lim[((n(1+(3/n)))/(n(1+(1/n))))]^n =lim(((1+(3/n))^n )/((1+(1/n))^n ))=(e^(nln(1+(3/n))) /e^(nln(1+(1/n))) )=(e^3 /e^1 )=e^2](https://www.tinkutara.com/question/Q149525.png)
$${lim}\left[\frac{{n}\left(\mathrm{1}+\frac{\mathrm{3}}{{n}}\right)}{{n}\left(\mathrm{1}+\frac{\mathrm{1}}{{n}}\right)}\right]^{{n}} ={lim}\frac{\left(\mathrm{1}+\frac{\mathrm{3}}{{n}}\right)^{{n}} }{\left(\mathrm{1}+\frac{\mathrm{1}}{{n}}\right)^{{n}} }=\frac{{e}^{{nln}\left(\mathrm{1}+\frac{\mathrm{3}}{{n}}\right)} }{{e}^{{nln}\left(\mathrm{1}+\frac{\mathrm{1}}{{n}}\right)} }=\frac{{e}^{\mathrm{3}} }{{e}^{\mathrm{1}} }={e}^{\mathrm{2}} \\ $$
Commented by mathdanisur last updated on 06/Aug/21

$$\mathrm{Thankyou}\:\boldsymbol{\mathrm{Ser}} \\ $$
Answered by Olaf_Thorendsen last updated on 06/Aug/21
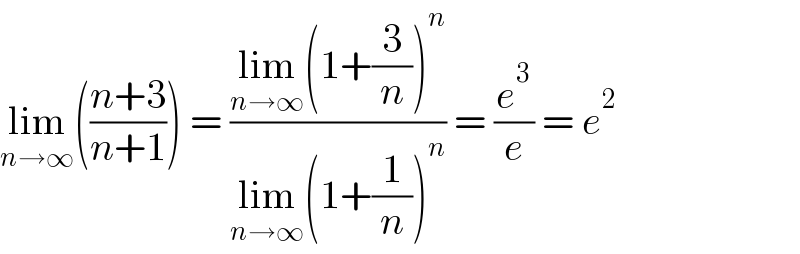
$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\frac{{n}+\mathrm{3}}{{n}+\mathrm{1}}\right)\:=\:\frac{\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\mathrm{1}+\frac{\mathrm{3}}{{n}}\right)^{{n}} }{\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\mathrm{1}+\frac{\mathrm{1}}{{n}}\right)^{{n}} }\:=\:\frac{{e}^{\mathrm{3}} }{{e}}\:=\:{e}^{\mathrm{2}} \\ $$
Commented by mathdanisur last updated on 06/Aug/21

$$\mathrm{Thankyou}\:\boldsymbol{\mathrm{Ser}} \\ $$
